When it comes to understanding the behavior of light at the interface between two different mediums, the Fresnel equations play a crucial role. These equations, named after French physicist Augustin-Jean Fresnel, provide valuable insights into the reflection and transmission of light waves at boundaries. Not only are the Fresnel equations essential for optics and the study of electromagnetic waves, but they also have practical applications in fields like photography, engineering, and telecommunications.
In this article, we will explore eight fascinating facts about Fresnel equations that will deepen your understanding of this fundamental concept in physics. Whether you are a physics enthusiast or simply curious about the nature of light, join us as we delve into the intricacies of Fresnel equations and uncover the remarkable insights they offer.
Key Takeaways:
- Fresnel Equations describe how light behaves at boundaries, helping us understand reflection and transmission. They’re crucial in designing optical systems and minimizing unwanted reflections in everyday objects like glasses.
- These equations aren’t just for optics! They also help us understand seismic and sound waves, showing how physics concepts can apply to different fields.
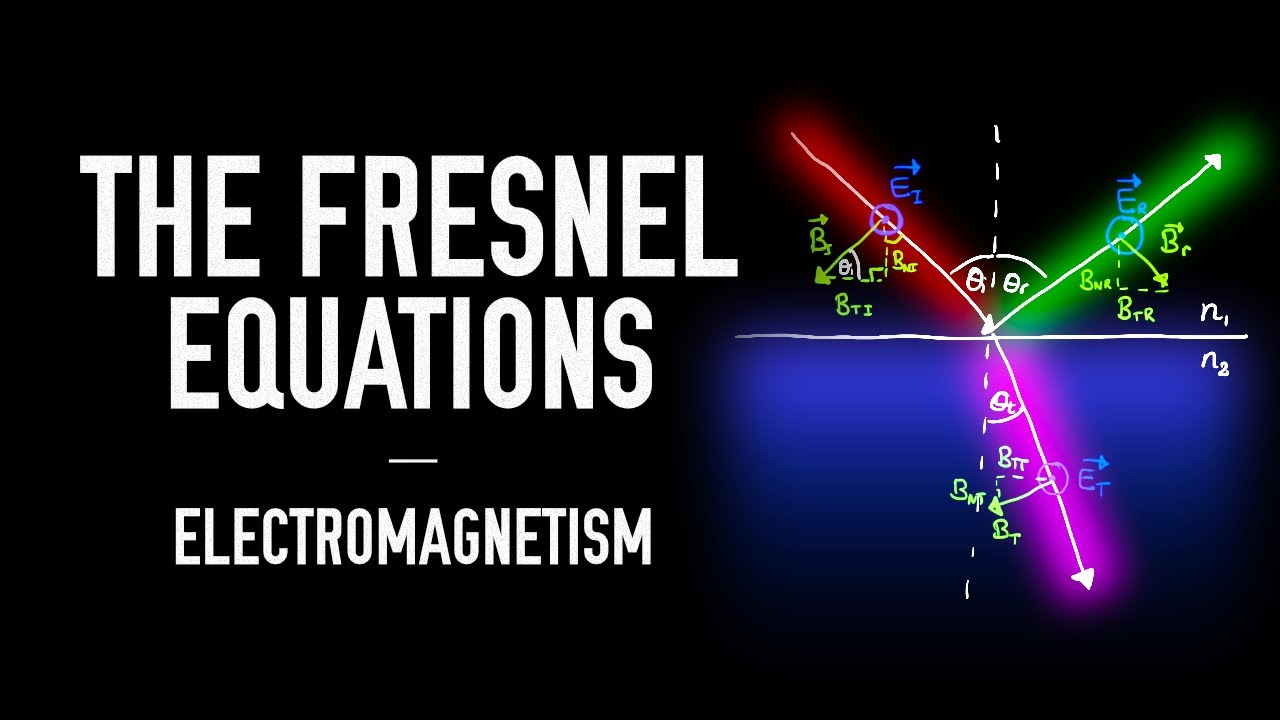
The Fresnel Equations explain how light behaves at the boundary between two mediums.
The Fresnel Equations, named after the French physicist Augustin-Jean Fresnel, are a set of equations that describe the behavior of light when it encounters the boundary between two different media such as air and glass or air and water. These equations provide valuable insights into how light is reflected and refracted at the interface.
The equations account for both reflection and transmission of light at the boundary.
The Fresnel Equations take into account the fact that when light encounters a boundary, a portion of it is reflected back into the initial medium, while the remaining portion is transmitted into the second medium. This division of light into reflected and transmitted components is crucial in understanding various optical phenomena.
The Fresnel Equations depend on the angle of incidence and the properties of the mediums involved.
The behavior of light at the boundary is determined by the angle at which the light ray strikes the interface, known as the angle of incidence. Additionally, the refractive indices of the two mediums play a crucial role in calculating the intensity and polarization of the reflected and transmitted light.
The equations are used in numerous applications, including optics and antireflection coatings.
The Fresnel Equations have widespread applications in optics, allowing scientists and engineers to analyze and design optical systems. They are particularly useful in the development of antireflection coatings, which minimize unwanted reflections and maximize the transmission of light through optical components.
The equations are fundamental in understanding the behavior of light in thin films.
When light passes through a thin film, such as a soap bubble or an oil slick, it undergoes multiple reflections and transmissions between the boundaries of the film. The Fresnel Equations play a crucial role in understanding and predicting the interference patterns observed in thin films, leading to applications in technology such as anti-reflective coatings and thin-film optics.
The equations account for both s-polarized and p-polarized light.
Light consists of both electric and magnetic fields oscillating perpendicular to the direction of propagation. The Fresnel Equations consider two different polarization states of light: s-polarized (where the electric field is perpendicular to the plane of incidence) and p-polarized (where the electric field lies in the plane of incidence). These polarization states influence the reflection and transmission properties of light at the boundary.
The equations are derived from Maxwell’s equations and boundary conditions.
The Fresnel Equations are derived from Maxwell’s equations, the fundamental equations that describe the behavior of electromagnetic waves. By applying boundary conditions at the interface between two media, such as the continuity of the electric and magnetic fields, the Fresnel Equations can be derived, providing a mathematical description of the behavior of light at the boundary.
The equations have implications in fields beyond optics, such as seismology and acoustics.
While the Fresnel Equations are commonly associated with optics, they have found applications in other fields as well. In seismology, these equations are used to understand the reflection and transmission of seismic waves, while in acoustics, they help analyze the behavior of sound waves at boundaries between different media.
Conclusion
Fresnel equations are an integral part of optics and provide a deep understanding of the behavior of light at interfaces. As we explored in this article, these equations were developed by French physicist Augustin-Jean Fresnel in the early 19th century and are still widely used today.Through the Fresnel equations, we uncovered fascinating facts about how light is reflected and transmitted at the interface between two media with different refractive indices. We learned about the concepts of reflection and refraction, as well as the importance of incident angles and polarization.These equations have numerous applications, from understanding the behavior of light in optical devices like lenses and prisms to analyzing the interactions of light with surfaces in fields such as materials science and astronomy.Overall, studying the Fresnel equations not only deepens our comprehension of optics but also opens up new possibilities for technological advancements and scientific discoveries.
FAQs
Q: What are the Fresnel equations?
A: The Fresnel equations are a set of mathematical equations developed by Augustin-Jean Fresnel to describe how light is reflected and transmitted at the interface between two media with different refractive indices.
Q: What is the importance of incident angles in the Fresnel equations?
A: Incident angles play a crucial role in the Fresnel equations as they determine the proportion of light that is reflected and transmitted at the interface. The angle of incidence affects the amount and direction of the reflected and refracted light.
Q: How do the Fresnel equations explain polarization?
A: The Fresnel equations take into account the polarization of light by considering the polarizing effects of the interface. They describe how the electric field of the incident light is affected by the interface and how it leads to changes in the polarization state of the reflected and transmitted light.
Q: What are some applications of the Fresnel equations?
A: The Fresnel equations have a wide range of applications. They are used in designing optical devices like lenses, prisms, and mirrors. They are also employed in determining the reflectivity and transmittance of materials, analyzing light-matter interactions in materials science, and studying the behavior of light in astronomy and atmospheric optics.
Q: Are the Fresnel equations applicable only to visible light?
A: No, the Fresnel equations are applicable to a broad spectrum of electromagnetic waves, including but not limited to visible light. They can be applied to the full range of electromagnetic radiation, from radio waves to X-rays and beyond.
Fresnel equations, a cornerstone of optics, have far-reaching implications. Want to explore more fascinating aspects of light? Dive into the captivating world of visible light waves, uncover mind-blowing facts about polarization, and discover the incredible applications of fiber optics. Each topic offers a unique perspective on the behavior of light, from its fundamental properties to its practical uses. Embark on a journey through these enlightening subjects and expand your understanding of the optical phenomena that shape our world.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.


