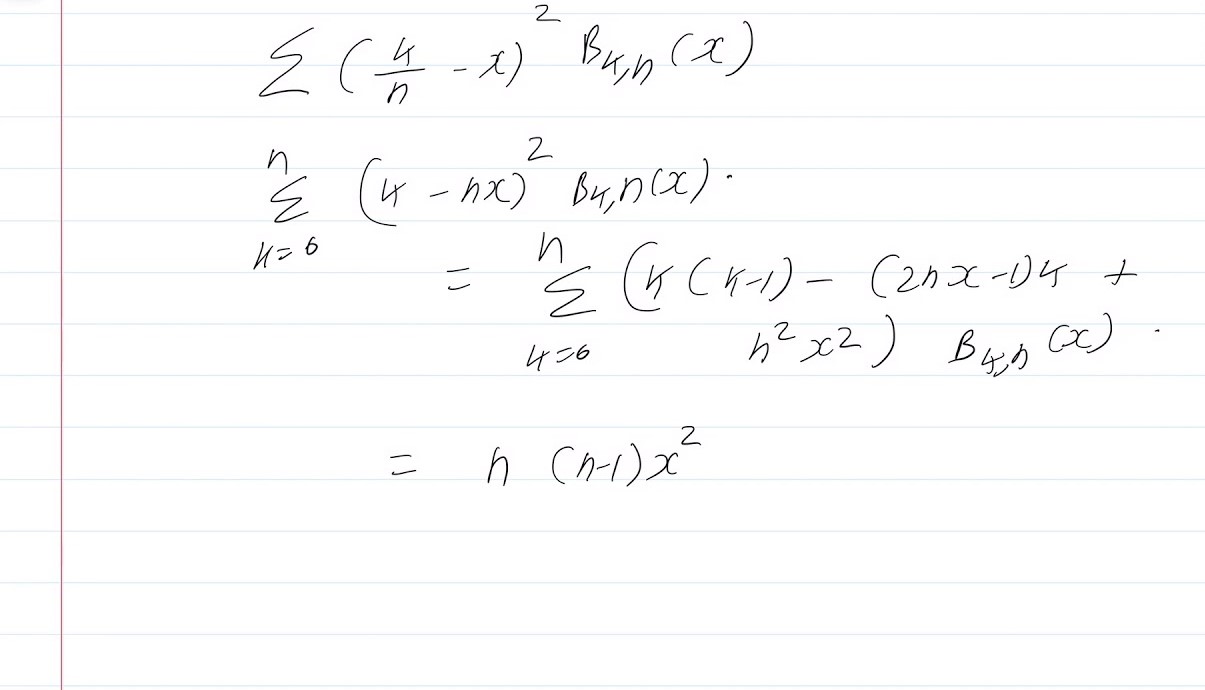
The Weierstrass Approximation Theorem is a fundamental concept in mathematical analysis, shedding light on the remarkable ability to approximate complex functions with simpler ones. This theorem, named after the eminent mathematician Karl Weierstrass, holds significant implications in various fields, including engineering, physics, and computer science. By understanding the key facts about this theorem, one can grasp its profound impact on mathematical modeling and problem-solving.
In this article, we will delve into eight essential facts about the Weierstrass Approximation Theorem, unraveling its significance and practical applications. From its historical origins to its modern-day relevance, each fact will illuminate different facets of this theorem, providing a comprehensive understanding of its importance in the realm of mathematical sciences. Let's embark on a journey to explore the intricacies of the Weierstrass Approximation Theorem and uncover the profound insights it offers to mathematicians, scientists, and enthusiasts alike.
Key Takeaways:
- The Weierstrass Approximation Theorem, formulated by Karl Weierstrass, shows how polynomials can closely mimic continuous functions, impacting fields like computer graphics and machine learning.
- This theorem’s influence extends to physics, engineering, and numerical methods, sparking ongoing research in mathematical analysis and shaping modern technological innovations.
The theorem was formulated by Karl Weierstrass.
Karl Weierstrass, a prominent German mathematician, introduced the Weierstrass Approximation Theorem in the 19th century. His groundbreaking work in mathematical analysis and the development of this theorem have had a lasting impact on the field of mathematics.
It states that continuous functions can be uniformly approximated by polynomials.
The theorem asserts that for any continuous function defined on a closed interval, there exists a sequence of polynomials that converges uniformly to the function. This concept has profound implications in numerical analysis, approximation theory, and various engineering and scientific applications.
The theorem plays a crucial role in approximation theory.
Weierstrass's theorem forms the cornerstone of approximation theory, providing a theoretical foundation for approximating complex functions with simpler ones. This has wide-ranging implications in fields such as signal processing, data analysis, and computational mathematics.
It has applications in computer graphics and image processing.
The theorem's principles are instrumental in computer graphics and image processing, where smooth curves and surfaces need to be approximated by polynomial functions. This enables the creation of realistic visual effects and the manipulation of digital images with precision.
The theorem has connections to the field of machine learning.
In the realm of machine learning, the Weierstrass Approximation Theorem underpins the development of algorithms that seek to approximate complex, high-dimensional data with simpler models. This is crucial for tasks such as pattern recognition, predictive modeling, and artificial intelligence applications.
The theorem has implications in physics and engineering.
In the domains of physics and engineering, the theorem's ability to approximate continuous functions with polynomials is invaluable. It facilitates the modeling and analysis of physical phenomena, aiding in the formulation of mathematical representations for natural processes and engineering systems.
The theorem has influenced the development of numerical methods.
Numerical methods for solving differential equations, optimization problems, and other mathematical challenges often rely on the principles established by the Weierstrass Approximation Theorem. These methods are essential in scientific computing and engineering simulations.
The theorem has sparked further research and advancements in mathematical analysis.
Weierstrass's theorem continues to inspire new avenues of research in mathematical analysis, leading to the exploration of advanced approximation techniques and the development of sophisticated mathematical models that underpin modern technological innovations.
The Weierstrass Approximation Theorem stands as a testament to the profound impact of mathematical principles on diverse fields, from computer science and engineering to physics and machine learning. Its enduring relevance underscores the pivotal role of mathematical analysis in shaping our understanding of the world around us.
Conclusion
In conclusion, the Weierstrass Approximation Theorem stands as a testament to the power and versatility of mathematical approximation. By demonstrating that continuous functions can be uniformly approximated by polynomials, this theorem has far-reaching implications in various fields, from engineering to computer science. Understanding the significance of this theorem not only enriches our knowledge of mathematical principles but also sheds light on its practical applications in real-world scenarios. As we delve deeper into the intricacies of mathematical theorems, we uncover the beauty and complexity that underpin the world of mathematics, inspiring awe and curiosity in equal measure.
FAQs
Q: What are the practical applications of the Weierstrass Approximation Theorem?
A: The Weierstrass Approximation Theorem has practical applications in various fields, including signal processing, numerical analysis, and computer-aided design. It provides a foundation for approximating complex, continuous functions with polynomials, which is essential in engineering and scientific computations.
Q: How does the Weierstrass Approximation Theorem contribute to the field of mathematics?
A: The theorem plays a pivotal role in the study of approximation theory and serves as a fundamental concept in understanding the behavior of continuous functions. It has implications in areas such as functional analysis, real analysis, and computational mathematics, enriching our understanding of mathematical principles and their applications.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
