The Banach-Steinhaus Theorem, also known as the Uniform Boundedness Principle, is a fundamental concept in mathematical analysis with far-reaching implications. This theorem provides a powerful tool for understanding the behavior of families of continuous linear operators on Banach spaces. Its significance extends to various areas of mathematics, including functional analysis, operator theory, and partial differential equations.
Understanding the Banach-Steinhaus Theorem requires delving into the fascinating world of mathematical analysis and exploring the profound implications of uniform boundedness. This theorem has practical applications in fields such as quantum mechanics, signal processing, and harmonic analysis, making it a crucial component of modern mathematical theory.
In this article, we will delve into 11 essential facts about the Banach-Steinhaus Theorem, shedding light on its significance, applications, and implications in mathematical sciences. By unraveling the intricacies of this theorem, we aim to provide a comprehensive understanding of its importance and relevance in the realm of mathematical analysis.
Key Takeaways:
- The Banach-Steinhaus Theorem ensures that a group of operators behaves consistently across a space, helping mathematicians and physicists understand and predict their behavior in various contexts.
- This theorem is like a powerful tool that helps mathematicians and physicists analyze and control the behavior of functions and operators in different mathematical and physical situations.
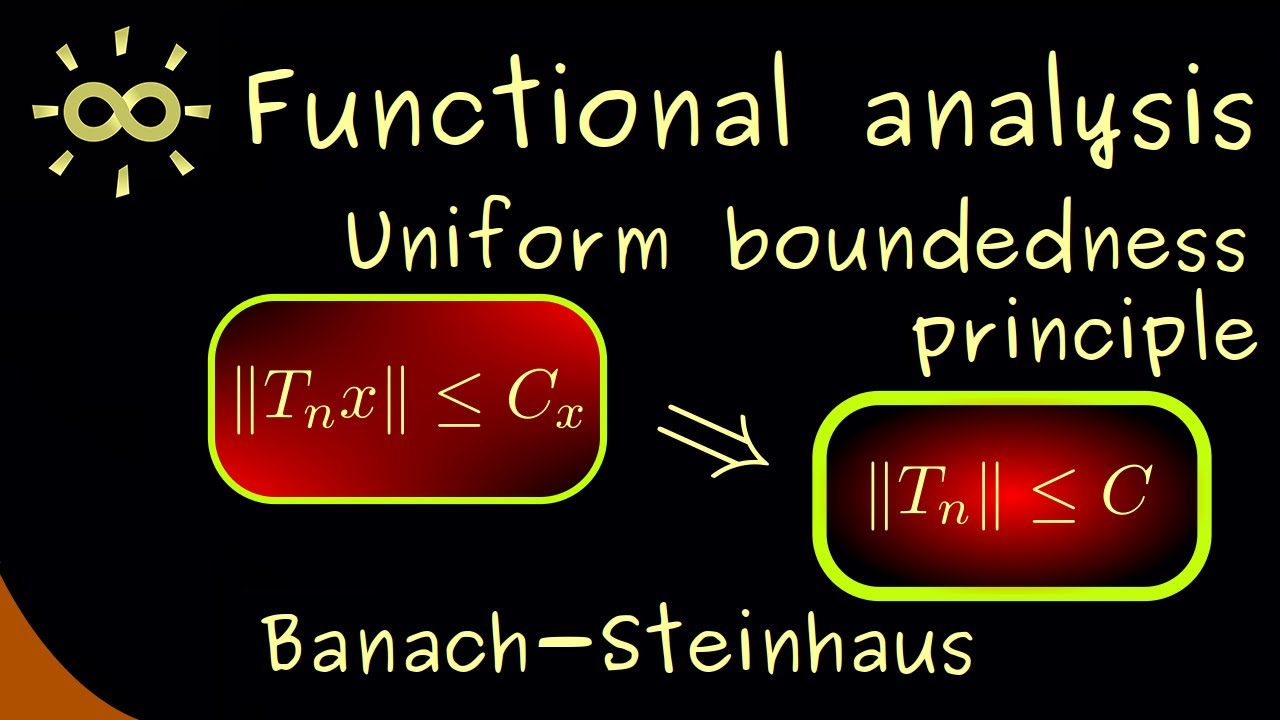
The Banach-Steinhaus Theorem (Uniform Boundedness Principle)
The Banach-Steinhaus Theorem, also known as the Uniform Boundedness Principle, is a fundamental result in functional analysis, a branch of mathematics that studies vector spaces endowed with a topology. This theorem provides a powerful tool for analyzing the convergence and boundedness of sets of functions or operators. It has wide-ranging applications in various areas of mathematics, including differential equations, harmonic analysis, and quantum mechanics.
It Ensures Uniform Boundedness
The Banach-Steinhaus Theorem guarantees that if a family of continuous linear operators from a Banach space to a normed space is pointwise bounded, then the family is uniformly bounded. In simpler terms, it ensures that the behavior of a collection of operators remains consistent and controlled across the entire space, rather than exhibiting erratic or unbounded characteristics in different regions.
This principle is invaluable in understanding the behavior of operators in functional analysis and is a cornerstone of many mathematical proofs and applications.
It Arises from the Study of Functional Analysis
The theorem originates from the field of functional analysis, which focuses on vector spaces of functions and operators. Functional analysis provides a framework for studying infinite-dimensional spaces and is essential in various areas of mathematics and physics, including quantum mechanics and signal processing.
The Banach-Steinhaus Theorem is a key result in functional analysis, shedding light on the properties of operators and their behavior in different function spaces.
It Has Applications in Quantum Mechanics
In the realm of quantum mechanics, the Banach-Steinhaus Theorem plays a crucial role in understanding the behavior of operators that represent physical observables. By ensuring the uniform boundedness of these operators, the theorem provides insights into the consistency and predictability of quantum mechanical systems, contributing to the foundational principles of quantum theory.
It Provides Insights into Boundedness of Functions
The theorem offers valuable insights into the boundedness of functions and operators, allowing mathematicians and physicists to analyze and predict the behavior of these entities in various mathematical and physical contexts. This understanding is essential for formulating accurate models and making predictions in diverse scientific disciplines.
It Influences the Study of Differential Equations
In the realm of differential equations, the Banach-Steinhaus Theorem is instrumental in analyzing the behavior of solutions and operators associated with these fundamental mathematical entities. By ensuring uniform boundedness, the theorem aids in understanding the stability and convergence of solutions to differential equations, providing essential tools for mathematical modeling and analysis.
It Shapes the Field of Harmonic Analysis
Harmonic analysis, a branch of mathematics that studies the representation of functions or signals as superpositions of basic waves, benefits significantly from the insights provided by the Banach-Steinhaus Theorem. This principle contributes to the understanding of the boundedness and convergence properties of harmonic functions and operators, enriching the study of complex wave phenomena and signal processing.
It Facilitates the Study of Operator Theory
The Banach-Steinhaus Theorem is a cornerstone of operator theory, a branch of functional analysis that investigates linear operators on function spaces. By ensuring the uniform boundedness of operators, this theorem underpins the study of operator algebras, spectral theory, and other essential concepts in operator theory, providing a solid foundation for advanced mathematical investigations.
It Offers a Fundamental Tool for Mathematical Analysis
As a fundamental result in functional analysis, the Banach-Steinhaus Theorem serves as a powerful tool for mathematical analysis, enabling mathematicians and physicists to establish the boundedness and convergence properties of operators and functions in diverse mathematical contexts. Its broad applicability and foundational significance make it an indispensable component of advanced mathematical reasoning and problem-solving.
It Demonstrates the Interplay Between Analysis and Topology
The Banach-Steinhaus Theorem highlights the intricate interplay between analysis and topology, two central branches of mathematics. By addressing the boundedness of operators in the context of topological vector spaces, the theorem exemplifies the deep connections between analytical properties and geometric structures, offering profound insights into the nature of mathematical spaces and their associated transformations.
It Reflects the Beauty of Mathematical Principles
The Banach-Steinhaus Theorem exemplifies the elegance and power of mathematical principles in elucidating fundamental properties of abstract mathematical structures. Its far-reaching implications and applications underscore the beauty and utility of mathematical reasoning, inspiring further exploration and discovery in the rich landscape of mathematical analysis and its diverse applications.
The Banach-Steinhaus Theorem (Uniform Boundedness Principle) stands as a testament to the profound connections between mathematical analysis, functional properties, and geometric structures, shaping the landscape of modern mathematics and its applications in physics, engineering, and beyond.
Conclusion
In conclusion, the Banach-Steinhaus Theorem, also known as the Uniform Boundedness Principle, is a fundamental concept in functional analysis with wide-ranging applications in mathematics and other fields. This theorem provides a powerful tool for establishing the boundedness of a set of linear operators, offering valuable insights into the behavior of such operators within a given space. By understanding the key principles and implications of this theorem, mathematicians and researchers can make significant advancements in various areas of analysis, paving the way for innovative solutions and discoveries.
FAQs
What is the significance of the Banach-Steinhaus Theorem?
The Banach-Steinhaus Theorem, or the Uniform Boundedness Principle, is significant as it provides a powerful tool for establishing the boundedness of a set of linear operators in a functional analysis setting. This theorem has wide-ranging applications in mathematics, particularly in areas such as functional analysis, operator theory, and other fields where the behavior of linear operators within a given space is of interest.
How does the Banach-Steinhaus Theorem impact mathematical research?
The Banach-Steinhaus Theorem has a profound impact on mathematical research by offering valuable insights into the behavior of linear operators within a given space. This theorem enables mathematicians and researchers to establish the boundedness of a set of operators, leading to advancements in various areas of analysis and facilitating the development of innovative solutions and discoveries.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
