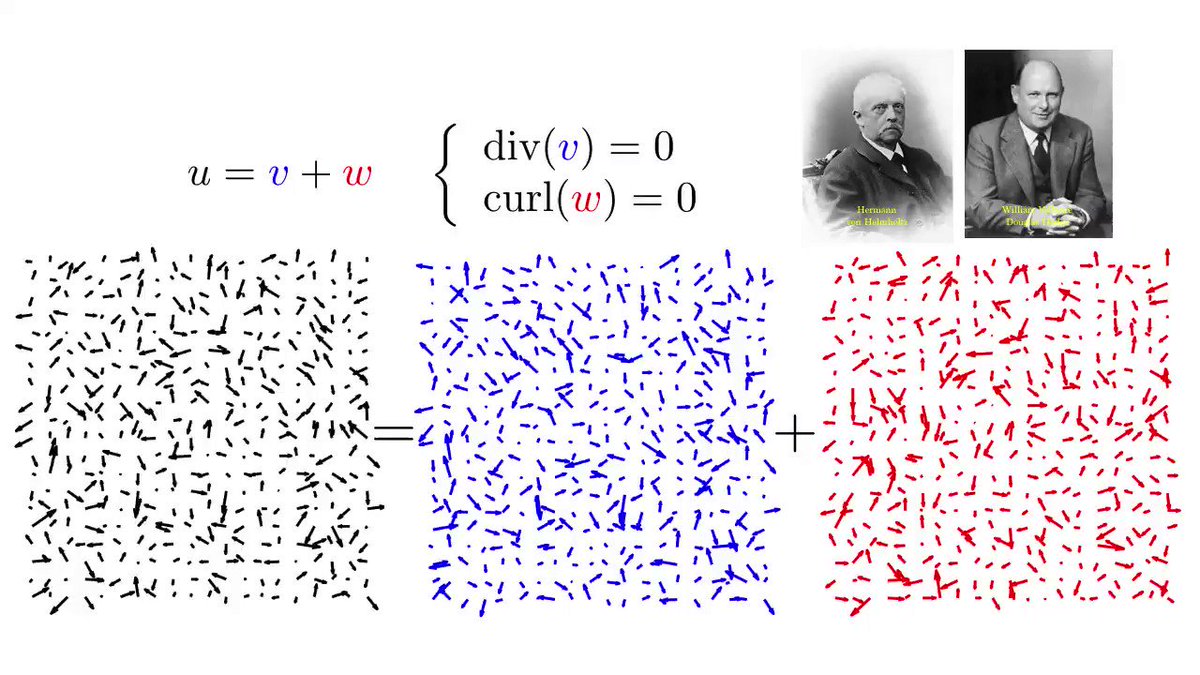
The Hodge decomposition theorem is a fundamental concept in mathematics, particularly in the field of differential geometry. It provides a powerful framework for understanding and analyzing differential forms on smooth manifolds. By decomposing these forms into orthogonal components, the theorem offers deep insights into the underlying geometric and topological structures.
The theorem, named after the mathematician W. V. D. Hodge, has far-reaching implications in various areas of mathematics and its applications extend to physics and engineering. Understanding the Hodge decomposition theorem is essential for grasping the intricate interplay between differential forms, harmonic forms, and cohomology classes.
In this article, we will delve into 11 essential facts about the Hodge decomposition theorem, shedding light on its significance, applications, and implications in diverse mathematical contexts. From its foundational principles to its practical implications, each fact will illuminate a different facet of this profound theorem, providing a comprehensive understanding of its importance in the realm of mathematical sciences.
Key Takeaways:
- The Hodge Decomposition Theorem helps mathematicians understand complex systems by breaking down differential forms into their fundamental components, empowering them to solve intricate problems with elegance and precision.
- This theorem offers a unified framework for analyzing mathematical structures, revealing the beauty and sophistication of mathematical theory while inspiring researchers to explore new frontiers in mathematics with creativity and rigor.
The Hodge Decomposition Theorem is a fundamental concept in mathematics.
The Hodge Decomposition Theorem is a fundamental concept in mathematics that provides a powerful framework for understanding and analyzing complex systems. It offers a unique perspective on the interplay between differential forms, harmonic forms, and exact forms, shedding light on the underlying structure of various mathematical spaces. This theorem serves as a cornerstone in diverse fields, including differential geometry, algebraic geometry, and mathematical physics, enabling researchers to tackle intricate problems with elegance and precision.
It reveals the intricate nature of differential forms.
The Hodge Decomposition Theorem unveils the intricate nature of differential forms, showcasing their underlying structure and behavior within mathematical spaces. By decomposing a differential form into harmonic, exact, and co-exact components, this theorem provides valuable insights into the dynamics and interactions within complex systems. Through this lens, mathematicians can discern the fundamental building blocks of differential forms and gain a deeper understanding of their properties and relationships.
It plays a pivotal role in algebraic geometry.
The Hodge Decomposition Theorem plays a pivotal role in algebraic geometry, offering a powerful tool for analyzing geometric objects and their underlying structures. By leveraging this theorem, mathematicians can unravel the intricate connections between topology, geometry, and algebra, paving the way for groundbreaking discoveries and innovative approaches to geometric problems. This theorem serves as a guiding light, illuminating the rich tapestry of algebraic geometry and empowering researchers to explore its depths with clarity and insight.
It provides a unified framework for mathematical analysis.
The Hodge Decomposition Theorem provides a unified framework for mathematical analysis, offering a cohesive perspective on diverse mathematical concepts and their interrelationships. By integrating differential forms, harmonic forms, and exact forms within a single framework, this theorem fosters a holistic understanding of mathematical structures, enabling mathematicians to navigate complex terrain with confidence and precision. Its unifying power transcends traditional boundaries, fostering interdisciplinary collaborations and inspiring new avenues of exploration in the mathematical landscape.
It offers a deep understanding of mathematical spaces.
The Hodge Decomposition Theorem offers a deep understanding of mathematical spaces, unraveling their intricate properties and revealing the underlying harmony within complex systems. By dissecting differential forms into orthogonal components, this theorem illuminates the inner workings of mathematical spaces, providing mathematicians with a profound insight into the geometric and algebraic underpinnings of diverse mathematical domains. Through this lens, researchers can discern the subtle symmetries and patterns that govern mathematical spaces, unlocking new frontiers in mathematical exploration.
It has far-reaching implications in mathematical physics.
The Hodge Decomposition Theorem has far-reaching implications in mathematical physics, offering a powerful lens through which physicists can explore the underlying structure of physical phenomena. By applying this theorem to the analysis of differential forms in the context of mathematical physics, researchers can unravel the intricate tapestry of physical laws and phenomena, shedding light on the deep connections between mathematics and the natural world. This theorem serves as a beacon of insight, guiding physicists to uncover the hidden symmetries and structures that underpin the fabric of the universe.
It facilitates the study of harmonic forms.
The Hodge Decomposition Theorem facilitates the study of harmonic forms, providing a systematic approach to understanding the intrinsic properties of these essential mathematical entities. By decomposing differential forms into harmonic components, mathematicians can delve into the rich properties of harmonic forms, uncovering their unique characteristics and significance within mathematical spaces. This theorem serves as a gateway to the profound exploration of harmonic forms, offering a roadmap for unraveling their mysteries and harnessing their potential in diverse mathematical contexts.
It fosters a deeper appreciation for differential geometry.
The Hodge Decomposition Theorem fosters a deeper appreciation for differential geometry, offering a lens through which mathematicians can explore the rich tapestry of geometric structures and their underlying properties. By leveraging this theorem, researchers can gain profound insights into the intricate interplay between differential forms and geometric spaces, paving the way for innovative approaches to geometric problems and the discovery of new geometric phenomena. This theorem serves as a guiding light, illuminating the beauty and complexity of differential geometry and inspiring mathematicians to embark on transformative journeys of exploration.
It unveils the interplay between topology and analysis.
The Hodge Decomposition Theorem unveils the intricate interplay between topology and analysis, shedding light on the deep connections between these two fundamental branches of mathematics. By providing a unified framework for understanding the geometric and analytical aspects of mathematical spaces, this theorem bridges the gap between topology and analysis, offering a cohesive perspective on the underlying structure of diverse mathematical domains. Its insights empower mathematicians to navigate the rich landscape of mathematical theory with clarity and coherence, fostering a deeper appreciation for the profound connections between topology and analysis.
It empowers researchers to tackle complex mathematical problems.
The Hodge Decomposition Theorem empowers researchers to tackle complex mathematical problems with confidence and insight, providing a versatile toolkit for unraveling the intricate mysteries of mathematical spaces. By leveraging the insights offered by this theorem, mathematicians can embark on transformative journeys of exploration, delving into the depths of differential forms, harmonic forms, and exact forms to unlock new frontiers in mathematical theory and application. This theorem serves as a beacon of inspiration, guiding researchers to push the boundaries of mathematical knowledge and embark on groundbreaking quests for discovery.
It embodies the elegance and depth of mathematical theory.
The Hodge Decomposition Theorem embodies the elegance and depth of mathematical theory, showcasing the beauty and sophistication inherent in the study of mathematical structures and their interrelationships. By offering a profound understanding of differential forms, harmonic forms, and exact forms within a unified framework, this theorem exemplifies the power and elegance of mathematical theory, inspiring mathematicians to explore the rich tapestry of mathematical concepts with creativity and rigor. Its timeless significance resonates across diverse mathematical disciplines, serving as a testament to the enduring allure and profound insights offered by the Hodge Decomposition Theorem.
In conclusion, the Hodge Decomposition Theorem stands as a beacon of insight and inspiration in the realm of mathematics, offering a profound understanding of differential forms, harmonic forms, and exact forms within a unified framework. Its far-reaching implications span across diverse mathematical disciplines, empowering researchers to unravel the intricate mysteries of mathematical spaces and embark on transformative journeys of exploration. With its unifying power and timeless significance, the Hodge Decomposition Theorem continues to illuminate the path for mathematical discovery and innovation, embodying the elegance and depth of mathematical theory.
Conclusion
In conclusion, the Hodge decomposition theorem is a fundamental result in mathematical analysis that provides a powerful framework for understanding and decomposing differential forms on smooth manifolds. By establishing the existence of harmonic forms and elucidating their relationship with exact and coexact forms, the theorem offers deep insights into the structure of differential geometry and the behavior of solutions to partial differential equations. Through its elegant decomposition of differential forms into orthogonal components, the theorem plays a pivotal role in diverse fields such as algebraic geometry, topology, and mathematical physics. Its far-reaching implications underscore the profound impact of the Hodge decomposition theorem on modern mathematics, making it an indispensable tool for researchers and practitioners alike.
FAQs
What is the significance of the Hodge decomposition theorem?The Hodge decomposition theorem holds immense significance in mathematics, particularly in the realms of differential geometry, algebraic geometry, and mathematical physics. It provides a powerful framework for decomposing differential forms on smooth manifolds, shedding light on the interplay between harmonic, exact, and coexact forms. This decomposition not only facilitates a deeper understanding of the geometric and topological properties of manifolds but also plays a crucial role in solving partial differential equations and studying the behavior of solutions in various mathematical contexts.
How does the Hodge decomposition theorem impact mathematical research and applications?The Hodge decomposition theorem has a profound impact on mathematical research and its practical applications. By enabling the decomposition of differential forms into orthogonal components, the theorem offers valuable insights into the structure of manifolds and the behavior of differential equations. This has far-reaching implications in diverse fields, including algebraic geometry, topology, and mathematical physics, where the theorem serves as a fundamental tool for analyzing and understanding complex mathematical structures and phenomena. Its applications extend to areas such as geometric analysis, string theory, and quantum mechanics, making it an indispensable concept in modern mathematics.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.


