11 Facts You Must Know About Clairaut’s Theorem

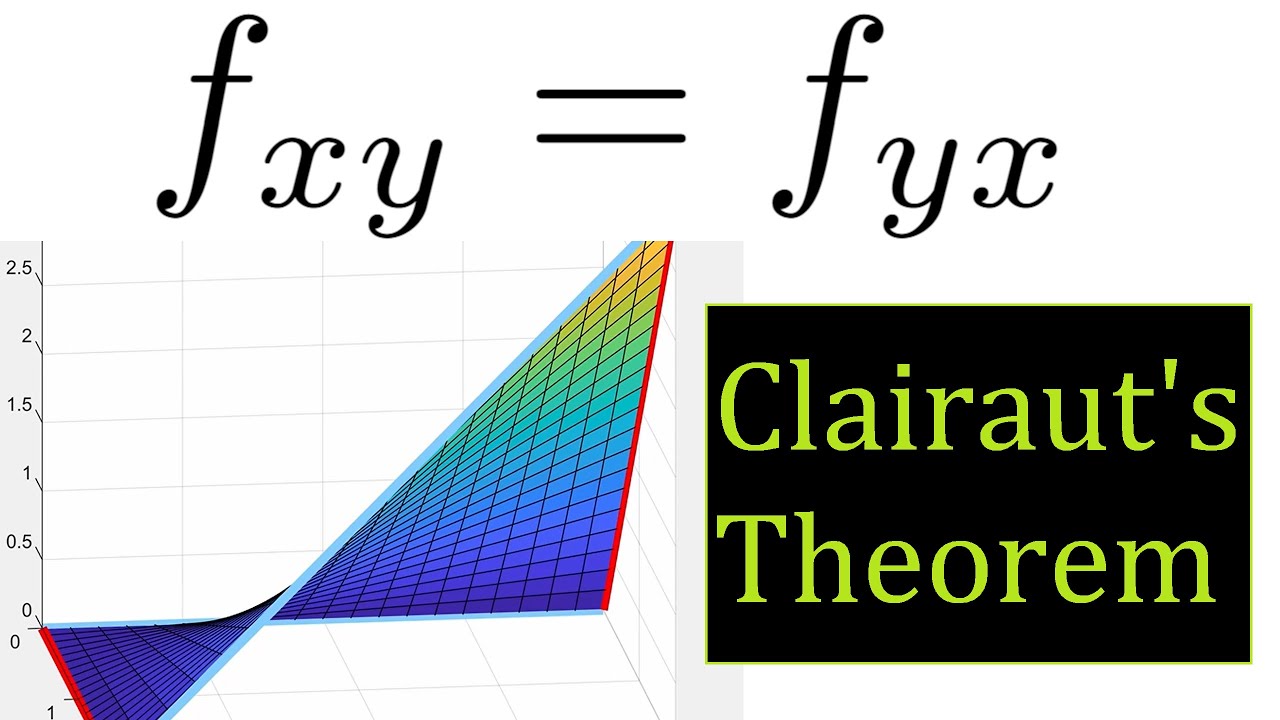
Clairaut's Theorem is Named After Alexis-Claude Clairaut

Alexis-Claude Clairaut, a French mathematician, astronomer, and geophysicist, is the eponym behind the theorem. His significant contributions to mathematics and celestial mechanics led to the naming of this theorem in his honor.

It Relates to the Second Derivative of a Function

Clairaut's Theorem is a fundamental concept in calculus that deals with the equality of mixed partial derivatives of a function. This theorem provides insights into the behavior of functions and their derivatives, offering valuable tools for mathematical analysis and problem-solving.

The Theorem is a Cornerstone of Multivariable Calculus

In the realm of multivariable calculus, Clairaut's Theorem holds immense importance. It serves as a cornerstone for understanding the relationships between partial derivatives and their applications in various fields, including physics, engineering, and economics.

It Demonstrates the Symmetry of Second Derivatives

One of the key insights offered by Clairaut's Theorem is the symmetry of second derivatives. This property sheds light on the interconnectedness of different variables within a function, unveiling patterns that aid in unraveling complex mathematical problems.

The Theorem Plays a Vital Role in Optimization Problems

In the domain of optimization, Clairaut's Theorem provides a powerful tool for analyzing critical points and determining whether they correspond to maximum, minimum, or saddle points. This capability is instrumental in various real-world scenarios, such as maximizing profits or minimizing costs in economics and engineering.

It Offers a Unified Perspective on Partial Derivatives

Clairaut's Theorem unifies the understanding of partial derivatives, showcasing the interplay between different variables within a multivariable function. This holistic view enhances the comprehension of functions with multiple inputs and outputs, paving the way for sophisticated mathematical modeling.

The Theorem Has Applications in Physics and Engineering

From analyzing the behavior of physical systems to optimizing engineering designs, Clairaut's Theorem finds widespread applications in diverse scientific and technical domains. Its insights into the behavior of multivariable functions contribute to the development of innovative solutions and advancements in these fields.

It Provides Essential Tools for Economic Analysis

In economics, the theorem's implications for analyzing production functions, utility functions, and constrained optimization problems are invaluable. By leveraging the principles of Clairaut's Theorem, economists gain deeper insights into the dynamics of economic systems and decision-making processes.

The Theorem Facilitates Insights into Geometric Properties of Surfaces

Beyond its applications in calculus and mathematical analysis, Clairaut's Theorem offers valuable perspectives on the geometric properties of surfaces. By exploring the relationships between partial derivatives, it enriches the study of surfaces and their characteristics in geometry and differential geometry.

It Empowers Problem-Solving Across Diverse Disciplines

The versatility of Clairaut's Theorem transcends traditional disciplinary boundaries, empowering problem-solving endeavors in mathematics, physics, engineering, economics, and beyond. Its broad applicability underscores its significance as a foundational concept with far-reaching implications.

The Theorem Continues to Inspire Mathematical Inquiry and Exploration

As a timeless pillar of mathematical theory, Clairaut's Theorem fuels ongoing inquiry and exploration in the realms of calculus, analysis, and applied mathematics. Its enduring relevance and profound insights ensure that it remains a source of inspiration for mathematicians and scholars worldwide.

Clairaut's Theorem, named after the esteemed mathematician Alexis-Claude Clairaut, stands as a cornerstone of multivariable calculus, offering profound insights into the behavior of functions and their derivatives. This fundamental theorem, which relates to the equality of mixed partial derivatives of a function, plays a pivotal role in diverse fields, including physics, engineering, and economics. By demonstrating the symmetry of second derivatives and providing essential tools for optimization problems, economic analysis, and geometric inquiries, Clairaut's Theorem enriches mathematical exploration and problem-solving across a spectrum of disciplines. Its enduring significance and broad applicability ensure that it continues to inspire mathematical inquiry and remains a timeless source of insight and inspiration in the world of mathematics.

Conclusion

In conclusion, Clairaut's Theorem is a powerful tool in the realm of mathematical sciences, offering profound insights into the behavior of functions and their derivatives. Its applications extend across various fields, from physics to engineering, making it a fundamental concept for understanding the intricacies of mathematical phenomena. By grasping the 11 key facts about Clairaut's Theorem, one can gain a deeper appreciation for its significance and practical implications. Embracing the elegance of this theorem equips mathematicians, scientists, and enthusiasts with a robust framework for analyzing and interpreting complex systems, paving the way for groundbreaking discoveries and innovative solutions.

FAQs

What is the significance of Clairaut's Theorem?Clairaut's Theorem holds immense significance in mathematics and its applications in diverse disciplines. It provides a fundamental understanding of the relationships between mixed partial derivatives, offering valuable insights into the behavior of multivariable functions.

How does Clairaut's Theorem impact real-world applications?Clairaut's Theorem plays a pivotal role in real-world applications, particularly in fields such as physics, engineering, and economics. By elucidating the behavior of functions and their derivatives, it enables the analysis and optimization of complex systems, contributing to advancements in various industries and scientific endeavors.