The McKay correspondence is a fascinating concept that intertwines algebraic geometry and group theory, offering profound insights into the connections between these two mathematical domains. This correspondence, named after mathematician John McKay, has garnered significant attention for its intricate relationships between finite groups and algebraic singularities. Understanding the McKay correspondence unveils a captivating interplay of abstract algebraic structures and geometric properties, shedding light on the deep-seated connections within mathematics.
In this article, we will delve into seven essential facts about the McKay correspondence, unraveling its significance and implications in the realm of mathematical sciences. From its historical origins to its contemporary applications, each fact will illuminate a distinct facet of this intriguing correspondence, providing a comprehensive overview for both enthusiasts and scholars alike. Let's embark on a journey to explore the profound intricacies of the McKay correspondence and uncover the profound implications it holds within the mathematical landscape.
Key Takeaways:
- McKay Correspondence connects geometry and group theory, revealing hidden symmetries and structures in math. It helps us understand the relationship between algebraic surfaces and finite subgroups, impacting fields like algebraic geometry and particle physics.
- Discovered in string theory, McKay Correspondence inspires new mathematical discoveries and deepens our understanding of fundamental principles. It bridges diverse mathematical disciplines, shaping the landscape of modern mathematics and fueling the quest for deeper understanding.
McKay Correspondence is a Fundamental Concept in Algebraic Geometry
McKay Correspondence is a fundamental concept in algebraic geometry that establishes a deep connection between two seemingly unrelated areas of mathematics: algebraic geometry and group theory. This correspondence provides a powerful framework for understanding the intricate interplay between these two fields, shedding light on profound mathematical relationships that have far-reaching implications.
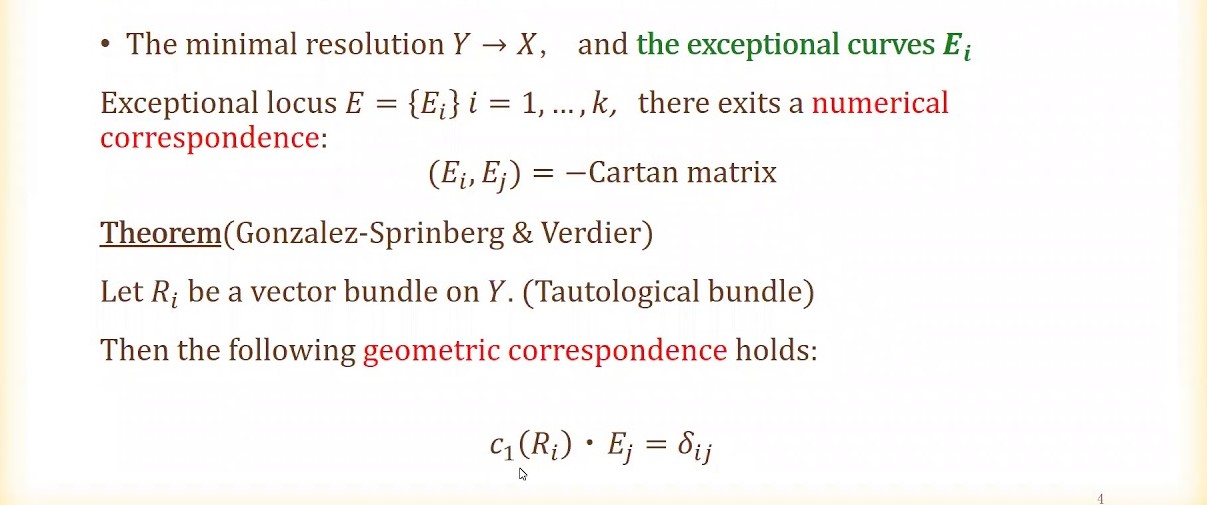
It Relates Singularities of Algebraic Surfaces to Finite Subgroups of SU(2)
The McKay Correspondence reveals a remarkable relationship between the singularities of certain algebraic surfaces and the finite subgroups of the special unitary group SU(2). This connection unveils a captivating interplay between the geometric properties of algebraic surfaces and the algebraic structures of finite subgroups, offering profound insights into the underlying symmetries and structures inherent in these mathematical entities.
It Was First Discovered in the Context of String Theory
Originally discovered in the context of string theory, the McKay Correspondence has since transcended its origins and found applications in various branches of mathematics, including algebraic geometry, representation theory, and combinatorics. This interdisciplinary nature underscores the far-reaching significance of the McKay Correspondence, as it continues to inspire new mathematical discoveries and deepen our understanding of fundamental mathematical principles.
It Provides a Bridge Between Geometry and Group Theory
By establishing a bridge between geometry and group theory, the McKay Correspondence enriches our comprehension of the intricate connections between these mathematical domains. This profound linkage offers a unified perspective that unearths hidden symmetries and structures, fostering a deeper appreciation of the underlying mathematical fabric that permeates diverse areas of mathematics.
It Unveils Intricate Symmetry Structures
The McKay Correspondence unveils intricate symmetry structures encoded within algebraic surfaces and finite subgroups, illuminating the profound interplay between geometry and group theory. This revelation provides a fertile ground for exploring the rich tapestry of mathematical symmetries and their profound implications across various mathematical disciplines.
It Has Implications in Particle Physics
The McKay Correspondence has intriguing implications in particle physics, particularly in the study of quiver representations and their connection to gauge theories. This connection underscores the far-reaching impact of the McKay Correspondence, as it intertwines with the fabric of theoretical physics, offering profound insights into the underlying symmetries and structures that underpin the fundamental forces of the universe.
It Continues to Inspire Mathematical Research and Discoveries
As a rich source of mathematical inspiration, the McKay Correspondence continues to stimulate groundbreaking research and discoveries across diverse mathematical disciplines. Its profound implications reverberate through algebraic geometry, group theory, representation theory, and beyond, shaping the landscape of modern mathematics and fueling the quest for deeper mathematical understanding.
The McKay Correspondence stands as a testament to the profound interconnectedness of mathematical concepts, transcending disciplinary boundaries to unveil the intricate symmetries and structures that permeate the mathematical universe. Its far-reaching implications continue to inspire new avenues of research and deepen our appreciation of the profound relationships that underpin the fabric of mathematics.
Conclusion
In conclusion, the McKay correspondence is a fascinating and powerful concept that has deep connections between algebraic geometry and representation theory. Understanding this correspondence provides valuable insights into the interplay between these two seemingly disparate areas of mathematics. The intricate relationships it reveals shed light on the underlying structures of both fields, offering a rich tapestry of connections waiting to be explored. As mathematicians continue to delve into the depths of this correspondence, its significance and applications are likely to unfold even further, enriching our understanding of the fundamental principles that govern these mathematical domains.
FAQs
What is the McKay correspondence?The McKay correspondence is a profound mathematical relationship that links algebraic geometry and representation theory. It establishes connections between the geometry of certain singularities and the representation theory of finite groups.
How does the McKay correspondence impact mathematics?The McKay correspondence has far-reaching implications in mathematics, providing insights into the interplay between algebraic geometry and representation theory. It offers a deeper understanding of the underlying structures in these fields and has led to significant advancements in various mathematical theories and applications.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
