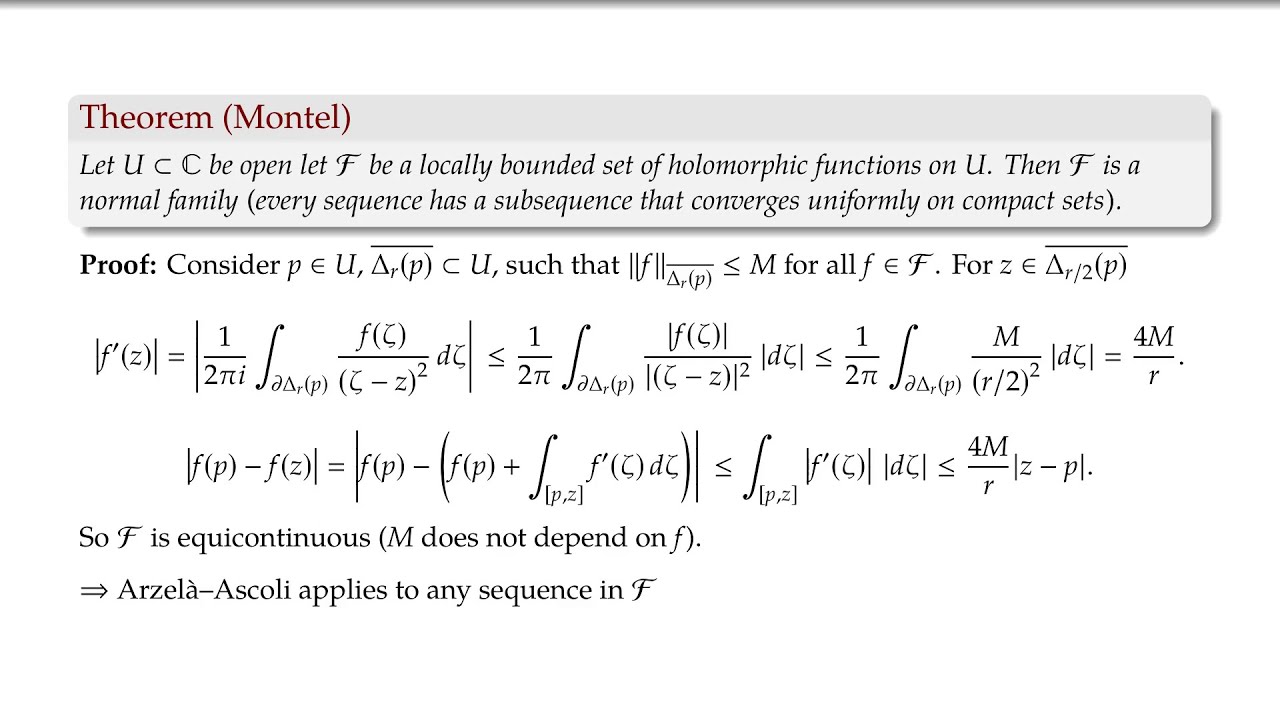
Montel's Theorem, a fundamental concept in complex analysis, holds significant importance in the field of mathematical sciences. Understanding this theorem is crucial for grasping the behavior of families of analytic functions. In this article, we will delve into 11 essential facts about Montel's Theorem, shedding light on its applications, implications, and relevance in mathematical analysis.
Montel's Theorem, named after French mathematician Pierre Montel, provides valuable insights into the behavior of families of analytic functions in complex analysis. It serves as a powerful tool for analyzing the properties of such functions within a given domain, offering a deeper understanding of their behavior and characteristics.
As we explore the intricacies of Montel's Theorem, we will uncover its practical applications in various mathematical contexts, highlighting its role in shaping the foundations of complex analysis. By unraveling the significance of this theorem, we aim to provide a comprehensive overview that illuminates its relevance and impact within the realm of mathematical sciences.
Key Takeaways:
- Montel’s Theorem is a crucial concept in math that helps us understand how special functions behave in different situations, like superheroes with unique powers in a comic book universe.
- It provides important principles for studying complex functions, like uncovering the secrets of a mysterious puzzle that can unlock the hidden potential of mathematical equations.
Montel's Theorem is a fundamental concept in complex analysis.
Montel's Theorem, a pivotal principle in complex analysis, provides essential insights into the behavior of families of holomorphic functions. This theorem plays a crucial role in understanding the properties of complex functions and their behavior within various domains.
It was first formulated by French mathematician Paul Montel.
Paul Montel, a prominent figure in the field of mathematics, introduced this theorem, which has since become a cornerstone of complex analysis. His groundbreaking work has significantly influenced the study of complex functions and their applications in diverse mathematical contexts.
Montel's Theorem addresses the behavior of families of holomorphic functions.
This theorem specifically focuses on the behavior of families of holomorphic functions within a given domain. It offers valuable principles for understanding the characteristics and dynamics of these functions, shedding light on their intricate properties and interactions.
The theorem provides crucial insights into the concept of normal families.
One of the key contributions of Montel's Theorem is its elucidation of the concept of normal families in complex analysis. By examining the behavior of holomorphic functions within a family, this theorem offers valuable perspectives on the notion of normality and its implications in mathematical analysis.
It offers significant implications for the study of complex functions.
Montel's Theorem holds substantial implications for the study of complex functions, providing foundational principles for analyzing their behavior and properties. This theorem serves as a vital tool for mathematicians and researchers delving into the intricate realm of complex analysis.
The theorem has applications in diverse mathematical fields.
Beyond its foundational significance in complex analysis, Montel's Theorem finds applications in various mathematical disciplines, contributing to the understanding of complex functions and their behavior in different contexts. Its far-reaching implications underscore its importance in mathematical research and applications.
Montel's Theorem plays a crucial role in understanding the dynamics of holomorphic functions.
By offering valuable insights into the behavior and characteristics of holomorphic functions, Montel's Theorem enriches the understanding of these functions' dynamics within complex analysis. Its contributions have a profound impact on the study of complex functions and their applications.
It provides essential principles for analyzing the behavior of holomorphic functions.
Montel's Theorem furnishes essential principles and methodologies for analyzing the behavior of holomorphic functions, offering valuable tools for mathematicians and researchers engaged in the study of complex analysis and related fields.
The theorem contributes to the foundational principles of complex analysis.
As a cornerstone of complex analysis, Montel's Theorem contributes to the foundational principles that underpin the study of complex functions and their behavior. Its significance resonates throughout the realm of mathematical analysis, shaping the understanding of complex functions and their applications.
It has implications for the study of mathematical functions in diverse domains.
Montel's Theorem extends its implications to the study of mathematical functions in diverse domains, enriching the understanding of their behavior and properties. Its far-reaching influence underscores its relevance in mathematical research and applications.
The theorem continues to inspire mathematical research and exploration.
Montel's Theorem stands as a source of inspiration for ongoing mathematical research and exploration, fueling the quest to unravel the complexities of holomorphic functions and their behavior. Its enduring significance motivates mathematicians and researchers to delve deeper into the intricate realm of complex analysis.
In conclusion, Montel's Theorem stands as a pivotal concept in complex analysis, offering valuable insights into the behavior of holomorphic functions and their applications in diverse mathematical contexts. Its foundational significance and far-reaching implications underscore its enduring relevance in mathematical research and exploration.
Conclusion
In conclusion, Montel's Theorem is a powerful and fundamental concept in complex analysis that has far-reaching implications in mathematics and its applications in various fields. Understanding the significance of Montel's Theorem provides a deeper insight into the behavior of complex functions and their properties. Its application in proving the existence of analytic functions within a given family of functions is invaluable, making it an indispensable tool in mathematical analysis. The theorem's elegant and profound nature underscores its enduring relevance in modern mathematics, serving as a cornerstone for further developments in the field of complex analysis.
FAQs
What is Montel's Theorem?Montel's Theorem is a fundamental result in complex analysis that provides conditions for the existence of a subsequence of a family of holomorphic functions that converges uniformly on compact subsets of the domain.
How is Montel's Theorem applied in mathematics?Montel's Theorem is applied to prove the existence of analytic functions within a given family of functions, providing crucial insights into the behavior and properties of complex functions.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.


