Triangle Facts

Definition of a Triangle

A triangle is a polygon with three sides and three angles.

Basic Properties

Triangles have three sides, which can vary in length and are connected by vertices. As a result, triangles also have three interior angles, and the sum of the angles in any triangle is always 180 degrees. Triangles also have vertices, which are the points where the sides of a triangle meet. These points are essential in determining the shape and orientation of the triangle.

Types of Triangles

Triangles can be classified based on their side lengths and angle measurements, giving rise to different types such as equilateral, isosceles, scalene, acute, obtuse, and right triangles.

Equilateral Triangle

An equilateral triangle has three equal sides and three equal angles, each measuring 60 degrees.

Isosceles Triangle

An isosceles triangle has two equal sides and two equal angles.

Scalene Triangle

A scalene triangle has no equal sides or angles.

Acute Triangle

An acute triangle has all interior angles measuring less than 90 degrees.

Obtuse Triangle

An obtuse triangle has one interior angle measuring more than 90 degrees.

Right Triangle

A right triangle has one interior angle measuring exactly 90 degrees.

Pythagorean Theorem

In a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. This theorem is expressed as a^2 + b^2 = c^2, where c represents the hypotenuse, and a and b represent the other two sides.

Triangle Inequality Theorem

The sum of the lengths of any two sides of a triangle is always greater than the length of the third side. This theorem helps determine the feasibility of constructing a triangle with given side lengths.

Area of a Triangle

The area of a triangle can be calculated using different formulas, such as the base times height divided by two, or Heron’s formula, which incorporates the lengths of all three sides.

Perimeter

The perimeter of a triangle is the sum of the lengths of its three sides.

Oldest Geometric Shape

Triangles are considered the oldest known geometric shape. They have been studied and used by civilizations throughout history, dating back thousands of years.

Architecture and Engineering

Triangular shapes provide structural stability and are commonly used in bridges and roofs. For instance, triangular truss structures are commonly employed in bridges, roofs, and towers, as they distribute weight evenly and offer structural integrity

Navigation and Surveying

Triangulation, a method that uses triangles to determine distances and locations, is vital in navigation, GPS systems, and surveying.

Art and Design

Triangular shapes are prevalent in art, design, and aesthetics, creating balance, symmetry, and visual interest in compositions.

Nature and Biology

Triangles are found abundantly in nature, from the hexagonal shapes of honeycombs to the triangular patterns on the wings of butterflies. These shapes optimize strength, efficiency, and functionality.

Fractals and Self-Similarity

Triangles play a crucial role in the creation of fractals, which are complex geometric patterns that exhibit self-similarity. Fractals can be found in natural phenomena like coastlines, clouds, and fern leaves.

Computer Graphics

Triangles are the basic building blocks of computer graphics and 3D modeling. They are used to represent and render complex shapes and surfaces.

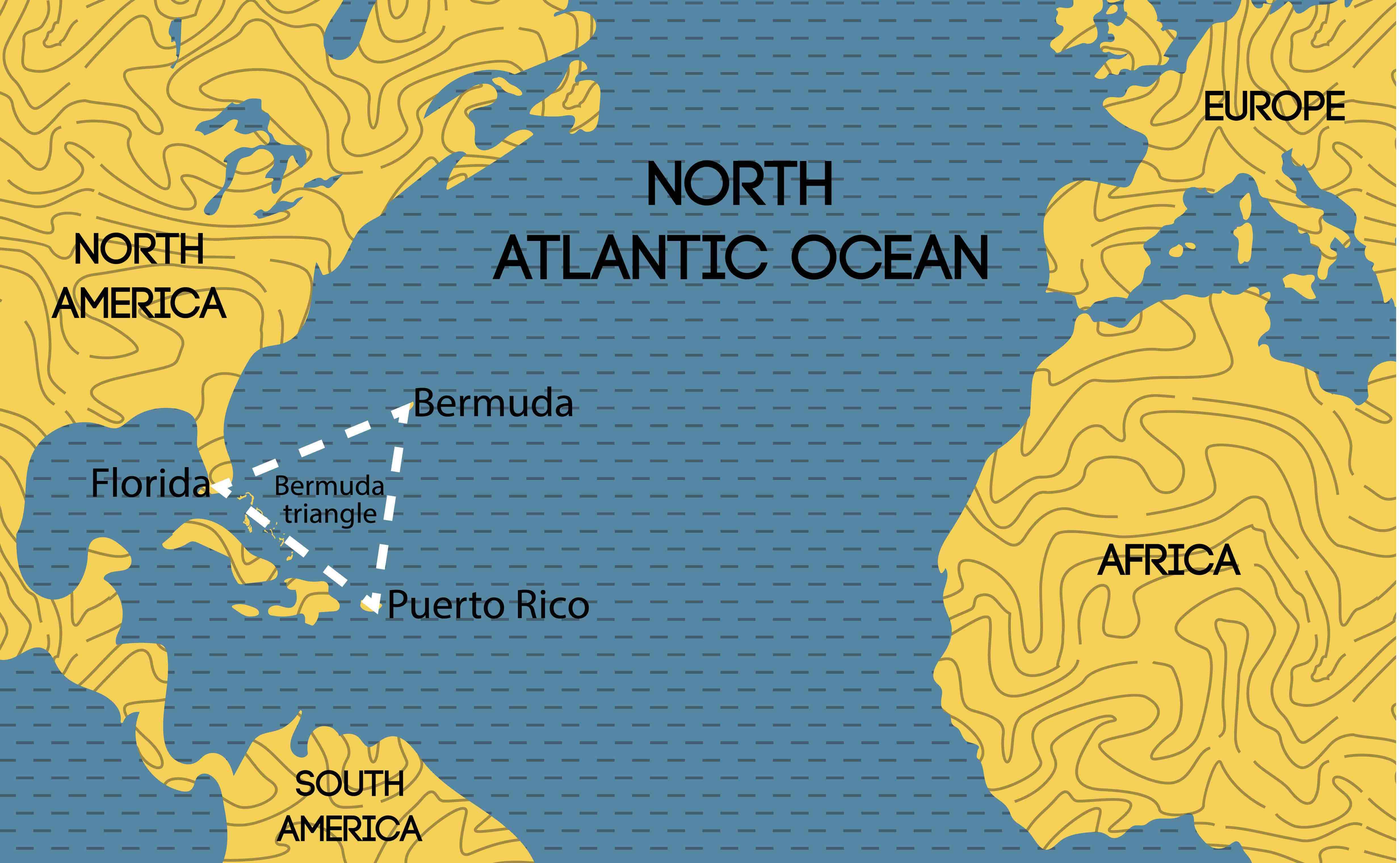
The Bermuda Triangle

The Bermuda Triangle, located in the western part of the North Atlantic Ocean, is infamous for its mysterious disappearances of ships and aircraft. Although many theories surround these disappearances, scientists attribute them to natural causes rather than any supernatural triangle-related phenomenon.

Sacred Symbolism

Triangles hold symbolic significance in various cultures and religions. For example, in Christianity, the triangle is associated with the Holy Trinity. In Hinduism, the upward-pointing triangle represents fire and male energy, while the downward-pointing triangle symbolizes water and female energy.

Conclusion

Triangles, with their geometric elegance and mathematical properties, are much more than mere shapes. They form the foundation of geometry, permeate various fields of knowledge, and exhibit stunning symmetry and balance in both natural and man-made structures. By understanding the different types, properties, and applications of triangles, we gain a deeper appreciation for their significance in the world around us. So, let us embrace the allure of triangles and embark on a journey of geometric exploration that expands our understanding of this fascinating shape.

Frequently Asked Questions (FAQs)

What is the sum of the interior angles of a triangle?

The sum of the interior angles of any triangle is always 180 degrees. This property holds true for all types of triangles.

Can a triangle have two right angles?

No, a triangle cannot have two right angles. The sum of the interior angles in a triangle is 180 degrees, and if two angles were right angles (90 degrees each), the third angle would be zero degrees, which is not possible in a triangle.

What is the difference between an acute triangle and an obtuse triangle?

An acute triangle has all interior angles measuring less than 90 degrees, while an obtuse triangle has one interior angle measuring more than 90 degrees.

Are all equilateral triangles also isosceles triangles?

Yes, all equilateral triangles are also isosceles triangles because they have at least two equal sides.

How is the perimeter of a triangle calculated?

The perimeter of a triangle is calculated by adding the lengths of all three sides. For example, if the lengths of the three sides are a, b, and c, the perimeter is given by the sum a + b + c.