Gauss’s Law is a fundamental concept in the field of physics that is named after the renowned mathematician and physicist, Carl Friedrich Gauss. It is a key principle in studying the behavior of electric fields and their relation to the distribution of electric charges. Gauss’s Law is a powerful tool that allows us to calculate the electric field produced by different charge distributions.
In this article, we will explore 11 intriguing facts about Gauss’s Law that highlight its significance in the world of physics. From its historical origins to its applications in various fields, we will delve into the fascinating aspects of this foundational principle. Whether you are a physics enthusiast or simply curious about the mysteries of the universe, join us as we uncover the captivating world of Gauss’s Law.
Key Takeaways:
- Gauss’s Law is a super important rule in electricity and magnetism. It helps us understand how electric fields come from charges and is used to solve tricky problems with symmetry.
- Gauss’s Law is like a secret code that unlocks the mysteries of electric fields. It’s not just for scientists, but also for engineers who design cool gadgets like phones and computers!
Gauss’s Law is a fundamental principle in the field of electromagnetism.
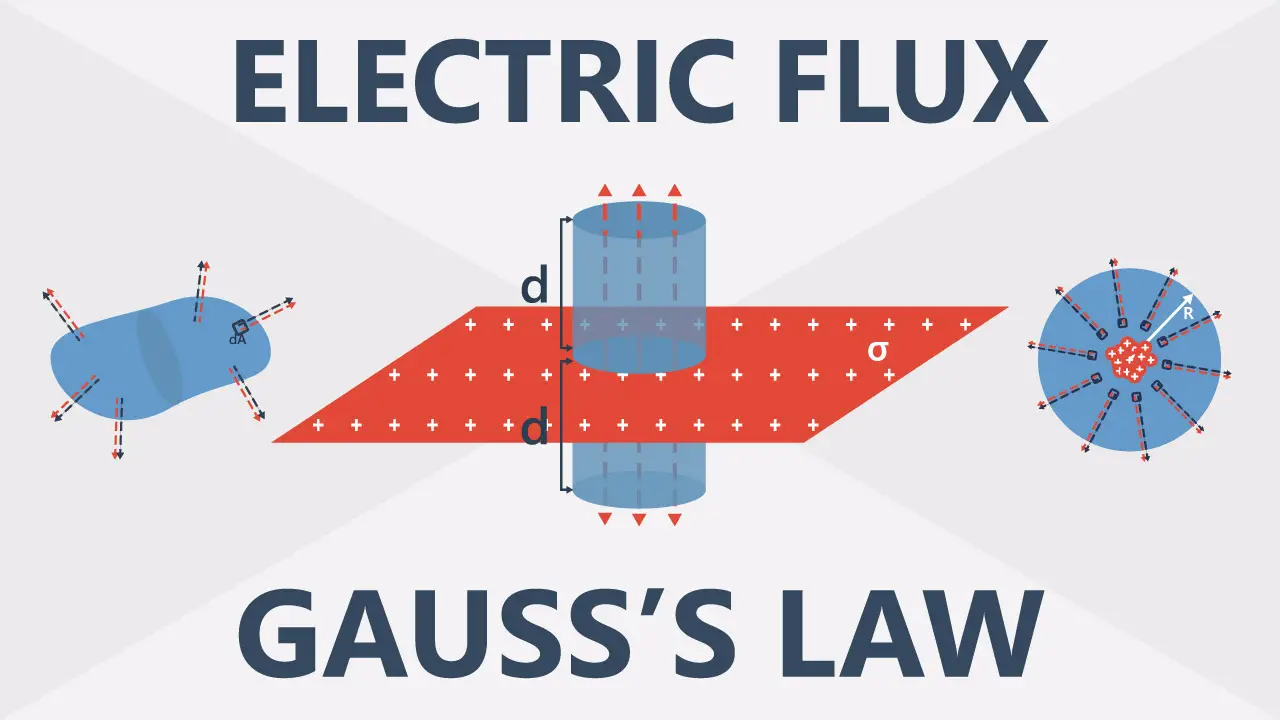
Gauss’s Law, named after the German mathematician Carl Friedrich Gauss, relates the electric flux flowing through a closed surface to the net electric charge enclosed by that surface. It provides a mathematical description of how electric fields originate from electric charges.
Gauss’s Law is one of Maxwell’s Equations.
Gauss’s Law is one of the four equations formulated by James Clerk Maxwell that describe the behavior of electric and magnetic fields. These equations form the foundation of classical electrodynamics and are fundamental to understanding the nature of light and electricity.
Gauss’s Law applies to both electric fields and magnetic fields.
While Gauss’s Law is commonly associated with electric fields, it also applies to magnetic fields. However, the magnetic flux through a closed surface is always zero, as there are no magnetic monopoles (isolated magnetic charges) in nature.
Gauss’s Law is a mathematical statement of the principle of charge conservation.
The integral form of Gauss’s Law states that the total electric flux through a closed surface is proportional to the total charge enclosed within that surface. This reflects the fundamental principle that electric charge cannot be created or destroyed, only redistributed.
Gauss’s Law is commonly used to calculate electric fields for symmetric charge distributions.
By exploiting the symmetry of a charge distribution, Gauss’s Law allows us to calculate the electric field at any point in space without having to consider the individual contributions from each charge element. This greatly simplifies the mathematical analysis of complex systems.
Gauss’s Law is valid for any closed surface.
Whether the surface is a sphere, a cube, or an arbitrary shape, Gauss’s Law applies as long as the surface is closed. This versatility makes it an invaluable tool for analyzing a wide range of physical systems.
Gauss’s Law is based on the inverse square relationship between electric fields and distance.
Gauss’s Law takes into account the inverse square law, stating that the electric field strength decreases with the square of the distance from a point charge. This relationship plays a crucial role in understanding the behavior of electric fields.
Gauss’s Law can be used to derive Coulomb’s Law.
By applying Gauss’s Law to a point charge, one can derive Coulomb’s Law, which quantitatively describes the interaction between two point charges. This demonstrates the deep connection between Gauss’s Law and the fundamental principles of electrostatics.
Gauss’s Law has applications in a variety of fields.
Gauss’s Law is not only important in the study of electromagnetism but also finds applications in diverse areas such as electrostatics, electrical engineering, and even astrophysics. It provides a powerful tool for analyzing and understanding electrical phenomena.
Gauss’s Law is a mathematical consequence of Maxwell’s Equations.
Maxwell’s Equations, which include Gauss’s Law, were formulated by James Clerk Maxwell in the 19th century. These equations are the result of years of scientific inquiry and experimentation and have played a crucial role in advancing our understanding of the physical world.
Gauss’s Law is essential for the study of electric fields and electric potential.
By applying Gauss’s Law, physicists and engineers can analyze and predict the behavior of electric fields and electric potentials in various situations. This enables the design and optimization of devices and systems that depend on the manipulation of electric fields, such as circuits and electrostatic devices.
Conclusion
In conclusion, Gauss’s Law is a fundamental concept in physics that explains the behavior of electric fields in a given space. Through this law, we can understand how electric charges distribute themselves on closed surfaces and calculate the electric flux passing through them. Gauss’s Law has immense practical applications in various fields, ranging from engineering to astrophysics.The 11 intriguing facts about Gauss’s Law covered in this article shed light on its significance and the intricacies involved. From the symmetrical nature of electric fields to the connection between Gauss’s Law and Coulomb’s Law, these facts provide a deeper understanding of this fundamental principle in physics.By exploring these facts, we can appreciate the elegance and power of Gauss’s Law and its role in shaping our understanding of the behavior of electric fields. Whether you’re a student, an academian, or simply curious about physics, delving into the realm of Gauss’s Law is sure to expand your knowledge and awe-inspiring insights into the world of electromagnetism.
FAQs
Q: What is Gauss’s Law?
A: Gauss’s Law is a fundamental concept in physics that relates the distribution of electric charges to the resulting electric fields.
Q: Who formulated Gauss’s Law?
A: Gauss’s Law was formulated by the German mathematician and physicist Carl Friedrich Gauss.
Q: How is Gauss’s Law represented mathematically?
A: Gauss’s Law is mathematically represented as the integral form ?E·dA = Q/??, where E represents the electric field, dA represents an infinitesimal area vector, Q represents the electric charges enclosed by the surface, and ?? represents the electric constant.
Q: What is the significance of Gauss’s Law in physics?
A: Gauss’s Law allows us to calculate the electric flux passing through a closed surface and provides insights into the behavior of electric fields.
Q: How is Gauss’s Law related to Coulomb’s Law?
A: Gauss’s Law and Coulomb’s Law are closely related. Gauss’s Law can be derived from Coulomb’s Law and provides a more general and elegant approach to understanding electric fields.
Q: What is meant by electric flux?
A: Electric flux refers to the total number of electric field lines passing through a given area or closed surface.
Q: Is Gauss’s Law applicable to all types of electric fields?
A: Gauss’s Law is applicable to electric fields with certain symmetries, such as those produced by point charges, charged spheres, or charged cylinders.
Q: How does Gauss’s Law relate to the concept of symmetry?
A: Gauss’s Law relies on the concept of symmetry in electric fields. It states that the electric field passing through a closed surface is proportional to the total amount of charge enclosed within the surface.
Q: Can Gauss’s Law be used to determine the electric field produced by a non-uniform charge distribution?
A: Yes, Gauss’s Law can be applied to determine the electric field of a non-uniform charge distribution by creating Gaussian surfaces that enclose small regions with uniform charge distributions.
Q: Can Gauss’s Law be used for magnetism?
A: No, Gauss’s Law is specific to electrostatics and does not apply to the behavior of magnetic fields.
Q: How can Gauss’s Law be used in practical applications?
A: Gauss’s Law finds applications in various fields, including electrical engineering, electronics, electromagnetic theory, and astrophysics, helping us understand and calculate electric fields in different scenarios.
Gauss's Law, a cornerstone of electromagnetism, continues to captivate physicists and enthusiasts alike. Its applications extend beyond electric fields, encompassing the realms of magnetism and gravitation. For those curious about the magnetic aspects of Gauss's Law, our article on surprising facts delves into its intricacies. Gravitational fields also fall under the purview of Gauss's Law, and our piece on intriguing facts explores this connection. Lastly, enigmatic facts about Gauss's Law of Gravitation await readers seeking to unravel its mysteries. Embark on a journey through these related topics and expand your understanding of this fundamental principle.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
