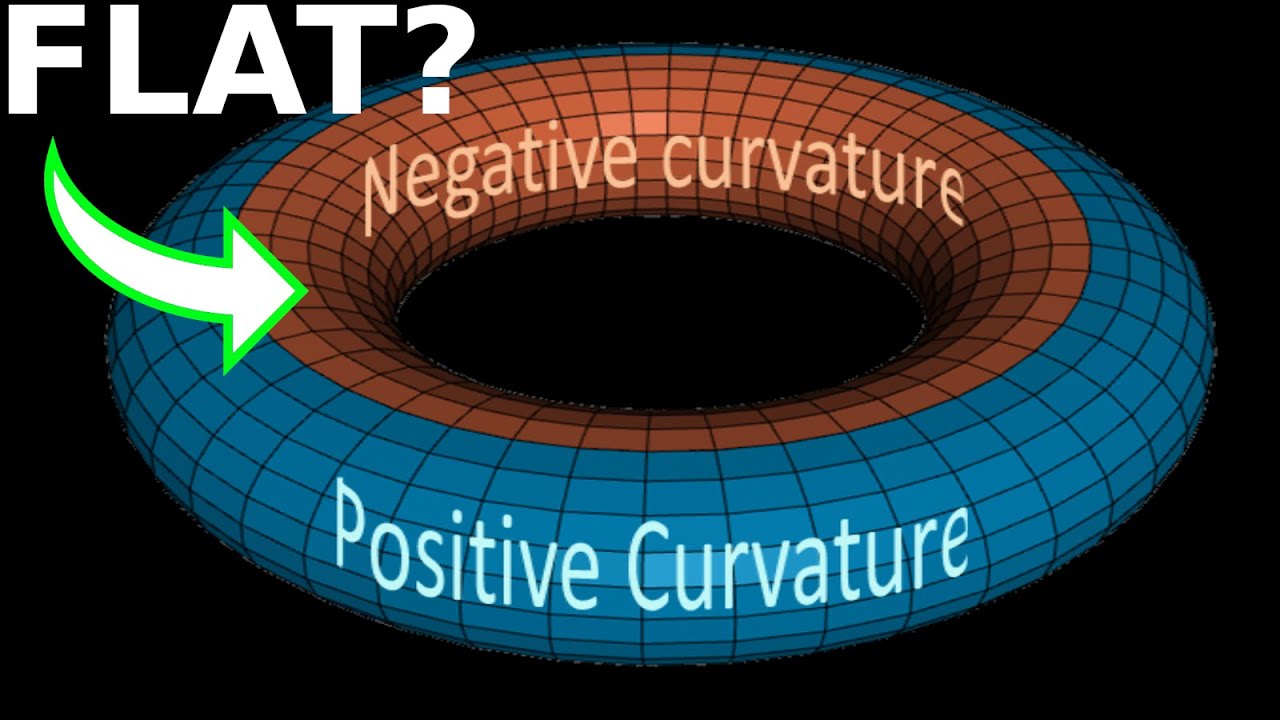
The Gauss-Bonnet Theorem is a fundamental concept in mathematics that has far-reaching implications in various fields, including differential geometry, topology, and physics. Understanding this theorem is crucial for grasping the intricate connections between curvature, topology, and the global structure of surfaces.
The theorem, named after Carl Friedrich Gauss and Pierre Ossian Bonnet, provides a profound insight into the relationship between the curvature of a surface and its topology. It establishes a remarkable link between local and global geometric properties, shedding light on the intrinsic nature of surfaces.
In this article, we will delve into nine essential facts about the Gauss-Bonnet Theorem, unraveling its significance and applications. From its historical origins to its profound impact on modern mathematics and theoretical physics, each fact will illuminate a different facet of this remarkable theorem. Join us on this journey as we explore the beauty and depth of the Gauss-Bonnet Theorem, uncovering its profound implications and captivating intricacies.
Key Takeaways:
- The Gauss-Bonnet Theorem connects the shape of curved surfaces to their hidden patterns, helping mathematicians understand the secrets of twisted spaces and how they’re put together.
- Named after two famous mathematicians, this theorem is like a powerful flashlight that helps us explore the mysterious world of curved spaces and understand their intricate geometry.
Gauss-Bonnet Theorem is a fundamental concept in differential geometry.
The Gauss-Bonnet Theorem is a fundamental concept in differential geometry, a branch of mathematics that explores the properties of curves and surfaces. It establishes a deep connection between the geometry of a surface and its topology, providing crucial insights into the intrinsic properties of curved spaces.
It relates the curvature of a surface to its topology.
The theorem establishes a profound relationship between the curvature of a surface and its topological characteristics. This connection allows mathematicians to discern intricate patterns and structures within curved spaces, shedding light on the underlying nature of these geometric entities.
The theorem is named after Carl Friedrich Gauss and Pierre Ossian Bonnet.
The Gauss-Bonnet Theorem is named in honor of two eminent mathematicians, Carl Friedrich Gauss and Pierre Ossian Bonnet, who made significant contributions to the fields of geometry and differential equations. Their collaborative work led to the development of this theorem, which has since become a cornerstone of modern mathematics.
It provides a powerful tool for studying the geometry of surfaces.
This theorem serves as a powerful tool for analyzing and understanding the intricate geometry of surfaces, enabling mathematicians to uncover hidden properties and relationships within curved spaces. By leveraging the insights derived from the Gauss-Bonnet Theorem, researchers can delve into the rich tapestry of geometric structures and unveil the underlying principles governing their behavior.
The theorem has applications in various fields, including physics and engineering.
The Gauss-Bonnet Theorem finds applications in diverse domains, ranging from theoretical physics to engineering. Its profound implications extend to the realms of general relativity, where it plays a pivotal role in elucidating the geometric properties of spacetime, as well as in the design and analysis of complex architectural structures.
It establishes a crucial link between curvature and Euler characteristic.
One of the key contributions of the Gauss-Bonnet Theorem is its establishment of a crucial link between the curvature of a surface and its Euler characteristic, a fundamental invariant in topology. This connection unveils profound insights into the interplay between geometric curvature and topological properties, enriching our understanding of curved spaces.
The theorem has far-reaching implications in the study of Riemannian manifolds.
In the realm of Riemannian geometry, the Gauss-Bonnet Theorem holds immense significance, providing a foundational framework for exploring the geometric properties of smooth manifolds. Its influence permeates through the intricate fabric of differential geometry, offering valuable tools for investigating the intrinsic curvature of multidimensional spaces.
It plays a pivotal role in the formulation of the Atiyah-Singer Index Theorem.
The Gauss-Bonnet Theorem plays a pivotal role in the formulation of the Atiyah-Singer Index Theorem, a profound result in mathematics with profound implications in diverse areas, including theoretical physics and quantum field theory. This connection underscores the far-reaching impact of the Gauss-Bonnet Theorem on advanced mathematical theories and their applications.
The theorem encapsulates the profound interplay between geometry and topology.
At its core, the Gauss-Bonnet Theorem encapsulates the profound interplay between geometry and topology, offering a unifying framework that intertwines the intrinsic curvature of surfaces with their underlying topological characteristics. This deep connection underscores the elegance and depth of mathematical structures, enriching the tapestry of differential geometry and topology.
The Gauss-Bonnet Theorem stands as a testament to the intricate interplay between geometry and topology, illuminating the profound connections that underpin the fabric of curved spaces. Through its profound implications and far-reaching applications, this fundamental theorem continues to inspire mathematicians, physicists, and researchers across diverse disciplines, shaping our understanding of the intricate geometric and topological landscapes that permeate the mathematical universe.
Conclusion
In conclusion, the Gauss-Bonnet Theorem stands as a remarkable achievement in the field of differential geometry, providing profound insights into the intrinsic curvature of surfaces. Its elegant formulation and far-reaching implications have solidified its status as a cornerstone of modern mathematics. By establishing a profound connection between topology and geometry, this theorem has not only enriched our understanding of the fundamental properties of surfaces but also found applications in diverse fields such as physics, engineering, and computer graphics. As we continue to unravel the mysteries of the mathematical universe, the Gauss-Bonnet Theorem remains a testament to the beauty and power of mathematical reasoning.
FAQs
What is the significance of the Gauss-Bonnet Theorem?The Gauss-Bonnet Theorem holds immense significance in mathematics and its applications in various fields. It provides a deep understanding of the intrinsic curvature of surfaces and establishes a profound connection between topology and geometry. This theorem has far-reaching implications in diverse areas, including physics, engineering, and computer graphics.
How is the Gauss-Bonnet Theorem applied in real-world scenarios?The Gauss-Bonnet Theorem finds applications in real-world scenarios such as geodesy, cartography, and computer graphics. In geodesy and cartography, it aids in understanding the curvature of the Earth's surface, while in computer graphics, it is utilized for rendering realistic and immersive visual environments. The theorem's applications extend to fields where the understanding of surface curvature and topology is crucial for practical and theoretical advancements.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
