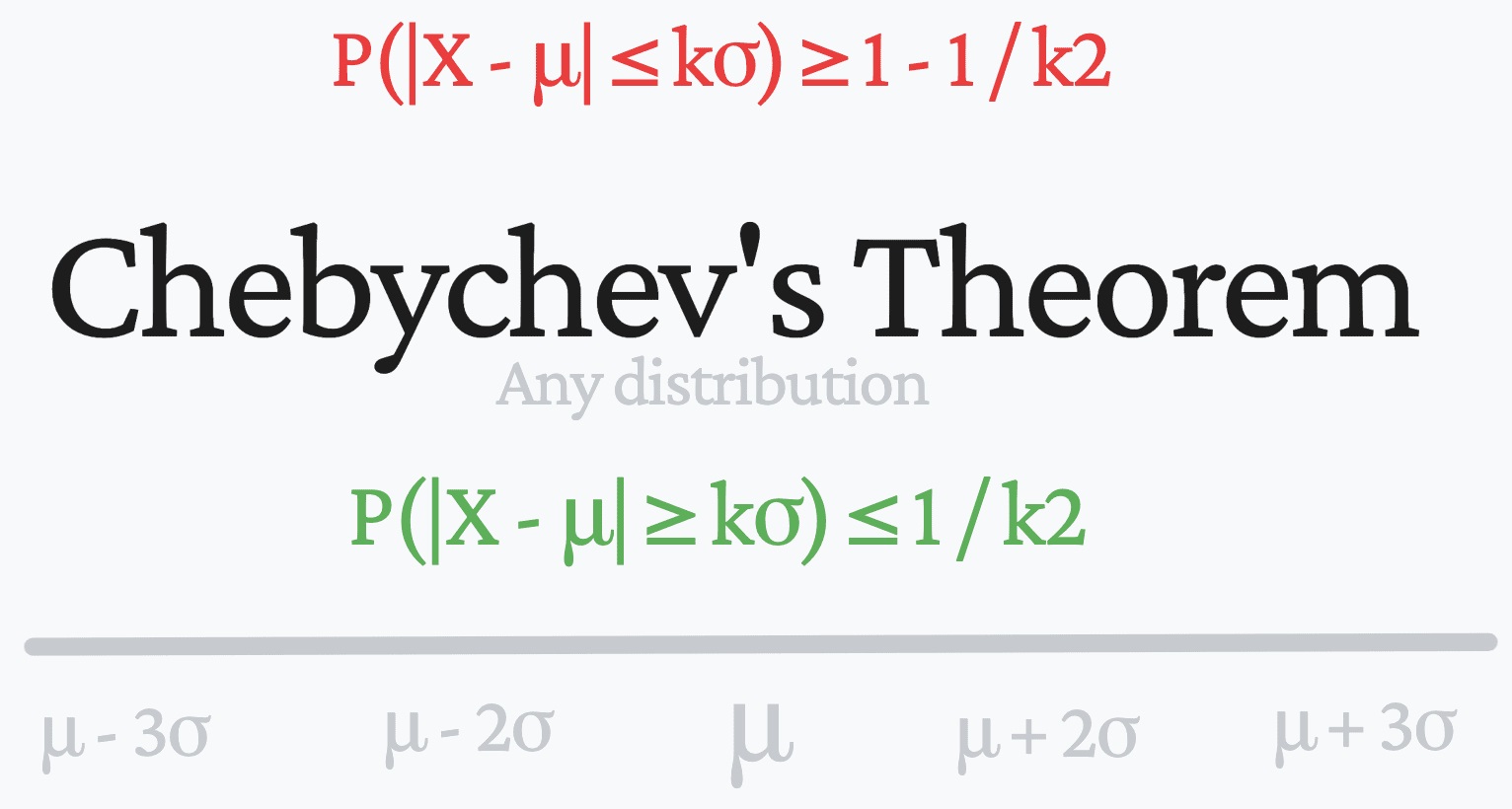
Chebyshev's Inequality is a fundamental concept in probability theory and statistics, providing a powerful tool for understanding the spread of data. Named after the Russian mathematician Pafnuty Chebyshev, this inequality offers valuable insights into the likelihood of observations falling within a certain distance from the mean. By exploring the principles of Chebyshev's Inequality, we can gain a deeper understanding of the dispersion of data points and the probability of events occurring within a specified range. In this article, we will delve into six essential facts about Chebyshev's Inequality, shedding light on its significance and practical applications. From its foundational principles to real-world implications, we will unravel the key aspects of this powerful statistical tool, empowering readers to grasp its relevance in diverse analytical contexts. Let's embark on a journey to uncover the intriguing facets of Chebyshev's Inequality and its role in shaping our understanding of probability and statistics.
Key Takeaways:
- Chebyshev’s Inequality provides a measure of how much data deviates from its average, helping us understand the spread of information and the likelihood of extreme outliers in various fields like finance and quality control.
- This fundamental theorem, named after the Russian mathematician Pafnuty Chebyshev, is a versatile tool applicable to any probability distribution, offering valuable insights into the behavior of random variables and the presence of outliers in data analysis.
Chebyshev's Inequality is a fundamental concept in probability and statistics.
Chebyshev's Inequality is a powerful theorem in probability and statistics that provides a bound on the probability of a random variable deviating from its mean. This inequality is a crucial tool in understanding the dispersion of data and is widely used in various fields, including finance, engineering, and social sciences. Named after the Russian mathematician Pafnuty Chebyshev, this inequality offers valuable insights into the behavior of random variables and is an essential component of statistical analysis.
It provides a measure of how much a random variable deviates from its mean.
Chebyshev's Inequality offers a quantitative measure of the dispersion of data around the mean. It states that for any set of data, regardless of its distribution, a minimum proportion of the data must lie within a certain distance from the mean. This provides a valuable measure of the spread of data and allows statisticians and analysts to assess the likelihood of extreme deviations from the mean.
The inequality is applicable to any probability distribution.
One of the remarkable aspects of Chebyshev's Inequality is its universality. Unlike some statistical theorems that are limited to specific distributions, Chebyshev's Inequality holds true for any probability distribution, making it a versatile and widely applicable tool in statistical analysis.
It is a key concept in understanding the concept of outliers in data analysis.
Chebyshev's Inequality plays a crucial role in identifying and understanding outliers in data analysis. By providing a bound on the proportion of data that can deviate from the mean, it offers valuable insights into the presence and significance of outliers in a dataset. This is particularly important in fields such as finance and quality control, where the identification of outliers is essential for decision-making and risk assessment.
The inequality is expressed in terms of the standard deviation.
In its mathematical form, Chebyshev's Inequality is expressed in terms of the standard deviation of a random variable. This highlights the fundamental relationship between the spread of data and the probability of deviations from the mean, providing a clear and concise expression of the inequality.
It is a foundational concept in probability theory and statistical analysis.
Chebyshev's Inequality holds a central position in probability theory and statistical analysis, serving as a foundational concept that underpins many statistical methods and principles. Its broad applicability and robustness make it an indispensable tool for researchers, analysts, and practitioners across various disciplines.
Chebyshev's Inequality stands as a cornerstone of statistical analysis, offering valuable insights into the dispersion of data and the likelihood of extreme deviations from the mean. This fundamental theorem, named after the Russian mathematician Pafnuty Chebyshev, provides a universal measure of the spread of data and plays a crucial role in identifying outliers and assessing the behavior of random variables. With its broad applicability and foundational significance, Chebyshev's Inequality remains a key concept in probability theory and statistical analysis, empowering researchers and analysts to gain deeper insights into the nature of data and probability distributions.
Conclusion
In conclusion, Chebyshev's Inequality is a powerful tool in probability theory and statistics, providing valuable insights into the spread of data around the mean. Its application extends across various fields, from finance to quality control, enabling analysts to make informed decisions based on the distribution of data. Understanding the six key facts about Chebyshev's Inequality equips individuals with the knowledge to interpret and utilize this fundamental concept effectively. By grasping the significance of the mean and standard deviation, recognizing the proportion of data within a certain range, and comprehending the relationship between dispersion and probability, one can harness the full potential of Chebyshev's Inequality in practical scenarios.
FAQs
Q: What is the primary purpose of Chebyshev's Inequality?A: Chebyshev's Inequality provides a bound on the probability that a random variable deviates from its mean, offering valuable insights into the spread of data and the likelihood of extreme values.
Q: How does Chebyshev's Inequality contribute to statistical analysis?A: Chebyshev's Inequality enables analysts to make probabilistic statements about the dispersion of data, aiding in decision-making processes and risk assessment across various domains.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
