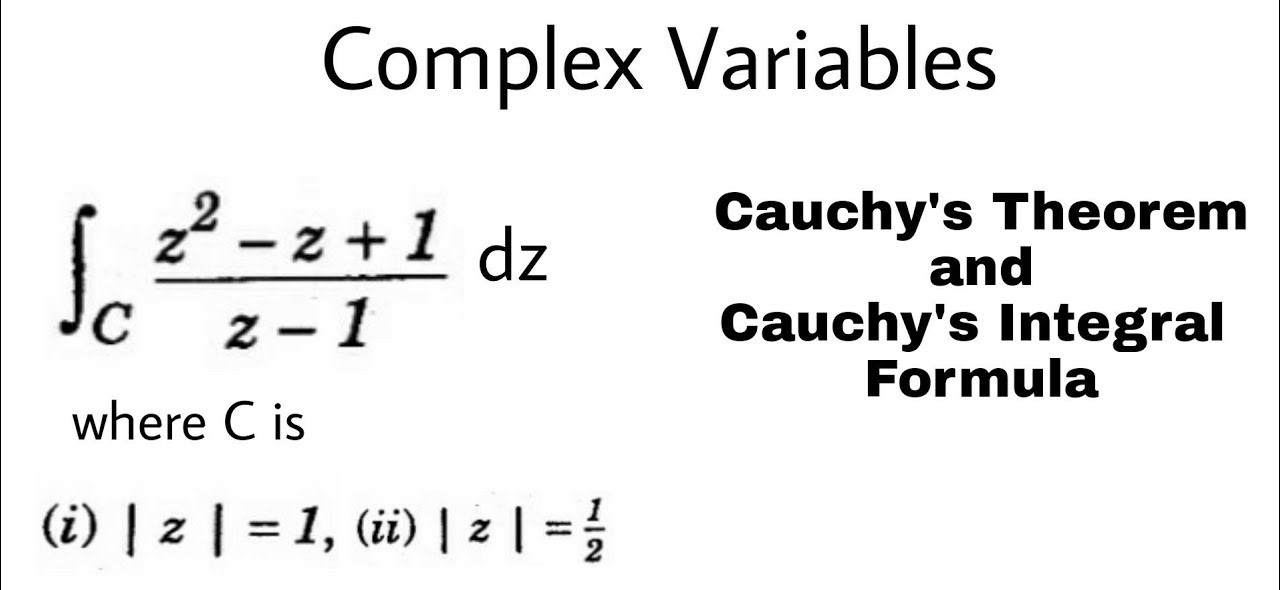
Cauchy's Integral Theorem is a fundamental concept in complex analysis, named after the renowned French mathematician Augustin-Louis Cauchy. This theorem plays a pivotal role in understanding the behavior of complex functions and their integrals. It establishes a profound connection between the values of a function inside a closed contour and its values on the contour itself. By delving into the intricacies of this theorem, mathematicians and scientists have unlocked a deeper comprehension of complex analysis and its applications in various fields.
In this article, we will explore 10 essential facts about Cauchy's Integral Theorem, shedding light on its significance, applications, and implications in the realm of mathematical sciences. From its historical origins to its practical utility in solving complex problems, each fact will unravel a unique aspect of this theorem, providing a comprehensive understanding of its relevance and impact. So, let's embark on a journey to unravel the mysteries and marvels of Cauchy's Integral Theorem.
Key Takeaways:
- Cauchy’s Integral Theorem connects complex function integrals to their boundary values, helping us solve complex integration problems and understand the behavior of holomorphic functions in a cool and powerful way!
- This theorem is like a superhero in the world of math, helping us understand complex functions, integrals, and even the behavior of things in the real world like fluid dynamics and electromagnetic theory.
Cauchy's Integral Theorem is a fundamental concept in complex analysis.
Cauchy's Integral Theorem, a cornerstone of complex analysis, plays a pivotal role in understanding the behavior of complex functions and their integration over closed curves. This theorem, formulated by the French mathematician Augustin-Louis Cauchy, has far-reaching implications in various branches of mathematics and physics, making it a crucial topic for students and researchers alike.
It establishes a relationship between the integral of a complex function and its values on the boundary of a region.
Cauchy's Integral Theorem states that if a function is holomorphic within a simply connected region and continuous on its boundary, then the integral of the function over a closed curve within the region is equal to zero. This profound connection between the integral of a complex function and its boundary values provides a powerful tool for evaluating complex integrals and understanding the intricate behavior of holomorphic functions.
The theorem is a key component of the theory of complex variables.
Cauchy's Integral Theorem forms the bedrock of the theory of complex variables, offering deep insights into the behavior of complex functions and their integration. It serves as a fundamental building block for more advanced concepts in complex analysis, such as Cauchy's Residue Theorem and the theory of analytic functions, making it an indispensable tool for mathematicians and physicists working in the field of complex analysis.
It is widely used in physics and engineering.
The applications of Cauchy's Integral Theorem extend beyond mathematics and are prevalent in the fields of physics and engineering. This theorem finds extensive use in solving problems related to potential theory, fluid dynamics, electromagnetic theory, and other areas where complex variables and functions play a crucial role. Its utility in these practical domains underscores the significance of understanding and applying Cauchy's Integral Theorem in real-world scenarios.
The theorem has connections to the concept of path independence.
Cauchy's Integral Theorem is intimately linked to the concept of path independence, wherein the value of a line integral depends only on the endpoints of the path and not the specific path taken. This connection provides a profound geometric and analytical understanding of complex integrals and their behavior, shedding light on the underlying principles governing the integration of complex functions over closed curves.
It forms the basis for the Cauchy Integral Formula.
Cauchy's Integral Theorem serves as the foundation for the development of the Cauchy Integral Formula, a powerful tool for evaluating complex integrals and expressing holomorphic functions in terms of their boundary values. This formula, derived from the insights of Cauchy's theorem, has wide-ranging applications in complex analysis and serves as a fundamental tool for solving complex integration problems.
The theorem is essential for understanding singularities and residues of complex functions.
In the study of complex analysis, Cauchy's Integral Theorem plays a crucial role in understanding the behavior of singularities and residues of complex functions. By providing a framework for evaluating integrals over closed curves, this theorem offers valuable insights into the structure of singularities and the calculation of residues, which are vital in various mathematical and physical contexts.
It has implications for the global behavior of holomorphic functions.
Cauchy's Integral Theorem has profound implications for the global behavior of holomorphic functions, offering valuable insights into the connectivity and analytic properties of complex functions. By establishing a relationship between the integral of a holomorphic function and its boundary values, this theorem contributes to our understanding of the overarching behavior of complex functions in the complex plane.
The theorem is a fundamental topic in mathematical education.
Cauchy's Integral Theorem holds a central position in mathematical education, serving as a fundamental concept in complex analysis courses at the undergraduate and graduate levels. Its significance in shaping the understanding of complex functions, integrals, and the theory of residues makes it an essential topic for students pursuing studies in mathematics, physics, and engineering.
It exemplifies the elegance and power of complex analysis.
Cauchy's Integral Theorem stands as a testament to the elegance and power of complex analysis, showcasing the profound connections between complex functions, integrals, and geometric properties. Its far-reaching implications and applications underscore the beauty and utility of complex analysis, inspiring further exploration and research in this captivating field of mathematics.
By delving into the intricacies of Cauchy's Integral Theorem, we gain a deeper appreciation for the profound connections between complex functions, integrals, and the global behavior of holomorphic functions, highlighting the enduring significance of this fundamental theorem in the realm of mathematics and its practical applications in physics and engineering.
Conclusion
In conclusion, Cauchy's Integral Theorem is a fundamental concept in complex analysis that has far-reaching implications in various fields, including mathematics, physics, and engineering. Understanding the key principles and applications of this theorem is crucial for anyone delving into advanced mathematical studies or seeking to comprehend the intricate behavior of complex functions. By grasping the significance of Cauchy's Integral Theorem and its related concepts, individuals can gain valuable insights into the behavior of analytic functions and their corresponding integrals within complex domains. This theorem's elegant mathematical framework and practical implications underscore its enduring relevance in modern scientific and mathematical discourse.
FAQs
What is the significance of Cauchy's Integral Theorem?Cauchy's Integral Theorem holds significant importance in complex analysis as it establishes a profound connection between the analyticity of functions and the behavior of their integrals within closed contours. This theorem forms the basis for numerous advanced concepts in mathematics and has wide-ranging applications in physics and engineering.
How does Cauchy's Integral Theorem relate to complex functions?Cauchy's Integral Theorem is closely linked to the behavior of complex functions, particularly those that are analytic within a given domain. The theorem provides crucial insights into the integrals of such functions over closed contours, shedding light on their properties and enabling the derivation of valuable results in complex analysis.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
