The Hairy Ball Theorem is a fascinating concept in the field of mathematics that has captured the curiosity of both mathematicians and enthusiasts alike. This theorem, which may sound whimsical at first, holds profound implications in various mathematical and scientific disciplines. Understanding the essence of the Hairy Ball Theorem requires delving into the intricate interplay between topology, geometry, and vector fields. As we embark on this exploration, we will uncover the tenacious nature of this theorem and its relevance in diverse real-world applications. Let's unravel the enigma of the Hairy Ball Theorem and discover the captivating insights it offers into the fabric of our mathematical universe.
Key Takeaways:
- The Hairy Ball Theorem shows that you can’t comb a hairy ball flat without creating a cowlick. It’s like trying to smooth out a messy bedhead – it just won’t work!
- This theorem is like a fascinating puzzle that mathematicians love to solve. It helps us understand how things move on a sphere and has cool applications in science and technology.
The Hairy Ball Theorem is a Fundamental Concept in Mathematics
The Hairy Ball Theorem is a fundamental concept in mathematics that has intrigued and puzzled mathematicians for decades. This theorem, which is a fundamental result in algebraic topology, holds a unique place in the realm of mathematical theory. It is a captivating subject that has sparked curiosity and fascination among mathematicians and enthusiasts alike. Let's delve into 10 intriguing facts about the Hairy Ball Theorem that shed light on its significance and implications.
The Theorem Addresses the Impossibility of Combing a Hairy Ball Flat
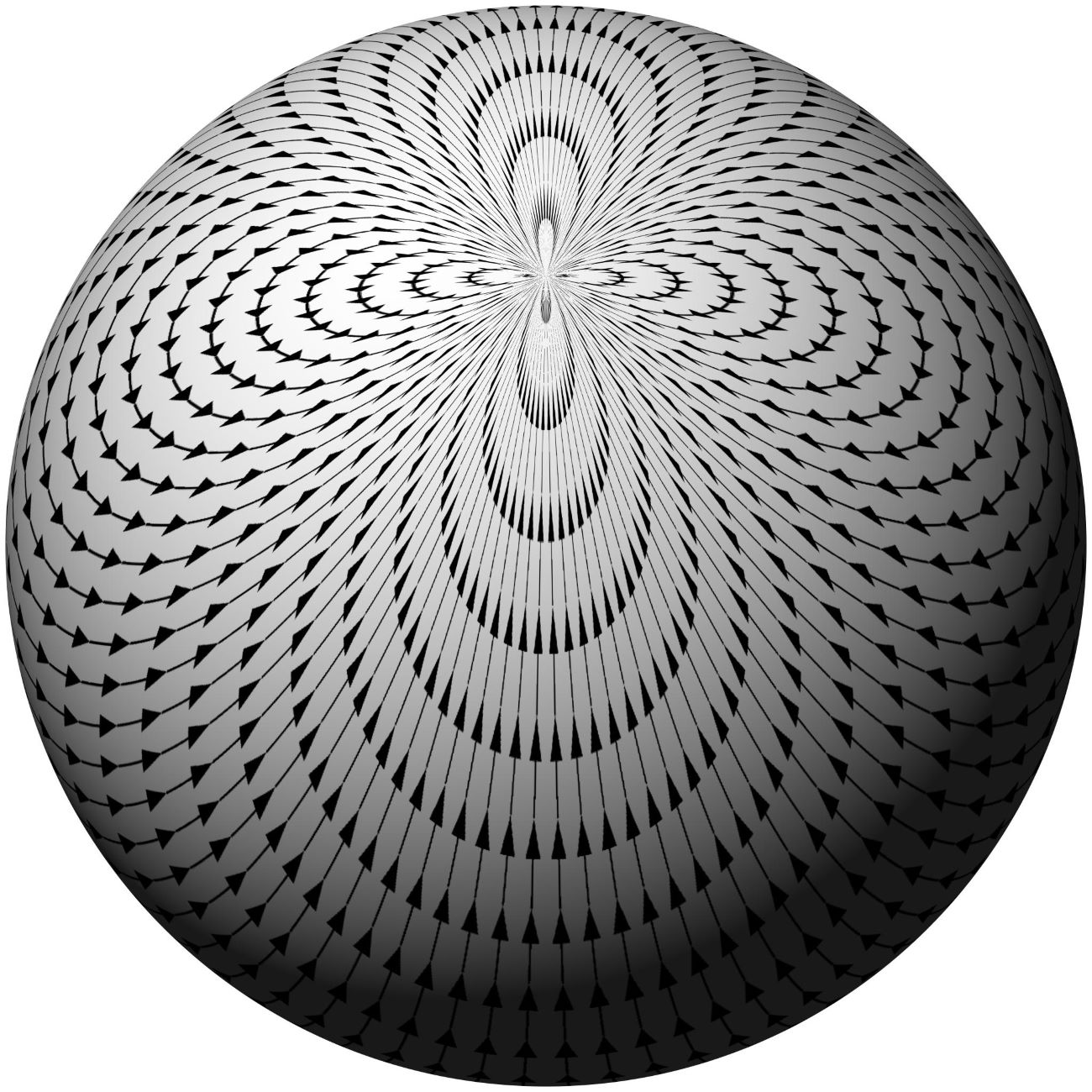
The Hairy Ball Theorem addresses the impossibility of combing a hairy ball flat without creating a cowlick, a point where the hair sticks straight up. This concept may seem whimsical at first, but it has profound implications in various fields, including computer graphics, meteorology, and robotics. The theorem's essence lies in its elegant and thought-provoking nature, captivating the minds of mathematicians and inspiring further exploration into its applications.
It Was First Conceived in the 19th Century
The Hairy Ball Theorem was first conceived in the 19th century, marking a significant milestone in the development of mathematical topology. This theorem has since become a cornerstone of modern mathematics, influencing diverse areas such as differential geometry, dynamical systems, and theoretical physics. Its enduring relevance underscores its status as a timeless mathematical principle that continues to shape the landscape of mathematical inquiry.
The Theorem Poses a Fascinating Challenge
The Hairy Ball Theorem poses a fascinating challenge that has captivated mathematicians and problem solvers. Its enigmatic nature and far-reaching implications have spurred extensive research and exploration, leading to profound insights and discoveries in the realm of mathematics. This theorem stands as a testament to the enduring allure of mathematical puzzles and the boundless potential for innovation and discovery within the field.
It Illuminates the Intricacies of Vector Fields
The Hairy Ball Theorem illuminates the intricacies of vector fields, shedding light on the behavior of these fundamental mathematical constructs. By elucidating the constraints and properties of vector fields on a sphere, this theorem offers profound insights into the underlying principles governing these dynamic systems. Its implications reverberate across various disciplines, enriching our understanding of complex phenomena and inspiring new avenues of inquiry.
The Theorem Holds Implications for Diverse Fields
The Hairy Ball Theorem holds implications for diverse fields, including physics, computer science, and engineering. Its far-reaching influence underscores the interconnectedness of mathematical theory and its applications in real-world scenarios. By unraveling the fundamental constraints of vector fields on a spherical surface, this theorem provides invaluable insights that resonate across a spectrum of scientific and technological domains.
It Reflects the Beauty of Mathematical Abstraction
The Hairy Ball Theorem reflects the beauty of mathematical abstraction, showcasing the elegance and depth of theoretical concepts. Its profound implications transcend the confines of pure mathematics, permeating various branches of science and engineering with its far-reaching influence. This theorem stands as a testament to the enduring allure of mathematical theory and its capacity to unveil the underlying principles governing the natural world.
The Theorem Inspires Thought-Provoking Questions
The Hairy Ball Theorem inspires thought-provoking questions that challenge conventional wisdom and spark intellectual curiosity. Its enigmatic nature invites contemplation and exploration, prompting mathematicians and scholars to delve into its intricacies and implications. This theorem serves as a catalyst for deepening our understanding of fundamental mathematical concepts and their broader significance in the context of scientific inquiry.
It Continues to Fascinate Mathematicians and Scholars
The Hairy Ball Theorem continues to fascinate mathematicians and scholars, serving as a wellspring of inspiration and inquiry. Its enduring relevance and enigmatic nature ensure that it remains a subject of ongoing exploration and fascination within the mathematical community. This theorem stands as a testament to the timeless allure of mathematical theory and its capacity to engender profound insights and discoveries.
The Theorem Embodies the Intriguing Nature of Mathematical Inquiry
The Hairy Ball Theorem embodies the intriguing nature of mathematical inquiry, encapsulating the spirit of exploration and discovery that defines the field of mathematics. Its enigmatic essence and far-reaching implications underscore the enduring fascination and relevance of mathematical theory in shaping our understanding of the natural world. This theorem stands as a testament to the boundless potential for innovation and insight that permeates the realm of mathematical inquiry.
The Hairy Ball Theorem, with its captivating intricacies and profound implications, stands as a testament to the enduring allure of mathematical theory and its capacity to inspire curiosity and exploration. As mathematicians continue to unravel its enigmatic nature, this timeless theorem remains a beacon of intellectual inquiry and a source of inspiration for future generations of problem solvers and innovators.
Conclusion
In conclusion, the Hairy Ball Theorem is a fascinating concept with profound implications in various fields, including mathematics, physics, and computer science. Its elegant yet counterintuitive nature challenges our understanding of continuous vector fields and has sparked numerous applications and extensions. From its humble origins to its far-reaching consequences, the Hairy Ball Theorem continues to captivate mathematicians and enthusiasts alike, serving as a testament to the beauty and complexity of mathematical reasoning.
FAQs
What is the Hairy Ball Theorem?The Hairy Ball Theorem, a fundamental concept in mathematics, states that it is impossible to comb the hair on a hairy ball without creating a cowlick, or a point where the hair stands straight up.
What are the practical implications of the Hairy Ball Theorem?The theorem has significant implications in various fields, such as physics, computer graphics, and robotics. For instance, it influences the design of 3D computer graphics and the development of algorithms for controlling robotic systems, highlighting its real-world relevance.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.