8 Facts You Must Know About Liouville’s Theorem

Liouville's Theorem: Unveiling the Mysteries of Dynamical Systems

Liouville's Theorem is a fundamental concept in the realm of dynamical systems, offering profound insights into the behavior of these complex systems. Let's delve into 8 intriguing facts that shed light on this captivating theorem.

Liouville's Theorem is a Pillar of Hamiltonian Mechanics

At its core, Liouville's Theorem serves as a cornerstone of Hamiltonian mechanics, providing a crucial framework for understanding the evolution of dynamical systems in phase space.

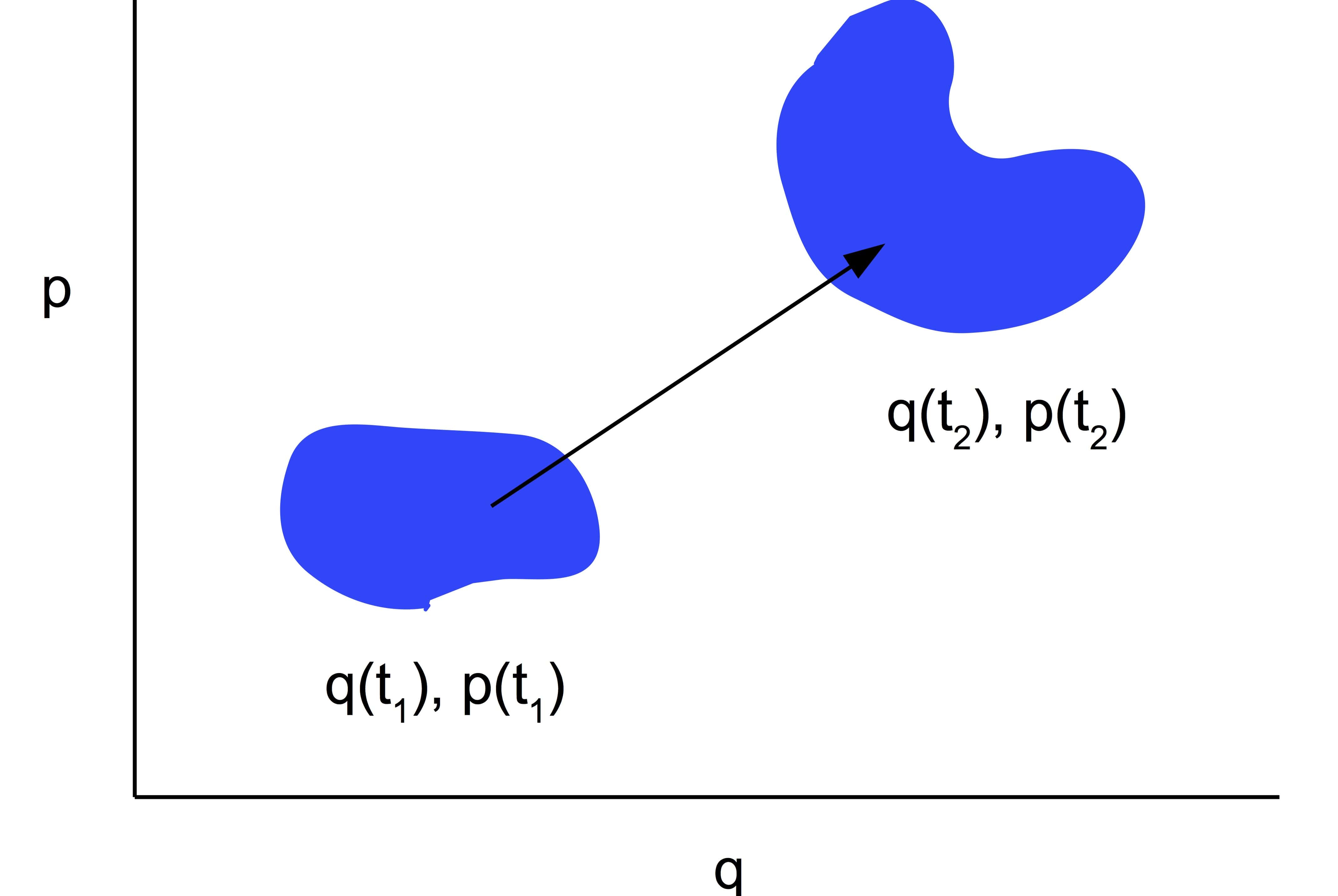
It Preserves Phase Space Volume

One of the most remarkable aspects of Liouville's Theorem is its preservation of phase space volume, signifying that as a dynamical system evolves, the volume of its phase space remains constant—a concept that has far-reaching implications in the study of physical systems.

Liouville's Theorem Reflects the Conservation of Phase Space Density

By maintaining the volume in phase space, Liouville's Theorem inherently reflects the conservation of phase space density, offering profound insights into the behavior and evolution of dynamical systems.

It Illuminates the Notion of Integrability

Liouville's Theorem plays a pivotal role in elucidating the concept of integrability within dynamical systems, providing a fundamental understanding of the behavior of these intricate systems.

Liouville's Theorem is Inextricably Linked to Symplectic Geometry

This theorem is intricately intertwined with symplectic geometry, a branch of mathematics that underpins the geometric structure of Hamiltonian mechanics, further highlighting its significance in the field.

It Empowers the Understanding of Ergodic Theory

Liouville's Theorem empowers the exploration of ergodic theory, a captivating area of study that delves into the long-term behavior of dynamical systems, offering profound insights into their intricate dynamics.

Liouville's Theorem: A Timeless Enigma

This theorem stands as a timeless enigma, captivating mathematicians and physicists alike with its profound implications for the understanding of dynamical systems, making it an indispensable concept in the realm of mathematical sciences.

Liouville's Theorem encapsulates the essence of dynamical systems, offering a profound understanding of their behavior and evolution. Its far-reaching implications extend into various branches of mathematics and physics, making it a cornerstone of theoretical frameworks and practical applications. As we unravel the mysteries of Liouville's Theorem, we gain invaluable insights into the intricate dynamics of the world around us.

Conclusion

In conclusion, Liouville's Theorem is a fundamental concept in mathematical physics and dynamical systems. It provides crucial insights into the behavior of systems governed by Hamiltonian dynamics, shedding light on the preservation of phase space volume under time evolution. Understanding the implications of Liouville's Theorem is essential for grasping the conservation of information in classical mechanics and the broader applications in statistical mechanics and quantum mechanics. By recognizing the significance of this theorem, we gain a deeper appreciation for the underlying principles that govern the dynamics of physical systems.

FAQs

What is the significance of Liouville's Theorem?Liouville's Theorem holds significant importance in the realm of mathematical physics and dynamical systems. It elucidates the preservation of phase space volume under Hamiltonian dynamics, providing crucial insights into the conservation of information in classical mechanics.

How does Liouville's Theorem relate to other branches of physics?Liouville's Theorem has far-reaching implications, extending its relevance to statistical mechanics and quantum mechanics. Its fundamental principles underpin the conservation of information and offer valuable insights into the behavior of physical systems across various domains of physics.