40 Facts About Orthogonal

Orthogonal: What Does It Mean?

Orthogonal is a term often used in mathematics, science, and engineering. It describes things that are at right angles to each other. This concept is crucial in various fields, from geometry to data analysis.

1. 01

   Orthogonal comes from the Greek words "orthos" (right) and "gonia" (angle).

2. 02

   In geometry, two lines are orthogonal if they intersect at a 90-degree angle.

3. 03

   Orthogonal vectors in a vector space have a dot product of zero.

4. 04

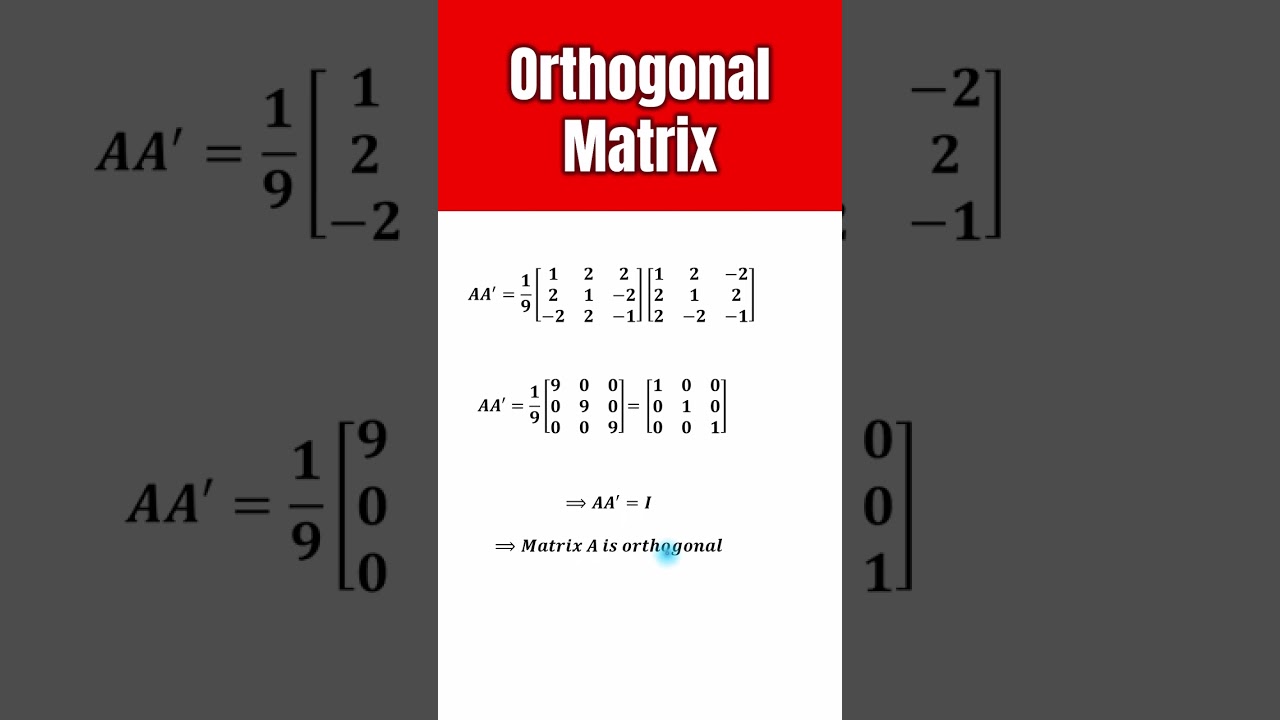
   Orthogonal matrices are square matrices whose rows and columns are orthonormal vectors.

5. 05

   In statistics, orthogonal variables are uncorrelated, meaning their covariance is zero.

Orthogonal in Mathematics

Mathematics heavily relies on the concept of orthogonality. It helps in simplifying complex problems and finding solutions efficiently.

6. 06

   Orthogonal transformations preserve the length of vectors and the angle between them.

7. 07

   Orthogonal polynomials are a sequence of polynomials that are orthogonal to each other with respect to a given inner product.

8. 08

   The Gram-Schmidt process is a method for orthogonalizing a set of vectors in an inner product space.

9. 09

   Orthogonal functions are functions that are orthogonal under some inner product.

10. 10

    Fourier series use orthogonal functions (sines and cosines) to represent periodic functions.

Orthogonal in Engineering

Engineering applications often use orthogonality to design and analyze systems. It ensures efficiency and accuracy in various processes.

11. 11

    Orthogonal frequency-division multiplexing (OFDM) is a method of encoding digital data on multiple carrier frequencies.

12. 12

    Orthogonal coding is used in communication systems to minimize interference.

13. 13

    Orthogonal designs in experiments help in studying the effects of multiple factors simultaneously.

14. 14

    Orthogonal projections are used in computer graphics to project 3D objects onto 2D screens.

15. 15

    Orthogonal signals in telecommunications are signals that do not interfere with each other.

Orthogonal in Computer Science

Computer science uses orthogonality to improve software design and data management. It helps in creating modular and maintainable systems.

16. 16

    Orthogonal programming languages have features that operate independently of each other.

17. 17

    Orthogonal persistence refers to the persistence of data that is independent of the data type.

18. 18

    Orthogonal arrays are used in software testing to ensure comprehensive test coverage.

19. 19

    Orthogonal instruction sets in CPUs allow for more efficient processing.

20. 20

    Orthogonal data structures are designed to minimize dependencies between different parts of the data.

Orthogonal in Physics

Physics uses orthogonality to describe various phenomena and solve complex equations. It helps in understanding the fundamental principles of the universe.

21. 21

    Orthogonal wave functions are used in quantum mechanics to describe the state of a system.

22. 22

    Orthogonal coordinates are coordinate systems where the coordinate lines intersect at right angles.

23. 23

    Orthogonal polarization refers to light waves that oscillate in perpendicular directions.

24. 24

    Orthogonal modes in waveguides are independent modes of propagation.

25. 25

    Orthogonal decompositions are used in signal processing to separate signals into independent components.

Orthogonal in Statistics

Statistics uses orthogonality to simplify data analysis and improve the accuracy of results. It helps in identifying relationships between variables.

26. 26

    Orthogonal contrasts are used in analysis of variance (ANOVA) to compare different groups.

27. 27

    Orthogonal regression minimizes the sum of the squared perpendicular distances from the data points to the regression line.

28. 28

    Orthogonal rotation in factor analysis simplifies the interpretation of factors.

29. 29

    Orthogonal designs in experiments ensure that the effects of different factors can be estimated independently.

30. 30

    Orthogonal projections are used in multivariate analysis to reduce the dimensionality of data.

Orthogonal in Everyday Life

Orthogonality isn't just for scientists and engineers. It appears in everyday life, often without us even realizing it.

31. 31

    Orthogonal streets in city planning create a grid layout, making navigation easier.

32. 32

    Orthogonal cutting in machining refers to cutting at a right angle to the workpiece.

33. 33

    Orthogonal joints in carpentry ensure strong and stable connections.

34. 34

    Orthogonal layouts in graphic design create clean and organized visuals.

35. 35

    Orthogonal movements in robotics allow for precise and controlled actions.

Fun Facts about Orthogonal

Orthogonality has some interesting and fun aspects that might surprise you.

36. 36

    Orthogonal Latin squares are used in puzzles and games like Sudoku.

37. 37

    Orthogonal art uses right angles and geometric shapes to create visually appealing designs.

38. 38

    Orthogonal music refers to compositions where different musical lines are independent yet harmonious.

39. 39

    Orthogonal thinking involves looking at problems from different, often perpendicular, perspectives.

40. 40

    Orthogonal symmetry in nature can be seen in crystals and other naturally occurring structures.

The Final Word on Orthogonal

Orthogonal concepts might sound complex, but they’re everywhere in daily life. From the way we design buildings to how we solve problems, orthogonality helps us keep things simple and efficient. It’s all about independence and clarity, making sure one thing doesn’t mess with another. Whether you’re into math, computer science, or just curious about how things work, understanding orthogonality can give you a fresh perspective. It’s like having a secret tool that makes complicated stuff easier to handle. So next time you hear the term, you’ll know it’s not just fancy jargon—it’s a practical idea that shapes our world. Keep an eye out for it, and you’ll start seeing orthogonal relationships everywhere.