34 Facts About Polynomial

What is a Polynomial?

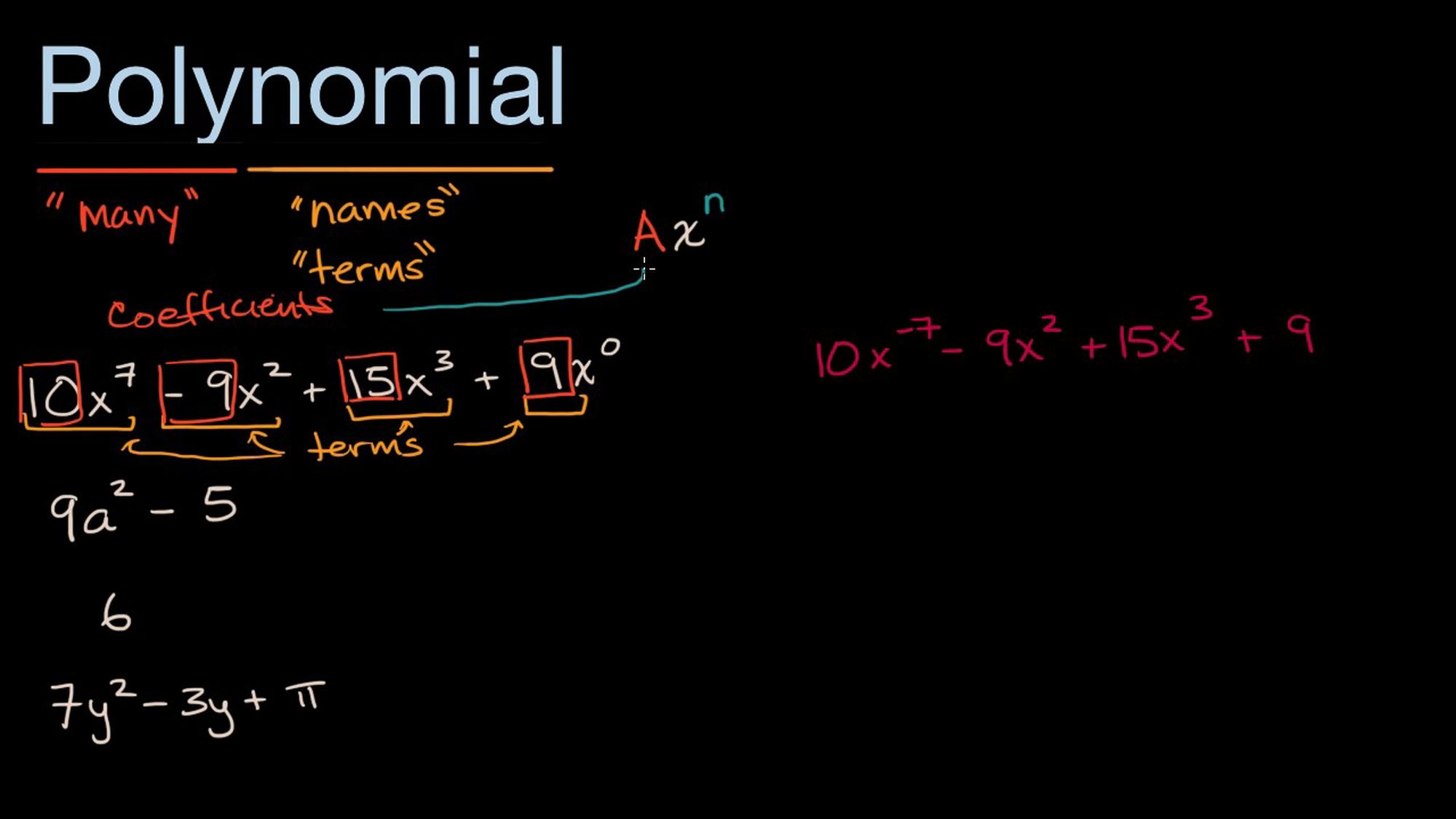
Polynomials are mathematical expressions involving a sum of powers in one or more variables multiplied by coefficients. They play a crucial role in algebra and calculus.

1. 01

   Definition: A polynomial is an expression of the form (a_nx^n + a_{n-1}x^{n-1} + … + a_1x + a_0), where (a_n, a_{n-1}, …, a_1, a_0) are coefficients, and (n) is a non-negative integer.

2. 02

   Origin: The term "polynomial" comes from the Greek words "poly," meaning "many," and "nomial," meaning "terms."

3. 03

   Degree: The degree of a polynomial is the highest power of the variable in the expression. For example, (3x^4 + 2x^3 + x + 7) has a degree of 4.

4. 04

   Coefficients: These are the numbers in front of the variables in a polynomial. In (5x^3 + 3x^2 + 2x + 1), the coefficients are 5, 3, 2, and 1.

Types of Polynomials

Polynomials can be classified based on their degree and the number of terms they contain.

5. 05

   Monomial: A polynomial with just one term, like (7x^3).

6. 06

   Binomial: A polynomial with two terms, such as (3x^2 + 2x).

7. 07

   Trinomial: A polynomial with three terms, for example, (x^2 + 5x + 6).

8. 08

   Quadratic Polynomial: A polynomial of degree 2, like (ax^2 + bx + c).

9. 09

   Cubic Polynomial: A polynomial of degree 3, such as (ax^3 + bx^2 + cx + d).

Operations on Polynomials

Polynomials can undergo various operations, including addition, subtraction, multiplication, and division.

10. 10

    Addition: Adding polynomials involves combining like terms. For instance, ((3x^2 + 2x) + (x^2 + 4x) = 4x^2 + 6x).

11. 11

    Subtraction: Subtracting polynomials also involves combining like terms. For example, ((5x^3 – 2x) – (3x^3 + x) = 2x^3 – 3x).

12. 12

    Multiplication: Multiplying polynomials involves distributing each term in the first polynomial to every term in the second. For instance, ((x + 2)(x + 3) = x^2 + 5x + 6).

13. 13

    Division: Polynomial division is similar to long division with numbers. For example, dividing (x^3 + 2x^2 + x + 1) by (x + 1) yields (x^2 + x + 1).

Polynomial Functions

Polynomials can be used to define functions, which have various applications in mathematics and science.

14. 14

    Polynomial Function: A function that can be represented by a polynomial equation, such as (f(x) = 2x^3 – x^2 + 3x – 5).

15. 15

    Roots: The values of (x) that make the polynomial equal to zero. For (x^2 – 5x + 6 = 0), the roots are (x = 2) and (x = 3).

16. 16

    Graphing: The graph of a polynomial function is a smooth, continuous curve. The degree of the polynomial determines the number of turning points.

17. 17

    End Behavior: The behavior of the graph as (x) approaches positive or negative infinity. For example, the graph of (x^2) opens upwards, while (x^3) has one end going up and the other down.

Applications of Polynomials

Polynomials are used in various fields, from physics to economics.

18. 18

    Physics: Polynomials describe motion, such as the trajectory of a projectile.

19. 19

    Economics: They model cost functions and revenue functions.

20. 20

    Engineering: Used in control systems and signal processing.

21. 21

    Computer Science: Algorithms for polynomial time complexity.

22. 22

    Statistics: Polynomial regression models relationships between variables.

Special Polynomials

Some polynomials have unique properties and names.

23. 23

    Constant Polynomial: A polynomial of degree 0, like (5).

24. 24

    Linear Polynomial: A polynomial of degree 1, such as (2x + 3).

25. 25

    Quadratic Polynomial: A polynomial of degree 2, like (x^2 – 4x + 4).

26. 26

    Cubic Polynomial: A polynomial of degree 3, such as (x^3 – 3x^2 + 3x – 1).

27. 27

    Quartic Polynomial: A polynomial of degree 4, like (x^4 – 2x^3 + x^2 – x + 1).

Polynomial Theorems and Properties

Several important theorems and properties are associated with polynomials.

28. 28

    Fundamental Theorem of Algebra: Every non-zero polynomial equation has at least one complex root.

29. 29

    Remainder Theorem: When a polynomial (f(x)) is divided by (x – c), the remainder is (f(c)).

30. 30

    Factor Theorem: (x – c) is a factor of (f(x)) if and only if (f(c) = 0).

31. 31

    Descartes' Rule of Signs: Determines the number of positive and negative real roots of a polynomial.

32. 32

    Vieta's Formulas: Relate the coefficients of a polynomial to sums and products of its roots.

Fun Facts about Polynomials

Polynomials have some interesting and fun aspects too.

33. 33

    Symmetry: The graph of an even-degree polynomial is symmetric about the y-axis, while an odd-degree polynomial is symmetric about the origin.

34. 34

    Pascal's Triangle: Used to find coefficients in the expansion of ((x + y)^n).

Final Thoughts on Polynomials

Polynomials are more than just algebraic expressions. They play a crucial role in various fields, from physics to computer science. Understanding their properties and applications can open doors to solving complex problems. Whether you're calculating the trajectory of a rocket or optimizing algorithms, polynomials are indispensable tools.

Remember, the degree of a polynomial tells you the highest power of the variable, and the coefficients are the numbers in front of those variables. Factoring polynomials can simplify equations, making them easier to solve.

So next time you encounter a polynomial, don't just see it as a bunch of numbers and letters. Recognize its potential to unlock solutions and drive innovation. Keep exploring, keep questioning, and you'll find that polynomials are not just a part of math—they're a part of life.