Hyperbolic geometry might sound like something from a sci-fi movie, but it's a fascinating branch of mathematics with real-world applications. Unlike the flat surfaces of Euclidean geometry, hyperbolic geometry deals with curved spaces. Imagine a world where parallel lines can diverge or converge, and the angles of a triangle add up to less than 180 degrees. Why should you care about hyperbolic geometry? Because it helps in understanding complex structures in nature, like coral reefs and even the universe's shape. Plus, it's used in computer graphics, art, and architecture. Ready to dive into 26 mind-blowing facts about hyperbolic geometry? Let's get started!
What is Hyperbolic Geometry?
Hyperbolic geometry is a fascinating branch of mathematics that diverges from the familiar Euclidean geometry. It explores the properties and relations of points, lines, and angles in a hyperbolic plane, where the parallel postulate of Euclidean geometry does not hold.
-
Non-Euclidean Geometry: Hyperbolic geometry is a type of non-Euclidean geometry. Unlike Euclidean geometry, it allows for multiple parallel lines to pass through a single point outside a given line.
-
Curved Space: In hyperbolic geometry, the space is negatively curved. This means that the sum of the angles of a triangle is always less than 180 degrees.
-
Hyperbolic Plane: The hyperbolic plane can be represented in several models, such as the Poincaré disk model and the hyperboloid model. Each model provides a different way to visualize hyperbolic space.
Historical Background
Understanding the history of hyperbolic geometry helps appreciate its development and significance in mathematics.
-
19th Century Discovery: Hyperbolic geometry was independently discovered by Nikolai Lobachevsky and János Bolyai in the early 19th century.
-
Gauss's Contribution: Carl Friedrich Gauss also worked on hyperbolic geometry but did not publish his findings. He recognized the importance of this new geometry and corresponded with Lobachevsky and Bolyai.
-
Revolutionary Impact: The discovery of hyperbolic geometry challenged the long-held belief that Euclidean geometry was the only true geometry, revolutionizing mathematical thought.
Key Properties
Hyperbolic geometry has unique properties that distinguish it from Euclidean geometry.
-
Parallel Lines: In hyperbolic geometry, through a point not on a given line, there are infinitely many lines that do not intersect the given line.
-
Angle Sum of Triangles: The sum of the angles of a triangle in hyperbolic space is always less than 180 degrees. The smaller the area of the triangle, the closer the sum is to 180 degrees.
-
Area of Triangles: The area of a triangle in hyperbolic geometry is proportional to the deficit of the angle sum from 180 degrees.
Applications in Science
Hyperbolic geometry is not just a theoretical construct; it has practical applications in various fields.
-
Relativity Theory: Albert Einstein's theory of general relativity uses hyperbolic geometry to describe the curvature of spacetime around massive objects.
-
Cosmology: Hyperbolic geometry helps cosmologists understand the shape and expansion of the universe, particularly in models of an open universe.
-
Art and Design: Artists like M.C. Escher have used hyperbolic geometry to create visually stunning works that explore infinite patterns and shapes.
Models of Hyperbolic Geometry
Different models help visualize and understand hyperbolic geometry.
-
Poincaré Disk Model: This model represents the hyperbolic plane within a disk, where lines are represented by arcs that intersect the boundary circle at right angles.
-
Hyperboloid Model: In this model, the hyperbolic plane is represented as a surface in three-dimensional space, resembling a saddle shape.
-
Klein Model: The Klein model represents the hyperbolic plane within a disk, but lines are represented by straight chords of the disk.
Mathematical Concepts
Several mathematical concepts are unique to hyperbolic geometry.
-
Hyperbolic Distance: Distance in hyperbolic geometry is measured differently than in Euclidean geometry, often using logarithmic functions.
-
Geodesics: The shortest path between two points in hyperbolic space is called a geodesic, which can appear as a curve in Euclidean representations.
-
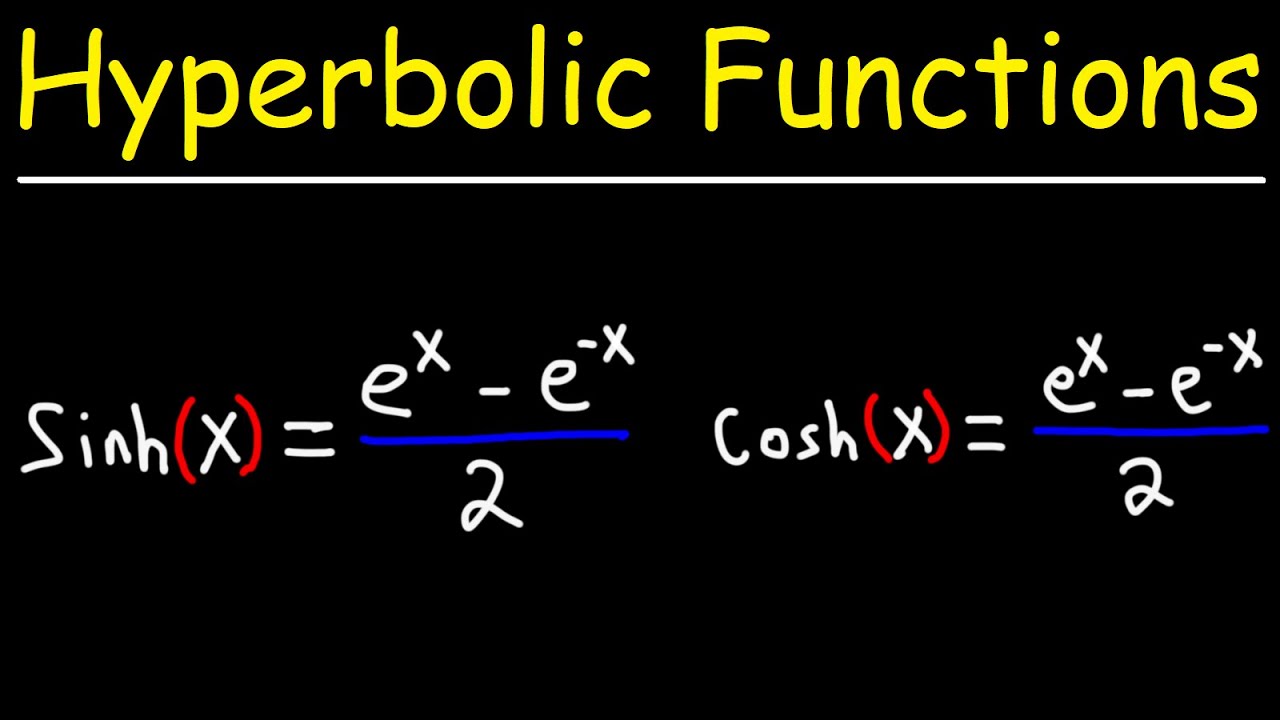
Hyperbolic Trigonometry: Trigonometric functions in hyperbolic geometry differ from those in Euclidean geometry, leading to unique identities and relationships.
Hyperbolic Geometry in Nature
Nature often exhibits patterns and structures that can be described using hyperbolic geometry.
-
Coral Reefs: The branching patterns of coral reefs can be modeled using hyperbolic geometry, reflecting their complex and intricate structures.
-
Leaf Venation: Some plants exhibit leaf venation patterns that resemble hyperbolic structures, optimizing nutrient distribution.
-
Spider Webs: Certain spider webs display hyperbolic patterns, allowing for efficient prey capture and structural stability.
Educational Importance
Learning about hyperbolic geometry can enhance mathematical understanding and problem-solving skills.
-
Critical Thinking: Studying hyperbolic geometry encourages critical thinking and challenges students to question and explore beyond traditional Euclidean concepts.
-
Mathematical Rigor: Hyperbolic geometry requires a high level of mathematical rigor, helping students develop precision and logical reasoning.
-
Interdisciplinary Connections: Understanding hyperbolic geometry can lead to insights in other disciplines, such as physics, art, and computer science.
Modern Research
Ongoing research continues to uncover new aspects and applications of hyperbolic geometry.
-
Quantum Computing: Researchers are exploring the use of hyperbolic geometry in quantum computing, potentially leading to breakthroughs in computational power and efficiency.
-
Network Theory: Hyperbolic geometry is used in network theory to model complex networks, such as the internet, social networks, and biological systems.
Final Thoughts on Hyperbolic Geometry
Hyperbolic geometry isn't just a math concept; it's a whole new way to see the world. From Einstein's theories to modern art, it pops up in the most unexpected places. Understanding it can change how you think about space, shapes, and even the universe.
Whether you're a student, a teacher, or just curious, diving into hyperbolic geometry can be a mind-bending adventure. It challenges what we know about Euclidean geometry and opens doors to new possibilities.
So next time you see a crocheted model or read about black holes, remember the role hyperbolic geometry plays. It's not just for mathematicians; it's for anyone who loves to explore and question the world around them. Keep your mind open, and who knows what you'll discover next?
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
