Bézout's Theorem is a fundamental concept in algebraic geometry and plays a crucial role in solving systems of polynomial equations. Understanding this theorem can provide valuable insights into the intersection of geometric objects and the number of solutions to polynomial equations. In this article, we will delve into 12 essential facts about Bézout's Theorem, shedding light on its significance and applications. From its historical origins to its practical implications in modern mathematics, each fact will unravel a unique aspect of this theorem, enriching your understanding of its relevance and impact. Whether you're a mathematics enthusiast, a student exploring algebraic concepts, or a curious mind seeking to grasp the intricacies of mathematical theorems, this exploration of Bézout's Theorem will offer valuable knowledge and insights. So, let's embark on a journey through the fascinating realm of Bézout's Theorem and uncover the 12 key facts that illuminate its importance and relevance in the world of mathematics.
Key Takeaways:
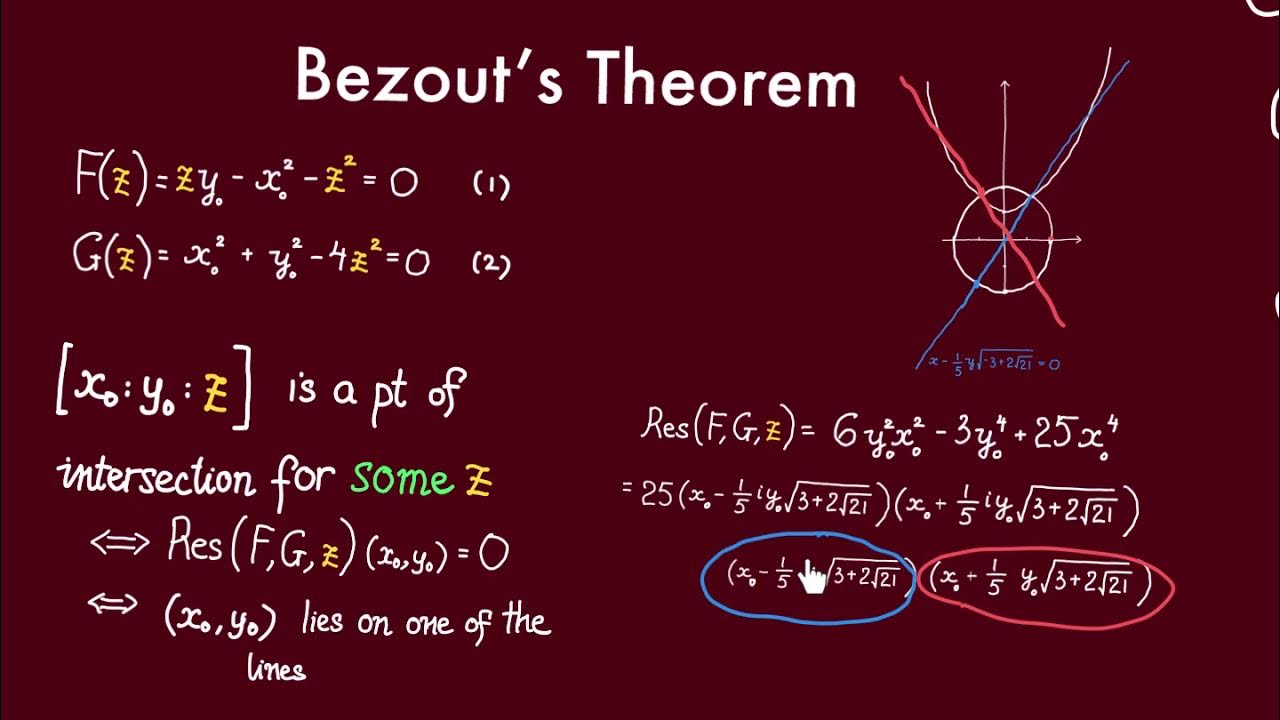
- Bézout’s Theorem helps us understand how many times two curves intersect, which is super important for designing cool stuff like robots and computer graphics!
- This theorem shows how algebra and geometry work together to solve real-world problems, inspiring new discoveries in math and technology.
Bézout's Theorem is a fundamental concept in algebraic geometry.
Bézout's Theorem, named after the French mathematician Étienne Bézout, is a fundamental concept in algebraic geometry. It states that the number of common points of intersection of two plane curves is equal to the product of their degrees, provided that the curves do not share any component. This theorem has far-reaching applications in various fields, including computer-aided design, robotics, and cryptography.
The theorem is based on the fundamental idea of algebraic geometry.
At its core, Bézout's Theorem is based on the fundamental idea of algebraic geometry, which deals with the study of solutions to polynomial equations. By applying algebraic techniques to geometric problems, mathematicians can gain deep insights into the properties of curves and surfaces, paving the way for advancements in diverse areas such as robotics, computer graphics, and cryptography.
Bézout's Theorem plays a crucial role in understanding the intersection of curves.
One of the key applications of Bézout's Theorem is in understanding the intersection of curves in a plane. By providing a precise method for determining the number of intersection points, this theorem forms the bedrock for solving complex geometric problems and has significant implications in fields such as computer-aided design and computational geometry.
The theorem has implications in computer-aided design and robotics.
In computer-aided design and robotics, Bézout's Theorem is instrumental in analyzing the intersections of curves and surfaces, enabling engineers and designers to create and manipulate complex shapes with precision and efficiency. This application underscores the practical significance of the theorem in modern technological advancements.
Bézout's Theorem is a cornerstone of modern cryptography.
In the realm of cryptography, Bézout's Theorem plays a pivotal role in the design and analysis of cryptographic algorithms. By providing a mathematical framework for understanding the intersections of curves, this theorem contributes to the development of secure and efficient cryptographic systems, safeguarding sensitive information in the digital age.
The theorem has historical significance in the development of algebraic geometry.
Bézout's Theorem holds historical significance in the development of algebraic geometry, a branch of mathematics that has deep connections to various other fields, including number theory, topology, and theoretical physics. The theorem's impact on the evolution of mathematical thought underscores its enduring importance in the realm of pure and applied mathematics.
Bézout's Theorem has connections to polynomial equations and their solutions.
At its essence, Bézout's Theorem is intimately connected to polynomial equations and their solutions. By providing a method for determining the number of common solutions to these equations, the theorem offers valuable insights into the geometric properties of curves and surfaces, laying the groundwork for advancements in diverse scientific and technological domains.
The theorem has practical applications in computational geometry.
In the realm of computational geometry, Bézout's Theorem finds practical applications in solving geometric problems related to the intersection of curves and surfaces. This computational aspect of the theorem underscores its relevance in modern scientific and engineering disciplines, where precise geometric analysis is essential for solving real-world challenges.
Bézout's Theorem is a key tool for analyzing geometric configurations.
As a key tool for analyzing geometric configurations, Bézout's Theorem provides mathematicians, engineers, and scientists with a powerful method for understanding the intersections of curves and surfaces. This analytical capability forms the basis for tackling complex geometric problems and has broad implications in fields ranging from computer graphics to theoretical physics.
The theorem has implications in theoretical physics and mathematical modeling.
In theoretical physics and mathematical modeling, Bézout's Theorem offers valuable insights into the geometric properties of physical phenomena and mathematical structures. By providing a rigorous framework for analyzing the intersections of curves and surfaces, the theorem contributes to the development of mathematical models that describe natural phenomena with precision and accuracy.
Bézout's Theorem is a testament to the profound interplay between algebra and geometry.
Bézout's Theorem stands as a testament to the profound interplay between algebra and geometry, showcasing how algebraic techniques can illuminate geometric phenomena and vice versa. This deep connection between two seemingly distinct branches of mathematics underscores the unity of mathematical thought and the richness of interdisciplinary exploration.
The theorem continues to inspire new avenues of mathematical research and innovation.
With its far-reaching implications and foundational significance, Bézout's Theorem continues to inspire new avenues of mathematical research and innovation. Its enduring legacy in algebraic geometry and its applications in diverse fields underscore the timeless relevance of this fundamental theorem in the ever-evolving landscape of mathematics and its intersections with science and technology.
Conclusion
In conclusion, Bézout's Theorem is a fundamental concept in algebraic geometry that provides a powerful tool for understanding the intersection of geometric objects. Its applications extend across various fields, including computer graphics, robotics, and cryptography. By grasping the 12 essential facts about Bézout's Theorem, one can gain a deeper appreciation for its significance and practical implications. From its historical origins to its modern-day relevance, this theorem continues to inspire mathematicians and scientists to explore the intricate connections between algebraic equations and geometric shapes.
FAQs
What is the significance of Bézout's Theorem?Bézout's Theorem plays a crucial role in algebraic geometry by providing a method to determine the number of intersection points between algebraic curves. This has wide-ranging applications in fields such as computer-aided design, robotics, and cryptography.
How does Bézout's Theorem relate to real-world applications?Bézout's Theorem has practical implications in various real-world scenarios, including computer graphics, where it is used to calculate the intersections of curves and surfaces, and in robotics, where it aids in path planning and collision detection.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
