L'Hôpital's Rule is a powerful tool in calculus that helps us evaluate limits involving indeterminate forms. Understanding this rule is crucial for tackling complex mathematical problems and gaining a deeper insight into the behavior of functions. In this article, we will explore 10 essential facts about L'Hôpital's Rule, shedding light on its origins, applications, and significance in calculus. Whether you're a student delving into the intricacies of calculus or a math enthusiast seeking to expand your knowledge, these facts will provide valuable insights into this fundamental concept. Let's embark on a journey to unravel the mysteries of L'Hôpital's Rule and discover its profound impact on the world of mathematics.
Key Takeaways:
- L’Hôpital’s Rule is like a secret weapon for solving tricky calculus problems involving limits, making math feel like a cool adventure rather than a daunting challenge.
- Understanding L’Hôpital’s Rule not only helps solve tough math problems but also opens doors to mastering more advanced mathematical concepts with confidence and skill.
L'Hôpital's Rule is a powerful tool in calculus.
L'Hôpital's Rule is a fundamental concept in calculus that allows us to evaluate indeterminate forms involving limits. It's like having a secret weapon in your mathematical arsenal, ready to tackle complex problems with finesse and precision.
The rule is named after the French mathematician Guillaume de l'Hôpital.
Guillaume de l'Hôpital was not only a mathematician but also a nobleman. He gained fame for his patronage of the renowned mathematician Johann Bernoulli and for his role in publishing Bernoulli's work. The rule bearing his name is a testament to his lasting impact on the field of mathematics.
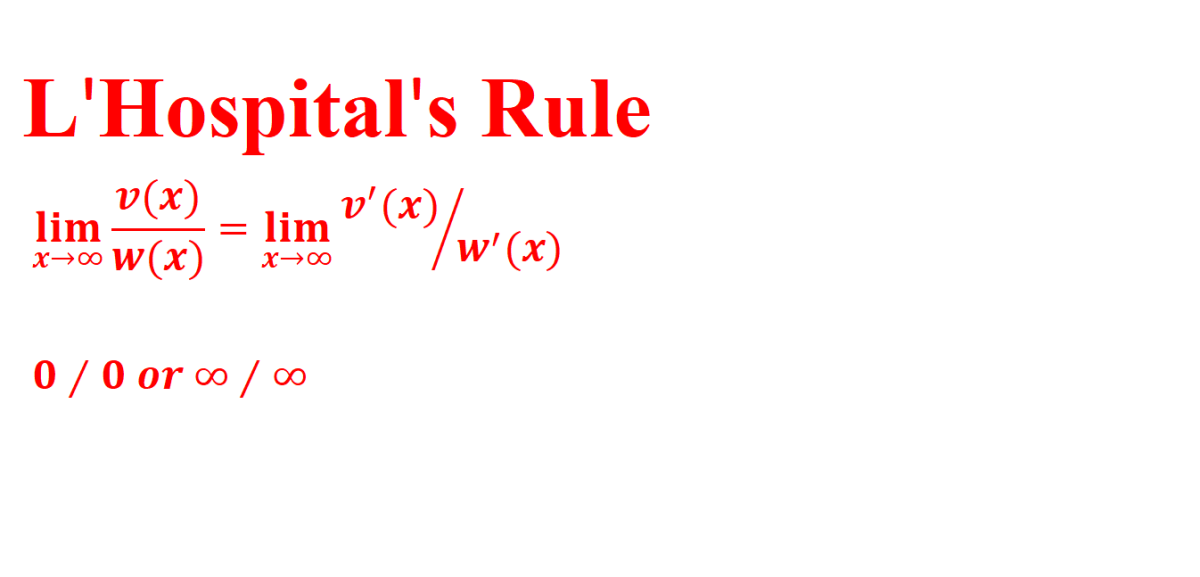
L'Hôpital's Rule is particularly useful when dealing with limits involving fractions.
When faced with a limit that results in an indeterminate form such as 0/0 or ?/?, L'Hôpital's Rule provides an elegant method to resolve these uncertainties and determine the true value of the limit.
It can be applied to various indeterminate forms.
L'Hôpital's Rule is not limited to just fractions; it can also be applied to other indeterminate forms such as 0*?, ?-?, and 0^This versatility makes it an indispensable tool for solving a wide range of limit problems.
The rule is based on derivatives.
At its core, L'Hôpital's Rule leverages the power of derivatives to simplify complex limits. By taking the derivatives of the numerator and denominator separately, we can often transform an indeterminate form into a determinate one, allowing us to find the limit with ease.
L'Hôpital's Rule is a go-to technique for solving challenging calculus problems.
When traditional methods for evaluating limits fall short, L'Hôpital's Rule steps in as a reliable strategy for resolving indeterminate forms and determining precise limit values. Its versatility and effectiveness make it a valuable asset for calculus students and mathematicians alike.
The rule provides a systematic approach to resolving uncertainties in limits.
By following a set of clear steps outlined in L'Hôpital's Rule, mathematicians can methodically address indeterminate forms and arrive at accurate limit evaluations. This systematic approach adds a layer of clarity to limit problem-solving.
It is often introduced in advanced calculus courses.
L'Hôpital's Rule typically makes its grand entrance in advanced calculus courses, where students delve into the intricacies of limits, derivatives, and their applications. Its introduction marks a pivotal moment in a calculus student's journey.
The rule has historical significance in the development of calculus.
L'Hôpital's Rule stands as a testament to the rich history of calculus, showcasing the innovative techniques and insights that have shaped the field over the centuries. Its historical significance underscores its enduring relevance in mathematical education and practice.
Understanding L'Hôpital's Rule opens doors to mastering complex mathematical concepts.
By grasping the intricacies of L'Hôpital's Rule, students gain a deeper understanding of limit theory, derivatives, and their interconnectedness. This knowledge serves as a springboard for tackling more advanced mathematical concepts with confidence and proficiency.
Conclusion
In conclusion, L'Hôpital's Rule is a powerful tool in calculus for evaluating indeterminate forms. Understanding its application and limitations is crucial for mastering advanced calculus and solving complex mathematical problems. By leveraging the insights gained from the 10 facts presented in this article, mathematicians and students alike can confidently navigate through challenging limits and derivatives. L'Hôpital's Rule stands as a testament to the ingenuity and depth of mathematical principles, offering a systematic approach to unraveling intricate mathematical conundrums.
FAQs
What are some common indeterminate forms that L'Hôpital's Rule can be applied to?L'Hôpital's Rule is commonly used to evaluate indeterminate forms such as 0/0, ?/?, 0*?, 0^0, ?^0, and 1^?.
Can L'Hôpital's Rule be applied to functions with multiple variables?No, L'Hôpital's Rule is specifically designed for functions with a single variable. When dealing with multivariable functions, alternative methods such as partial derivatives and limits are employed to evaluate indeterminate forms.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
