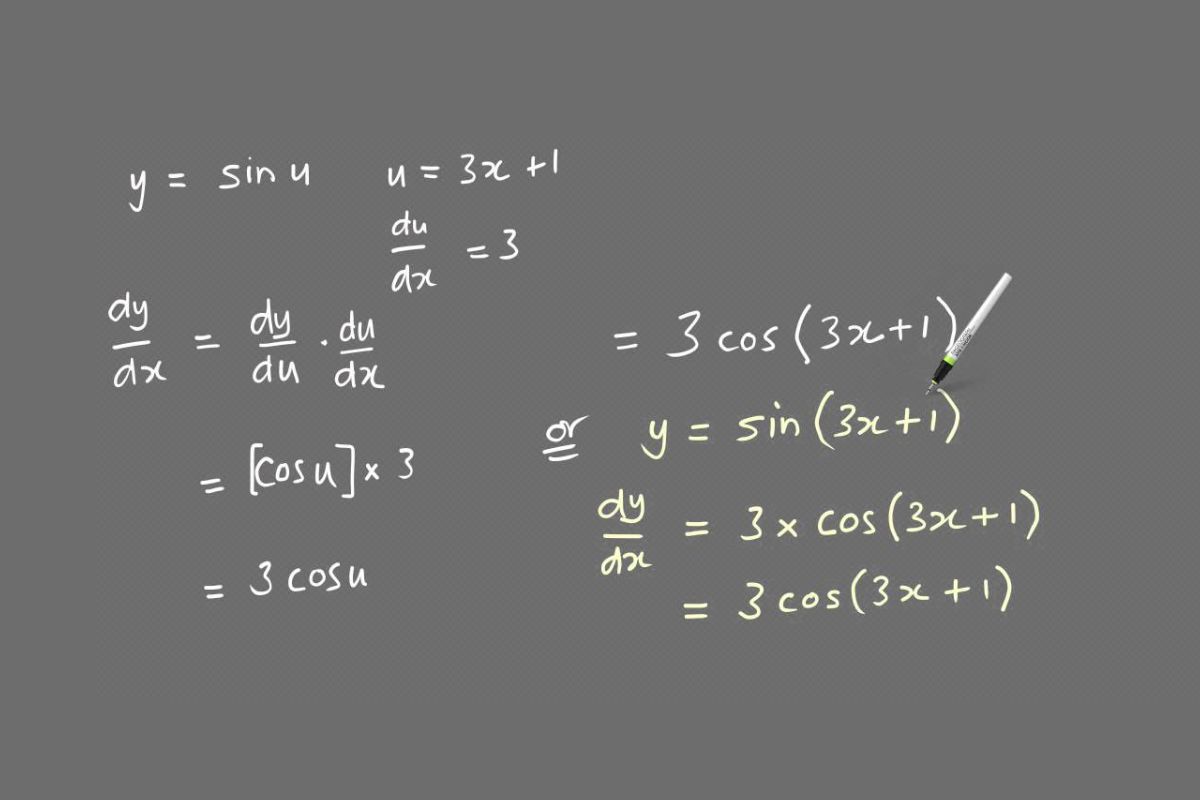
Calculus is a branch of mathematics that studies how things change. It’s all about understanding rates of change and accumulation. Isaac Newton and Gottfried Wilhelm Leibniz are credited with its development in the late 17th century. Calculus is divided into two main parts: differential calculus and integral calculus. Differential calculus focuses on finding the rate at which quantities change, while integral calculus deals with the accumulation of quantities. These methods have countless applications in science, engineering, economics, and even medicine. Ever wondered how your GPS calculates the fastest route? Or how engineers design roller coasters? Calculus is the answer. From predicting planetary movements to optimizing business profits, calculus methods are everywhere. Ready to dive into 28 fascinating facts about calculus methods? Let's get started!
What is Calculus?
Calculus is a branch of mathematics that studies how things change. It provides tools to model and understand dynamic systems. Let's dive into some fascinating facts about calculus methods.
-
Calculus was independently developed by Isaac Newton and Gottfried Wilhelm Leibniz in the late 17th century. Both mathematicians are credited with its creation.
-
The term "calculus" comes from the Latin word for "small stone," used for counting and calculations in ancient times.
-
Calculus is divided into two main branches: differential calculus and integral calculus. Differential calculus focuses on rates of change, while integral calculus deals with accumulation of quantities.
Differential Calculus
Differential calculus is all about understanding how things change. It helps in finding the rate at which a quantity changes.
-
The derivative is a fundamental concept in differential calculus. It measures how a function's output changes as its input changes.
-
Newton's notation for derivatives uses a dot above the variable (e.g., (dot{y})), while Leibniz's notation uses (dy/dx).
-
The power rule is a basic differentiation rule. It states that the derivative of (x^n) is (nx^{n-1}).
-
The chain rule allows us to differentiate composite functions. If (y = f(g(x))), then (dy/dx = f'(g(x)) cdot g'(x)).
-
The product rule is used to differentiate products of two functions. If (u) and (v) are functions of (x), then ((uv)' = u'v + uv').
-
The quotient rule helps differentiate ratios of two functions. If (u) and (v) are functions of (x), then ((u/v)' = (u'v – uv')/v^2).
Integral Calculus
Integral calculus deals with the accumulation of quantities. It helps in finding areas under curves and total accumulated change.
-
The integral is the inverse operation of the derivative. It accumulates the quantity over an interval.
-
The definite integral calculates the net area under a curve between two points. It is denoted by (int_a^b f(x) , dx).
-
The indefinite integral represents a family of functions and includes an arbitrary constant (C). It is denoted by (int f(x) , dx).
-
The Fundamental Theorem of Calculus links differentiation and integration. It states that differentiation and integration are inverse processes.
-
The substitution rule simplifies integration by changing variables. If (u = g(x)), then (int f(g(x))g'(x) , dx = int f(u) , du).
-
Integration by parts is a technique derived from the product rule. It is used to integrate products of functions. If (u) and (v) are functions of (x), then (int u , dv = uv – int v , du).
-
The area under a curve can be found using definite integrals. This is useful in physics for calculating work done by a force.
Applications of Calculus
Calculus isn't just theoretical; it has practical applications in various fields.
-
In physics, calculus helps describe motion, electricity, heat, light, harmonics, acoustics, astronomy, and even quantum mechanics.
-
Engineers use calculus to design and analyze systems and structures, ensuring safety and efficiency.
-
In economics, calculus helps in finding maximum profit and minimum cost by analyzing marginal functions.
-
Biologists use calculus to model population dynamics and the spread of diseases.
-
In computer science, algorithms for machine learning and data analysis often rely on calculus.
-
Calculus is essential in medicine for modeling the growth of tumors and the spread of diseases.
Advanced Calculus Concepts
Beyond the basics, calculus has more complex and intriguing concepts.
-
Multivariable calculus extends the principles of calculus to functions of several variables. It includes partial derivatives and multiple integrals.
-
Vector calculus deals with vector fields and includes operations like divergence and curl.
-
Stokes' Theorem and Green's Theorem are fundamental theorems in vector calculus, relating surface integrals to line integrals.
-
Differential equations involve functions and their derivatives. They are used to model real-world systems.
-
The Laplace transform is a technique to transform differential equations into algebraic equations, making them easier to solve.
-
Fourier series and transforms break down functions into sums of sine and cosine terms, useful in signal processing and heat transfer.
The Final Word on Calculus Methods
Calculus methods have shaped our understanding of the world in countless ways. From differentiation to integration, these techniques help solve complex problems in physics, engineering, and economics. Newton and Leibniz laid the groundwork, but modern advancements continue to push boundaries. Limits and derivatives are fundamental, while integrals offer a way to calculate areas and volumes. Chain rule and product rule simplify differentiation of complex functions. Partial derivatives and multiple integrals extend these ideas to higher dimensions. Understanding these concepts not only boosts problem-solving skills but also opens doors to various scientific fields. Calculus isn't just for mathematicians; it's a tool for anyone curious about how things change and interact. So, whether you're a student, a professional, or just a curious mind, mastering calculus methods can be a game-changer. Dive in, explore, and let calculus reveal the hidden patterns of the universe.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
