26 Facts About Maxwell’s Equations

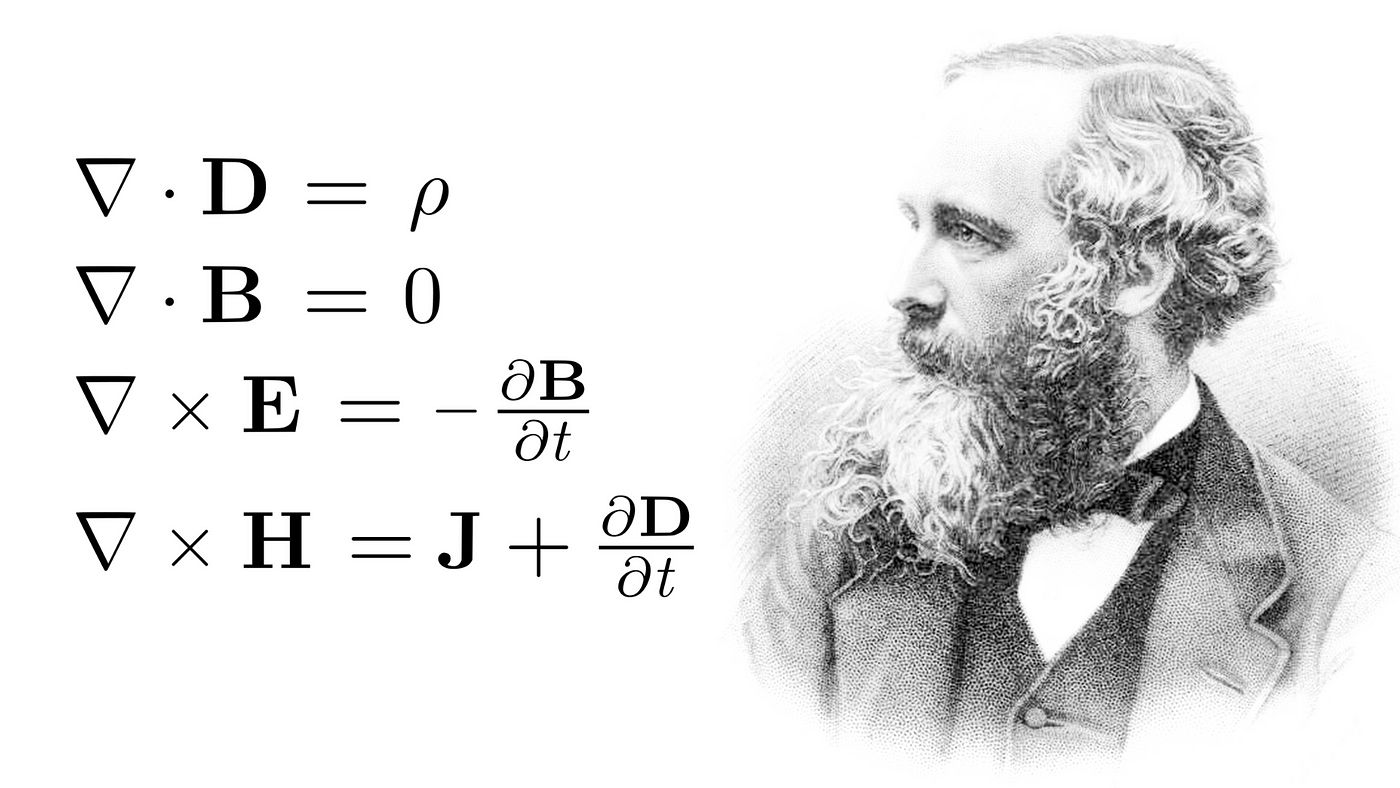
Maxwell’s Equations: A Brief Overview

Maxwell’s Equations form the foundation of classical electromagnetism. These four equations describe how electric and magnetic fields interact and propagate. Let’s dive into some fascinating facts about these pivotal equations.

1. James Clerk Maxwell formulated these equations in the 1860s. Maxwell, a Scottish physicist, unified the theories of electricity and magnetism into a single framework.
2. They consist of four equations. These are Gauss’s law for electricity, Gauss’s law for magnetism, Faraday’s law of induction, and Ampère’s law with Maxwell’s correction.
3. Maxwell’s Equations predict electromagnetic waves. They show that light is an electromagnetic wave, traveling at the speed of light.
4. They are partial differential equations. This means they involve rates of change with respect to continuous variables.
5. Maxwell’s Equations are linear. Solutions can be added together to form new solutions, making them easier to solve.

Gauss’s Law for Electricity

Gauss’s law for electricity describes how electric charges produce electric fields. It is one of the cornerstones of electromagnetism.

6. Gauss’s law states that the electric flux through a closed surface is proportional to the charge enclosed. This means the total electric field passing through a surface depends on the charge inside.
7. It is mathematically expressed as ∇·E = ρ/ε₀. Here, E is the electric field, ρ is the charge density, and ε₀ is the permittivity of free space.
8. Gauss’s law can be derived from Coulomb’s law. Coulomb’s law describes the force between two point charges, and Gauss’s law generalizes this to any charge distribution.

Gauss’s Law for Magnetism

Gauss’s law for magnetism deals with magnetic fields and their sources. Unlike electric fields, magnetic fields do not have isolated sources.

9. Gauss’s law for magnetism states that the net magnetic flux through a closed surface is zero. This implies there are no magnetic monopoles.
10. It is mathematically expressed as ∇·B = 0. Here, B is the magnetic field.
11. This law reflects the fact that magnetic field lines are continuous loops. They do not start or end at any point.

Faraday’s Law of Induction

Faraday’s law of induction explains how changing magnetic fields can induce electric fields. This principle is crucial for many electrical technologies.

12. Faraday’s law states that a changing magnetic field creates an electric field. This is the principle behind electric generators.
13. It is mathematically expressed as ∇×E = -∂B/∂t. Here, E is the electric field, B is the magnetic field, and t is time.
14. Faraday’s law is the basis for transformers and inductors. These devices rely on changing magnetic fields to function.

Ampère’s Law with Maxwell’s Correction

Ampère’s law originally described how electric currents produce magnetic fields. Maxwell added a crucial correction to account for changing electric fields.

15. Ampère’s law with Maxwell’s correction states that magnetic fields are generated by electric currents and changing electric fields. This unifies the behavior of electric and magnetic fields.
16. It is mathematically expressed as ∇×B = μ₀(J + ε₀∂E/∂t). Here, B is the magnetic field, J is the current density, E is the electric field, μ₀ is the permeability of free space, and t is time.
17. Maxwell’s correction term accounts for displacement current. This term is essential for the consistency of the equations.

Impact on Modern Technology

Maxwell’s Equations have far-reaching implications in various fields of science and technology. They are fundamental to understanding and developing many modern devices.

18. They are essential for the design of electrical circuits. Understanding how electric and magnetic fields interact helps in creating efficient circuits.
19. Maxwell’s Equations are crucial for telecommunications. They describe how electromagnetic waves propagate, which is vital for radio, TV, and mobile communications.
20. They underpin the operation of MRI machines. Magnetic Resonance Imaging relies on principles derived from these equations.
21. Maxwell’s Equations are used in antenna design. Engineers use them to optimize the transmission and reception of signals.

Theoretical Implications

Beyond practical applications, Maxwell’s Equations have profound theoretical implications. They have influenced many areas of physics.

22. They led to the development of special relativity. Einstein’s theory was partly inspired by the need to reconcile Maxwell’s Equations with the laws of mechanics.
23. Maxwell’s Equations are consistent with quantum mechanics. They can be extended to describe the behavior of electromagnetic fields at the quantum level.
24. They are a cornerstone of classical field theory. This framework describes how fields interact with matter.
25. Maxwell’s Equations are used in computational electromagnetics. Simulating electromagnetic fields requires solving these equations numerically.
26. They have inspired further unification in physics. Just as Maxwell unified electricity and magnetism, physicists seek to unify all fundamental forces.

The Legacy of Maxwell’s Equations

Maxwell’s Equations have stood the test of time, shaping modern physics and engineering. These four elegant formulas describe how electric and magnetic fields interact, laying the groundwork for technologies like radio, television, and even smartphones. Without them, our understanding of electromagnetism would be incomplete, and many of the conveniences we take for granted wouldn’t exist.

From guiding the development of wireless communication to influencing the theory of relativity, Maxwell’s Equations are more than just mathematical expressions. They’re a testament to human curiosity and ingenuity. As we continue to explore the universe, these equations will remain a cornerstone of scientific discovery, reminding us of the power of theoretical insight combined with practical application.

So next time you use your phone or watch TV, remember the genius of James Clerk Maxwell and his groundbreaking work that made it all possible.