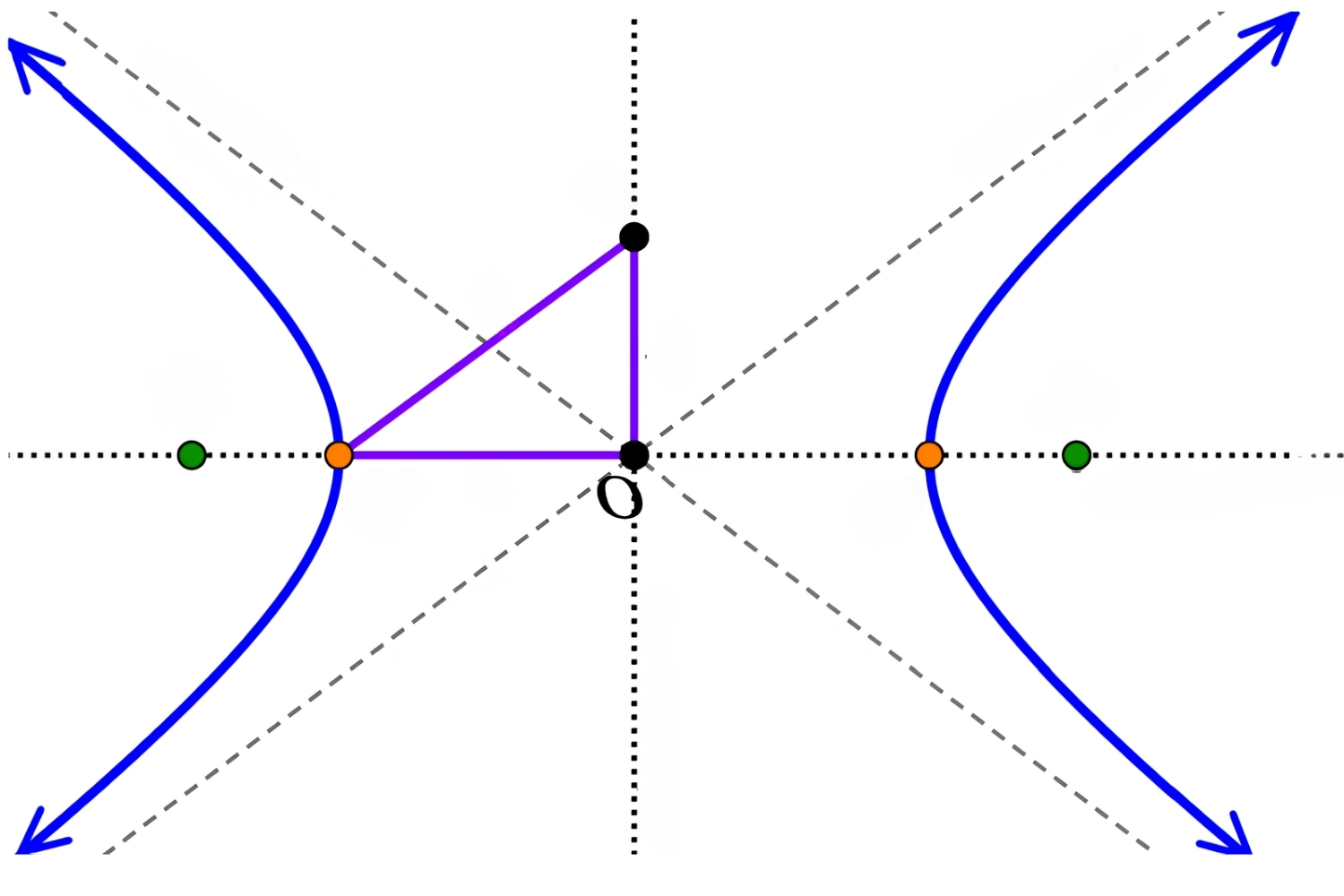
What is an asymptote? An asymptote is a line that a curve approaches but never quite touches. Imagine a race where you get closer and closer to the finish line but never actually cross it. That's how an asymptote works in math. These lines can be horizontal, vertical, or even slanted. They help us understand the behavior of graphs, especially when dealing with infinity. Knowing about asymptotes can make complex math problems easier to solve. Whether you're graphing functions or studying calculus, understanding asymptotes is crucial. Ready to dive into some cool facts about asymptotes? Let's get started!
What is an Asymptote?
An asymptote is a line that a curve approaches but never actually touches. This concept is fundamental in mathematics, especially in calculus and algebra. Let's dive into some intriguing facts about asymptotes.
-
The term "asymptote" comes from the Greek word "asymptotos," which means "not falling together."
-
Asymptotes can be vertical, horizontal, or oblique (slanted).
-
Vertical asymptotes occur when the function approaches infinity as the input approaches a specific value.
-
Horizontal asymptotes happen when the function approaches a constant value as the input goes to infinity.
-
Oblique asymptotes occur when the function approaches a line that isn't horizontal or vertical as the input goes to infinity.
Historical Background of Asymptotes
Understanding the history behind asymptotes can provide a deeper appreciation for their significance in mathematics.
-
The concept of asymptotes dates back to ancient Greek mathematicians like Apollonius of Perga.
-
Apollonius studied conic sections and identified asymptotes in hyperbolas.
-
René Descartes, a French philosopher and mathematician, further developed the concept in the 17th century.
-
Descartes introduced the idea of coordinate geometry, which made it easier to visualize asymptotes.
-
Isaac Newton and Gottfried Wilhelm Leibniz, the founders of calculus, used asymptotes in their work on limits and infinite series.
Types of Asymptotes
Different types of asymptotes serve various purposes in mathematical analysis.
-
Vertical asymptotes are often found in rational functions where the denominator equals zero.
-
Horizontal asymptotes are common in exponential and logarithmic functions.
-
Oblique asymptotes usually appear in rational functions where the degree of the numerator is one more than the degree of the denominator.
-
Functions can have more than one type of asymptote.
-
Some functions, like trigonometric functions, have asymptotes that repeat periodically.
Asymptotes in Real Life
Asymptotes aren't just theoretical; they have practical applications in various fields.
-
In physics, asymptotes describe the behavior of objects approaching the speed of light.
-
Asymptotes are used in economics to model diminishing returns.
-
In engineering, asymptotes help in understanding the behavior of control systems.
-
Asymptotes are used in biology to model population growth and carrying capacity.
-
In computer science, asymptotes describe the efficiency of algorithms.
Graphing Asymptotes
Graphing asymptotes helps visualize the behavior of functions.
-
To find vertical asymptotes, set the denominator of a rational function to zero and solve for the variable.
-
Horizontal asymptotes can be found by taking the limit of the function as the variable approaches infinity.
-
Oblique asymptotes are found by performing polynomial long division on the function.
-
Graphing software and calculators often have built-in tools for identifying asymptotes.
-
Understanding asymptotes can help in sketching accurate graphs of complex functions.
Fun Facts about Asymptotes
Let's explore some lesser-known, fun facts about asymptotes.
-
The hyperbola is the only conic section with asymptotes.
-
Asymptotes can be used to approximate complex functions with simpler ones.
-
In some cases, asymptotes can intersect the curve they are approximating.
-
The concept of asymptotes extends to higher dimensions, such as asymptotic planes in three-dimensional space.
-
Asymptotes play a crucial role in the study of fractals and chaos theory.
Misconceptions about Asymptotes
There are several common misconceptions about asymptotes that need clarification.
-
An asymptote is not a boundary that a function cannot cross.
-
Functions can cross their horizontal or oblique asymptotes.
-
Not all functions have asymptotes.
-
Asymptotes do not always indicate a function's behavior at infinity.
-
The presence of an asymptote does not imply that a function is undefined at that point.
Advanced Concepts Related to Asymptotes
For those interested in diving deeper, here are some advanced concepts related to asymptotes.
-
Asymptotic analysis is used in mathematics to describe the behavior of functions as inputs become large.
-
Asymptotic expansions provide approximations of functions using series.
-
In number theory, asymptotic density measures the distribution of prime numbers.
-
Asymptotic stability in differential equations describes the long-term behavior of solutions.
The Final Stretch
Asymptotes might seem tricky at first, but they're just lines that curves get close to without touching. They help us understand the behavior of graphs, especially in calculus. Horizontal asymptotes show us where a function heads as x goes to infinity, while vertical ones tell us where the function shoots up or down. Oblique asymptotes? They’re the slanted lines that come into play when functions grow without bound.
Knowing about asymptotes can make graphing functions way easier. They give us a sneak peek into the function's behavior at extreme values. Plus, they’re super useful in real-world applications like engineering and physics.
So next time you see a complex graph, remember, asymptotes are your friends. They guide you through the chaos, making sense of the seemingly unpredictable. Keep these facts in mind, and you'll master those tricky curves in no time!
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
