Logarithms might sound like a complicated math term, but they play a huge role in our everyday lives. From calculating interest rates to measuring the intensity of earthquakes, logarithms are everywhere. But what exactly are logarithms? In simple terms, they are the opposite of exponents. If you know that 2^3 equals 8, then the logarithm tells you that the log base 2 of 8 is 3. This concept helps us solve problems involving exponential growth or decay. Whether you're a student trying to ace your math test or just curious about the world, understanding logarithms can be incredibly useful. Ready to learn some cool facts about logarithms? Let's dive in!
What is a Logarithm?
Logarithms are a fundamental concept in mathematics, often used to solve equations involving exponential growth or decay. They help simplify complex calculations by transforming multiplicative relationships into additive ones.
-
Definition: A logarithm is the power to which a number must be raised to obtain another number. For example, in the equation (10^2 = 100), the logarithm of 100 with base 10 is 2.
-
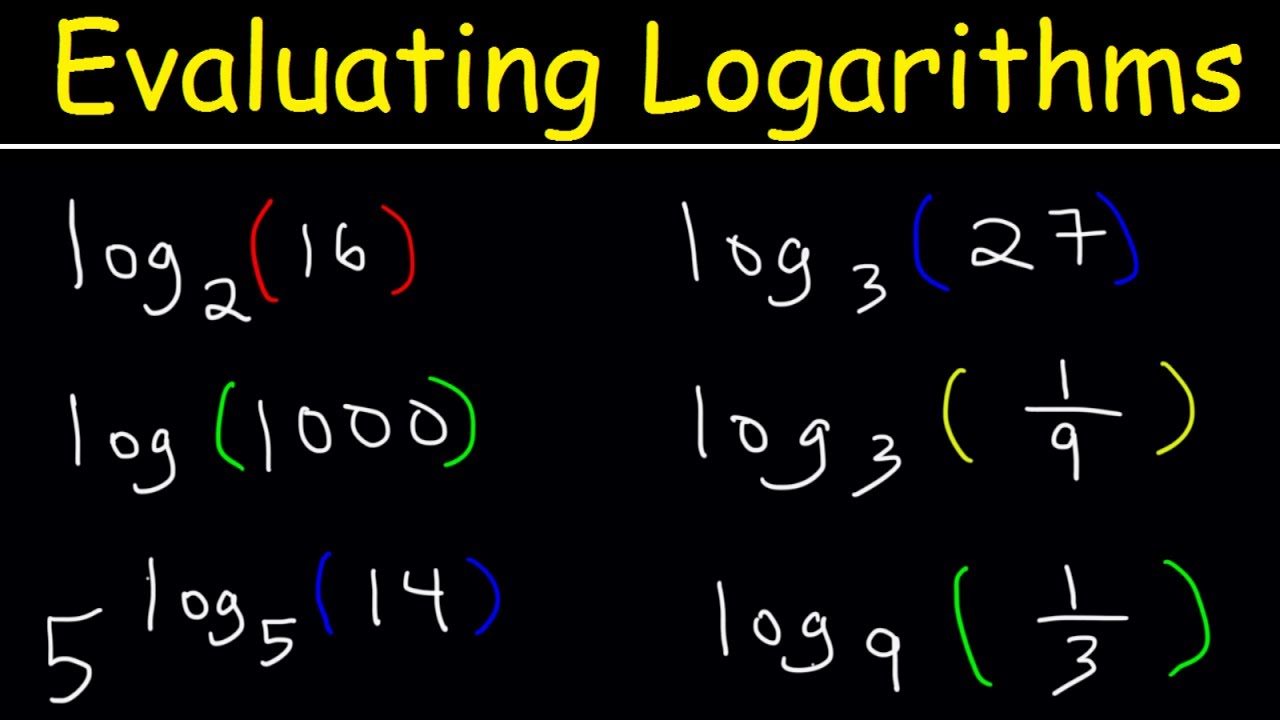
Notation: The logarithm of a number (x) with base (b) is written as (log_b(x)). For instance, (log_2(8) = 3) because (2^3 = 8).
-
Inverse Function: Logarithms are the inverse functions of exponentials. If (b^y = x), then (log_b(x) = y).
-
Common Logarithms: Logarithms with base 10 are called common logarithms and are often written as (log(x)) without the base.
-
Natural Logarithms: Logarithms with base (e) (approximately 2.718) are called natural logarithms and are denoted as (ln(x)).
Historical Background
Logarithms have a rich history, dating back several centuries. They were developed to simplify complex calculations, especially in astronomy and navigation.
-
John Napier: The Scottish mathematician John Napier introduced logarithms in 1614 to simplify multiplication and division.
-
Henry Briggs: Henry Briggs, an English mathematician, collaborated with Napier and introduced the common logarithm.
-
Slide Rule: Before calculators, slide rules, which use logarithmic scales, were essential tools for engineers and scientists.
-
Logarithm Tables: Logarithm tables were widely used before electronic calculators, providing precomputed values of logarithms for quick reference.
-
Euler's Contribution: The Swiss mathematician Leonhard Euler popularized the natural logarithm and the constant (e).
Properties of Logarithms
Logarithms have several key properties that make them useful in various mathematical applications.
-
Product Rule: (log_b(xy) = log_b(x) + log_b(y)). This property transforms multiplication into addition.
-
Quotient Rule: (log_bleft(frac{x}{y}right) = log_b(x) – log_b(y)). This property transforms division into subtraction.
-
Power Rule: (log_b(x^y) = y cdot log_b(x)). This property helps in dealing with exponents.
-
Change of Base Formula: (log_b(x) = frac{log_k(x)}{log_k(b)}). This formula allows changing the base of a logarithm.
-
Logarithm of 1: (log_b(1) = 0) for any base (b), because any number raised to the power of 0 is 1.
-
Logarithm of the Base: (log_b(b) = 1) because any number raised to the power of 1 is itself.
Applications of Logarithms
Logarithms are not just theoretical; they have practical applications in various fields.
-
Earthquakes: The Richter scale, used to measure earthquake magnitude, is logarithmic.
-
Sound Intensity: Decibels, a unit for measuring sound intensity, use a logarithmic scale.
-
pH Levels: The pH scale, which measures acidity or alkalinity, is logarithmic.
-
Finance: Logarithms are used in calculating compound interest and in financial modeling.
-
Computer Science: Algorithms, especially those involving data structures like binary trees, often use logarithms.
-
Population Growth: Logarithms help model exponential growth in populations.
Logarithms in Technology
Modern technology relies heavily on logarithms for various functions and processes.
-
Compression Algorithms: Logarithms are used in data compression algorithms to reduce file sizes.
-
Signal Processing: Logarithms help in analyzing and processing signals in telecommunications.
-
Machine Learning: Logarithmic functions are used in algorithms for training machine learning models.
-
Cryptography: Logarithms play a crucial role in encryption algorithms, ensuring data security.
Fun Facts about Logarithms
Logarithms can be fun and interesting, with some quirky facts that might surprise you.
-
Logarithmic Spiral: Many natural phenomena, like the shape of galaxies and seashells, follow a logarithmic spiral.
-
Benford's Law: In many real-life datasets, the leading digit is more likely to be small, a phenomenon explained by logarithms.
-
Music: The frequencies of musical notes are logarithmically spaced.
-
Human Perception: Humans perceive sound and light intensity logarithmically, not linearly.
-
Fractals: Logarithms are used in generating fractals, which are complex, self-similar patterns.
Challenges with Logarithms
Despite their usefulness, logarithms can be challenging to understand and apply.
-
Complex Numbers: Logarithms can be extended to complex numbers, but this requires advanced mathematical understanding.
-
Negative Numbers: Logarithms of negative numbers are not defined in the real number system.
-
Base Restrictions: The base of a logarithm must be positive and not equal to 1.
-
Approximation: Calculating logarithms by hand often involves approximation, which can introduce errors.
-
Graphing: Graphing logarithmic functions requires understanding their unique properties and behavior.
-
Educational Barriers: Many students find logarithms difficult to grasp, requiring effective teaching methods to overcome this challenge.
The Final Countdown
Logarithms might seem tricky at first, but they’re super useful once you get the hang of them. From simplifying complex calculations to helping us understand exponential growth, they play a big role in math and science. They’re like the secret sauce in everything from computer algorithms to measuring the intensity of earthquakes. Knowing how to work with logarithms can give you a leg up in many fields, whether you’re into engineering, finance, or even music theory. So next time you see a logarithm, don’t freak out. Remember, they’re just another tool in your math toolbox, ready to help you tackle problems with ease. Keep practicing, stay curious, and you’ll find that logarithms aren’t just numbers—they’re keys to unlocking a deeper understanding of the world around us.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
