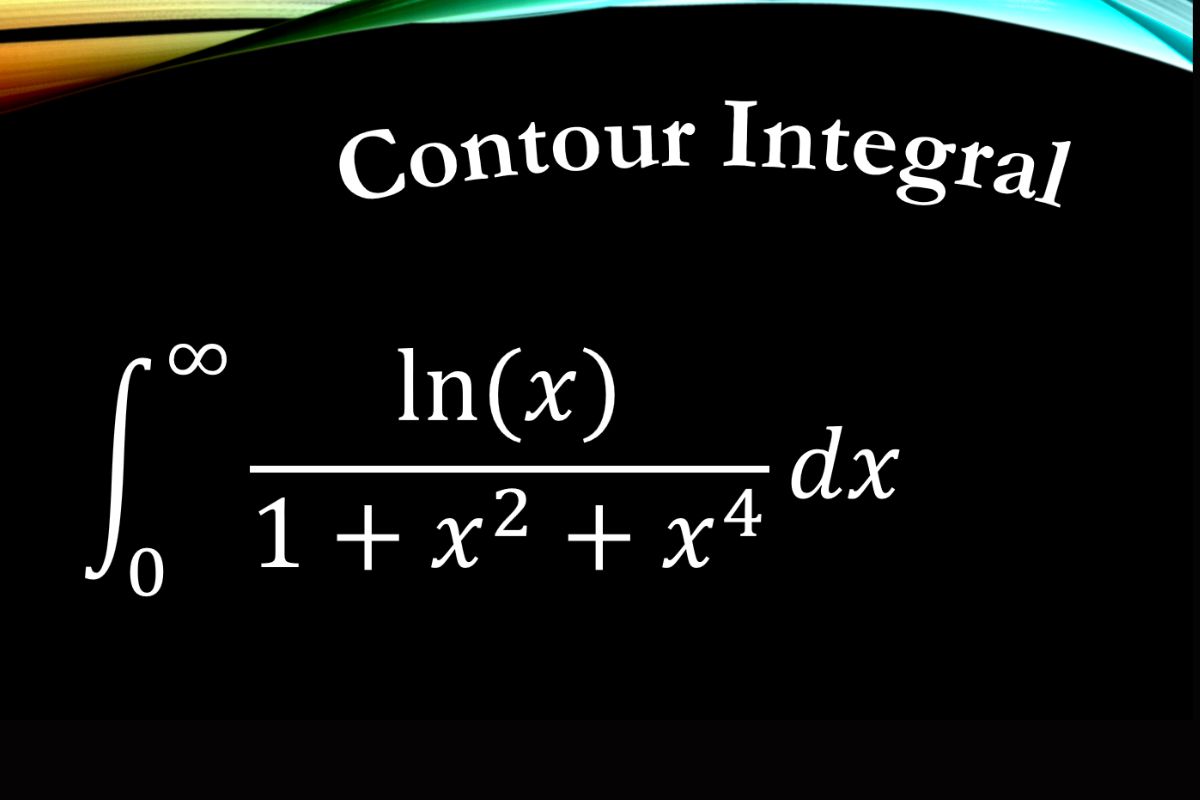
Integral analysis might sound like a complex topic, but it's actually a fundamental part of calculus that helps us understand the world around us. Ever wondered how we calculate areas under curves, volumes of odd shapes, or even the total distance traveled by a car? That's where integral analysis comes in. It’s all about summing up tiny pieces to get a big picture. Whether you're a student trying to grasp the basics or someone curious about how math applies to real life, these 31 facts will make integral analysis clearer and more interesting. Ready to dive into the world of integrals? Let's get started!
What is Integral Analysis?
Integral analysis is a branch of mathematics focusing on integrals and their properties. It plays a crucial role in various fields like physics, engineering, and economics. Here are some intriguing facts about integral analysis:
-
Integral analysis helps calculate areas under curves, which is essential for determining distances, volumes, and other physical properties.
-
The concept of integration dates back to ancient Greece, where mathematicians like Archimedes used it to find areas and volumes.
-
The fundamental theorem of calculus links differentiation and integration, showing they are inverse processes.
Types of Integrals
Integral analysis involves different types of integrals, each serving unique purposes. Let's explore some of them:
-
Definite integrals calculate the area under a curve between two specific points.
-
Indefinite integrals represent a family of functions and include an arbitrary constant, often denoted as 'C'.
-
Improper integrals extend the concept of definite integrals to unbounded intervals or functions with infinite discontinuities.
Applications in Physics
Integral analysis is indispensable in physics, helping to solve complex problems. Here are some examples:
-
In mechanics, integrals determine the center of mass and moments of inertia for objects.
-
Electromagnetic theory uses integrals to calculate electric and magnetic fields.
-
Quantum mechanics relies on integrals to find probabilities and expectation values of physical quantities.
Applications in Engineering
Engineers use integral analysis to design and optimize systems. Some key applications include:
-
In civil engineering, integrals help calculate loads and stresses on structures like bridges and buildings.
-
Electrical engineers use integrals to analyze circuits and signal processing.
-
In fluid mechanics, integrals determine flow rates and pressure distributions.
Historical Milestones
Integral analysis has a rich history with significant milestones. Here are a few:
-
Isaac Newton and Gottfried Wilhelm Leibniz independently developed the fundamental theorem of calculus in the late 17th century.
-
Bernhard Riemann introduced the concept of Riemann integrals in the 19th century, providing a rigorous foundation for integration.
-
Henri Lebesgue developed Lebesgue integration in the early 20th century, extending the scope of integrable functions.
Advanced Topics
Integral analysis continues to evolve, with advanced topics pushing the boundaries of mathematics. Some notable areas include:
-
Multiple integrals extend the concept of integration to functions of several variables, useful in higher-dimensional problems.
-
Line integrals and surface integrals generalize integration to curves and surfaces, essential in vector calculus.
-
The Laplace transform and Fourier transform use integrals to convert functions between time and frequency domains, aiding in signal analysis.
Real-World Examples
Integral analysis isn't just theoretical; it has practical applications in everyday life. Here are some examples:
-
Economists use integrals to model and predict economic behavior, such as consumer demand and market trends.
-
In medicine, integrals help analyze biological data, like blood flow rates and drug concentration over time.
-
Environmental scientists use integrals to study pollution dispersion and resource management.
Fun Facts
Integral analysis has some quirky and fun aspects. Check these out:
-
The symbol for integration, ∫, was introduced by Leibniz and is derived from the Latin word "summa."
-
The integral of e^x is one of the few functions that remains unchanged after integration.
-
Integrals can be visualized using various techniques, like slicing, summing, and rotating shapes.
Challenges and Puzzles
Integral analysis can be challenging but also rewarding. Here are some interesting puzzles:
-
The Basel problem, solved by Euler, involves finding the exact sum of the reciprocals of the squares of natural numbers.
-
The Dirichlet integral, which evaluates to π, is a famous example of an improper integral.
-
The Gaussian integral, used in probability theory, evaluates to the square root of π.
Integral Analysis in Technology
Modern technology heavily relies on integral analysis. Here are some examples:
-
Computer graphics use integrals to render realistic images and animations.
-
Machine learning algorithms often involve integrals to optimize models and make predictions.
-
Cryptography uses integrals to secure data and communications.
Future of Integral Analysis
The future of integral analysis looks promising, with ongoing research and new discoveries. Here are some exciting prospects:
- Advances in numerical integration techniques will enable more accurate and efficient solutions to complex problems.
Integral analysis remains a vital and dynamic field, continually shaping our understanding of the world.
Final Thoughts on Integral Analysis
Integral analysis isn't just for math enthusiasts. It plays a huge role in physics, engineering, economics, and even biology. Understanding integrals helps solve real-world problems, from calculating areas under curves to predicting population growth. It's a tool that bridges theoretical math with practical applications.
Grasping the basics of integral analysis can open doors to advanced studies and careers in various fields. Whether you're a student, a professional, or just curious, knowing these 31 facts can give you a solid foundation. Remember, math isn't just about numbers; it's about understanding the world around us.
So, keep exploring, stay curious, and don't be afraid to dive deeper into the fascinating world of integrals. The more you learn, the more you'll appreciate the beauty and utility of mathematics in everyday life. Happy learning!
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
