36 Facts About Yoneda

Yoneda Lemma: The Basics

The Yoneda Lemma is a fundamental result in category theory, a branch of mathematics. It provides deep insights into how objects in a category relate to each other through morphisms.

1. 01Named after Nobuo Yoneda, a Japanese mathematician who introduced it in 1954.
2. 02Central to category theory, often considered one of its cornerstones.
3. 03Relates objects and morphisms in a category, showing how an object can be understood by its relationships.
4. 04Uses functors, which are mappings between categories that preserve their structure.
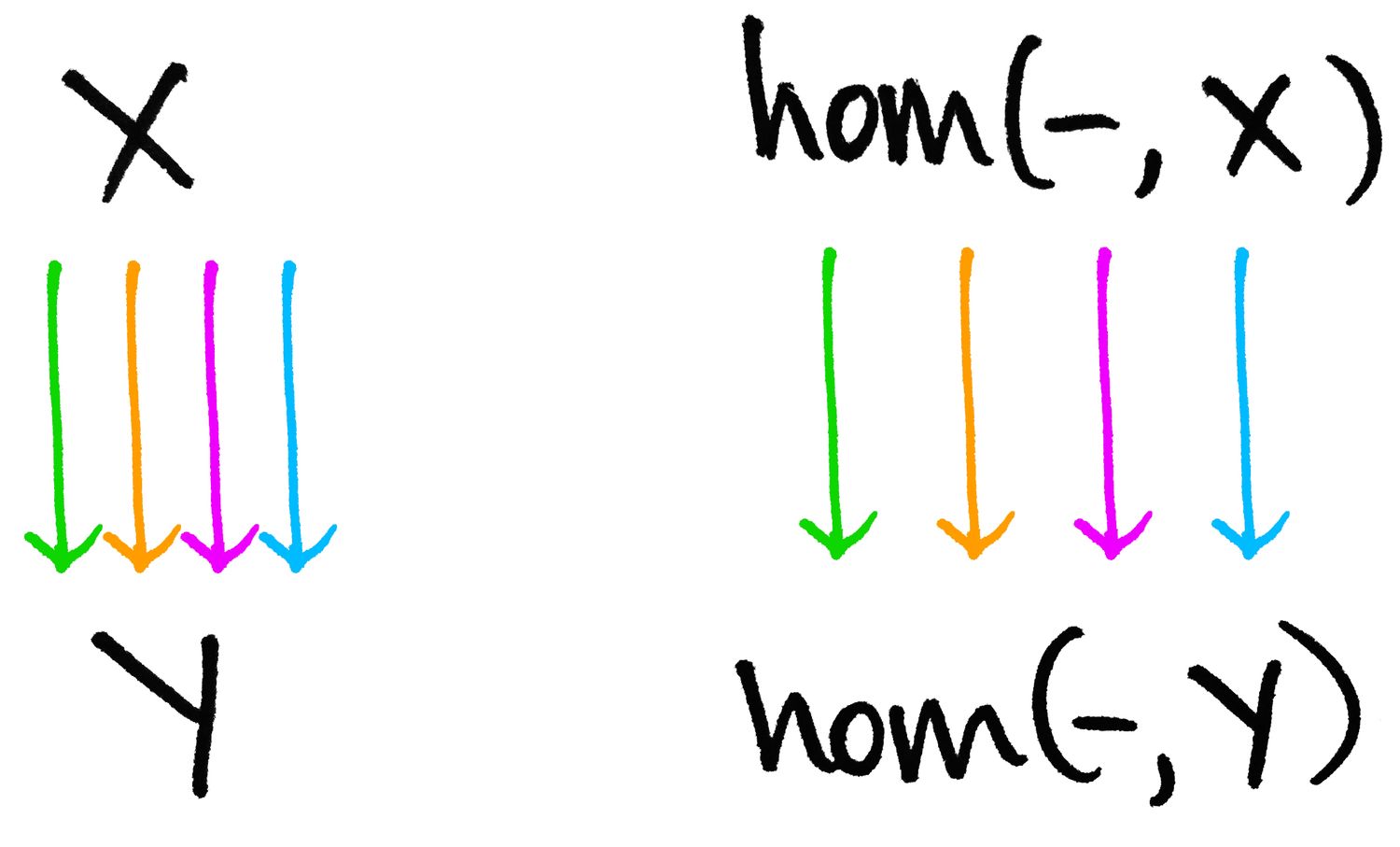
5. 05Helps in understanding representable functors, which are functors that can be represented by hom-sets.

Importance in Mathematics

The Yoneda Lemma is not just a theoretical concept; it has practical applications in various fields of mathematics.

6. 06Crucial for algebraic geometry, helping to understand schemes and sheaves.
7. 07Used in homological algebra, aiding in the study of chain complexes and derived categories.
8. 08Influences computer science, particularly in the theory of programming languages and type theory.
9. 09Aids in the study of topos theory, a branch of mathematics that generalizes set theory.
10. 10Helps in understanding natural transformations, which are mappings between functors.

Technical Aspects

Understanding the technical details of the Yoneda Lemma can be challenging but rewarding.

11. 11Involves hom-sets, which are sets of morphisms between objects in a category.
12. 12States that every functor is naturally isomorphic to a hom-functor.
13. 13Uses the concept of natural isomorphism, a way of showing that two functors are essentially the same.
14. 14Relies on the idea of representable functors, which can be represented by hom-sets.
15. 15Provides a way to embed a category into a functor category.

Applications in Other Fields

The Yoneda Lemma's influence extends beyond pure mathematics.

16. 16Used in theoretical computer science, particularly in the study of programming languages.
17. 17Helps in the development of functional programming languages, such as Haskell.
18. 18Influences type theory, a branch of mathematical logic.
19. 19Aids in the study of databases, particularly in understanding categorical database theory.
20. 20Used in physics, particularly in the study of quantum mechanics and quantum field theory.

Historical Context

Understanding the historical context of the Yoneda Lemma can provide deeper insights into its significance.

21. 21Introduced by Nobuo Yoneda in 1954, during a period of rapid development in category theory.
22. 22Part of the broader development of category theory, which began in the 1940s.
23. 23Influenced by earlier work in algebraic topology and homological algebra.
24. 24Helped to formalize the concept of functors, which were introduced in the 1940s.
25. 25Contributed to the development of modern algebraic geometry, particularly the theory of schemes.

Understanding Through Examples

Examples can help to illustrate the abstract concepts involved in the Yoneda Lemma.

26. 26Consider the category of sets, where objects are sets and morphisms are functions.
27. 27A functor from this category to itself can be represented by a hom-set.
28. 28The Yoneda Lemma shows that this functor is naturally isomorphic to the hom-functor.
29. 29In the category of groups, objects are groups and morphisms are group homomorphisms.
30. 30A functor from this category to the category of sets can be represented by a hom-set.

Further Reading

For those interested in learning more about the Yoneda Lemma, there are many resources available.

31. 31"Categories for the Working Mathematician" by Saunders Mac Lane, a foundational text in category theory.
32. 32"Sheaves in Geometry and Logic" by Saunders Mac Lane and Ieke Moerdijk, which explores the applications of category theory.
33. 33"Category Theory in Context" by Emily Riehl, a more accessible introduction to category theory.
34. 34Online resources, such as the nLab and Wikipedia, provide detailed explanations and examples.
35. 35Mathematical journals, where many papers on category theory and the Yoneda Lemma can be found.
36. 36University courses, which often include category theory as part of their curriculum.

Yoneda's Legacy in a Nutshell

Yoneda's contributions to mathematics, especially category theory, have left an indelible mark. His Yoneda Lemma is a cornerstone, influencing various fields like computer science and logic. This lemma simplifies complex concepts, making them accessible to both seasoned mathematicians and curious learners. Beyond his lemma, Yoneda's work on homological algebra and sheaf theory has paved the way for new discoveries and applications. His legacy isn't just in theorems and proofs; it's in the inspiration he provides to future generations of mathematicians. Yoneda's ability to see connections where others saw none has made him a giant in his field. As we continue to explore and expand upon his ideas, his influence will undoubtedly persist, guiding us through the intricate world of mathematics. Yoneda's work reminds us that even the most abstract concepts can have profound, real-world implications.