Cohomology might sound like a complex term, but it's a fascinating concept in mathematics. Cohomology is a method used to study the properties of geometric shapes and spaces. It helps mathematicians understand how different parts of a shape fit together. Imagine trying to figure out the hidden structure of a puzzle without seeing the picture on the box. That's what cohomology does for shapes and spaces. It reveals hidden connections and relationships that aren't immediately obvious. Whether you're a math enthusiast or just curious about how the world works, these 29 facts about cohomology will give you a glimpse into this intriguing field. Buckle up for a journey through the hidden layers of geometry!
What is Cohomology?
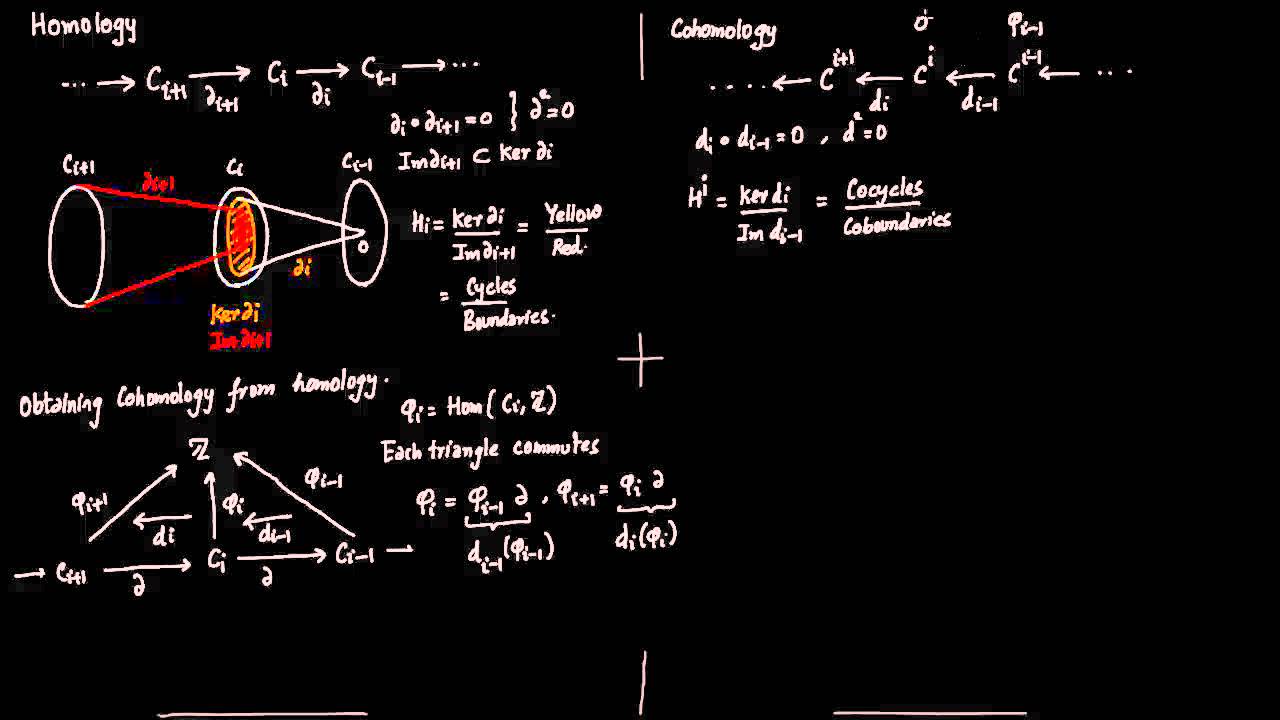
Cohomology is a branch of mathematics that studies the properties of spaces through algebraic tools. It provides a way to classify and measure the features of geometric objects. Here are some fascinating facts about cohomology.
-
Cohomology originated from topology, a field that explores the properties of space that are preserved under continuous transformations.
-
It was first introduced by mathematicians Élie Cartan and Henri Poincaré in the early 20th century.
-
Cohomology groups are algebraic structures that help in understanding the shape and structure of topological spaces.
-
These groups can be used to detect holes of different dimensions in a space, such as loops, voids, and higher-dimensional analogs.
-
The most common types of cohomology are de Rham cohomology, singular cohomology, and Čech cohomology.
Applications of Cohomology
Cohomology isn't just theoretical; it has practical applications in various fields. Let's explore some of these applications.
-
In physics, cohomology plays a crucial role in the study of gauge theory and string theory.
-
It helps in the classification of vector bundles, which are essential in understanding fiber spaces in mathematics and physics.
-
Cohomology is used in algebraic geometry to study the properties of algebraic varieties.
-
It aids in the analysis of differential equations by providing tools to solve complex systems.
-
In computer science, cohomology concepts are applied in data analysis and machine learning to understand the shape of data.
Key Concepts in Cohomology
Understanding cohomology requires familiarity with some key concepts. Here are a few important ones.
-
A cochain is a sequence of functions that assign values to the elements of a space.
-
The coboundary operator is a function that maps cochains to other cochains, helping to define cohomology groups.
-
A cocycle is a cochain that maps to zero under the coboundary operator, representing a closed form.
-
A coboundary is a cochain that is the image of another cochain under the coboundary operator, representing an exact form.
-
The cohomology group is the quotient of the group of cocycles by the group of coboundaries, providing a measure of the "holes" in a space.
Famous Theorems in Cohomology
Several important theorems form the backbone of cohomology theory. Here are some notable ones.
-
The de Rham theorem states that de Rham cohomology is isomorphic to singular cohomology with real coefficients.
-
The Mayer-Vietoris sequence is a long exact sequence used to compute the cohomology of a space from the cohomology of its parts.
-
The Künneth theorem provides a way to compute the cohomology of a product space from the cohomology of its factors.
-
The Hodge theorem relates the cohomology of a smooth manifold to the space of harmonic forms on the manifold.
-
Poincaré duality states that for a closed, oriented manifold, the cohomology groups in complementary dimensions are isomorphic.
Advanced Topics in Cohomology
For those delving deeper into cohomology, several advanced topics offer further insights. Here are a few.
-
Sheaf cohomology generalizes the concept of cohomology to sheaves, which are tools for systematically tracking locally defined data.
-
Spectral sequences provide a method for computing cohomology groups by filtering complex structures.
-
The Atiyah-Singer index theorem links the analytical properties of differential operators to the topological properties of manifolds.
-
Equivariant cohomology studies spaces with group actions, providing tools to understand symmetries in spaces.
-
Floer cohomology is used in symplectic geometry and low-dimensional topology to study the properties of Hamiltonian systems.
Fun Facts about Cohomology
Cohomology isn't just for mathematicians; it has some fun and quirky aspects too. Here are a few.
-
The term "cohomology" comes from the Greek words "co-" meaning "together" and "homology" meaning "similarity."
-
Cohomology can be visualized using diagrams and graphs, making it accessible to those with a visual learning style.
-
Some artists use concepts from cohomology to create intricate and abstract artworks.
-
There are online communities and forums where enthusiasts discuss and share their love for cohomology, making it a collaborative and engaging field.
Final Thoughts on Cohomology
Cohomology isn't just a fancy term in mathematics. It's a powerful tool that helps us understand shapes, spaces, and structures in ways we couldn't before. From its roots in algebraic topology to its applications in modern physics, cohomology bridges gaps between different areas of study. It provides insights into the properties of geometric objects and helps solve complex problems. Whether you're a student, a researcher, or just curious, knowing a bit about cohomology can open up new perspectives. So next time you hear the term, you'll know it's not just math jargon—it's a key to unlocking deeper understanding in various fields. Keep exploring, keep questioning, and who knows? You might find cohomology popping up in places you never expected.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.
