29 Facts About Algebraic Functions

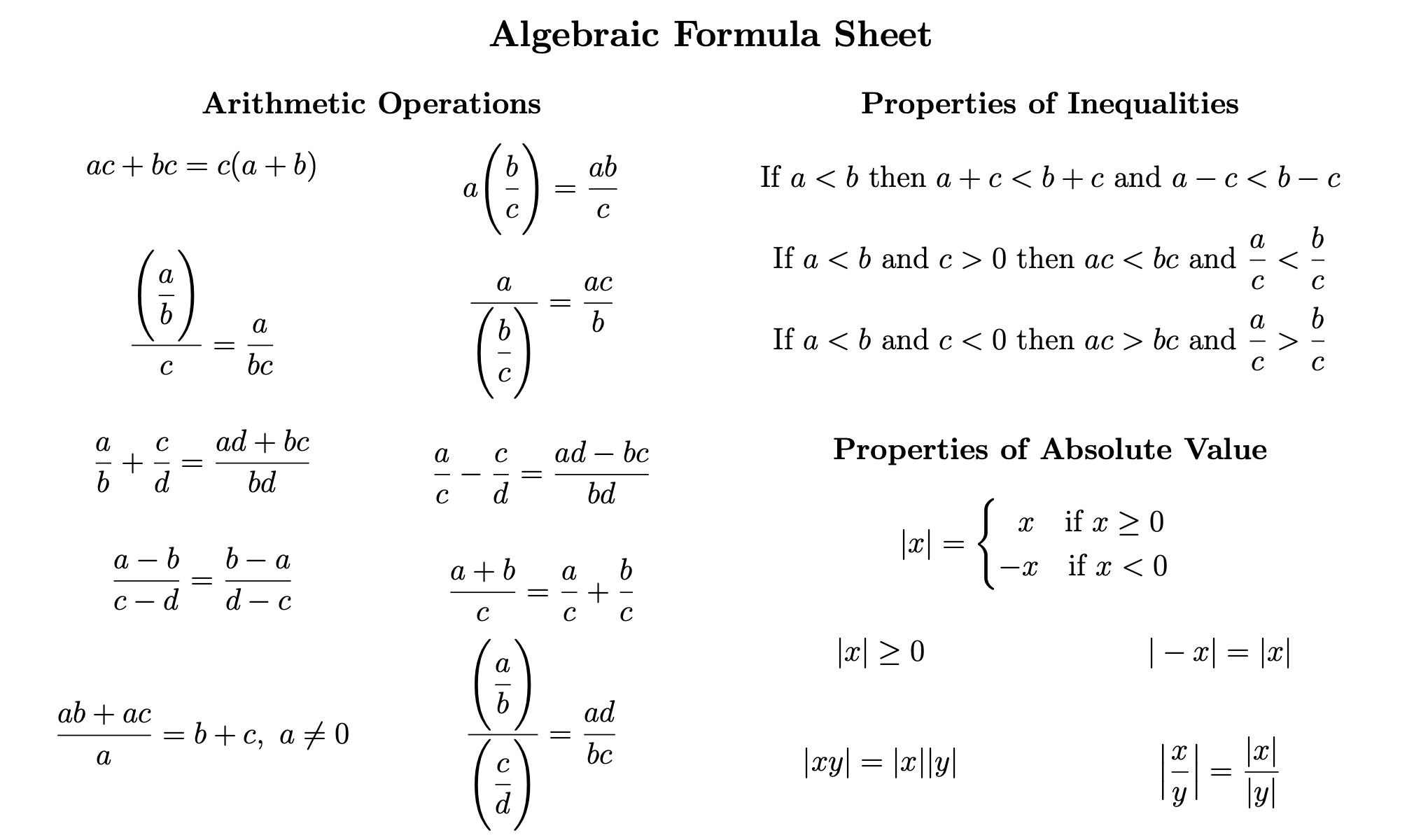
What Are Algebraic Functions?

Algebraic functions are mathematical expressions involving variables and constants. They can be simple or complex, but they all follow specific rules. Here are some fascinating facts about algebraic functions.

1. Algebraic functions can be polynomials, rational functions, or radicals.
2. Polynomials are algebraic functions with terms that are non-negative integer powers of variables.
3. Rational functions are ratios of two polynomials.
4. Radical functions involve roots, such as square roots or cube roots.

History of Algebraic Functions

The history of algebraic functions is rich and spans many centuries. Let's explore some key historical facts.

5. The word "algebra" comes from the Arabic word "al-jabr," meaning "reunion of broken parts."
6. Persian mathematician Al-Khwarizmi wrote a book in the 9th century that laid the foundation for algebra.
7. European mathematicians like René Descartes and Pierre de Fermat further developed algebraic functions in the 17th century.
8. The quadratic formula, used to solve quadratic equations, was known to ancient Babylonians around 2000 BC.

Types of Algebraic Functions

There are various types of algebraic functions, each with unique properties. Here are some interesting facts about them.

9. Linear functions have the form ( f(x) = mx + b ), where ( m ) and ( b ) are constants.
10. Quadratic functions have the form ( f(x) = ax^2 + bx + c ), where ( a ), ( b ), and ( c ) are constants.
11. Cubic functions have the form ( f(x) = ax^3 + bx^2 + cx + d ).
12. Quartic functions have the form ( f(x) = ax^4 + bx^3 + cx^2 + dx + e ).

Applications of Algebraic Functions

Algebraic functions are not just theoretical; they have practical applications in various fields. Here are some examples.

13. Engineers use algebraic functions to design and analyze structures.
14. Economists use them to model supply and demand curves.
15. Biologists use algebraic functions to describe population growth.
16. Computer scientists use them in algorithms and data structures.

Solving Algebraic Functions

Solving algebraic functions involves finding the values of variables that satisfy the equation. Here are some methods and facts.

17. Factoring is a common method for solving polynomial equations.
18. The quadratic formula ( x = frac{-b pm sqrt{b^2 – 4ac}}{2a} ) solves quadratic equations.
19. Synthetic division is a shortcut method for dividing polynomials.
20. The Rational Root Theorem helps find possible rational roots of polynomial equations.

Famous Algebraic Equations

Some algebraic equations have become famous due to their importance and complexity. Here are a few notable ones.

21. The Pythagorean theorem ( a^2 + b^2 = c^2 ) relates the sides of a right triangle.
22. Euler's identity ( e^{ipi} + 1 = 0 ) is considered one of the most beautiful equations in mathematics.
23. The cubic equation ( ax^3 + bx^2 + cx + d = 0 ) was solved by Italian mathematicians in the 16th century.
24. Fermat's Last Theorem states that ( x^n + y^n = z^n ) has no integer solutions for ( n > 2 ).

Interesting Properties of Algebraic Functions

Algebraic functions have some intriguing properties that make them unique. Here are a few.

25. The degree of a polynomial function is the highest power of the variable in the polynomial.
26. The Fundamental Theorem of Algebra states that every non-constant polynomial equation has at least one complex root.
27. Symmetry in algebraic functions can simplify solving them. For example, even functions are symmetric about the y-axis.
28. The roots of a polynomial function are the values of the variable that make the function equal to zero.

Challenges in Algebraic Functions

Working with algebraic functions can be challenging, but these challenges have led to significant mathematical advancements.

29. Finding the roots of higher-degree polynomials (degree 5 or higher) cannot always be done using radicals, as proven by the Abel-Ruffini theorem.

Algebraic Functions: A Quick Recap

Algebraic functions play a huge role in math and everyday life. From simple linear equations to complex polynomials, they help us solve problems and understand patterns. Knowing how to work with these functions can make tasks like budgeting, planning, and even coding easier. They’re not just for math class; they’re tools we use all the time without even realizing it. Whether you’re graphing a line or figuring out how much paint you need for a room, algebraic functions are there to help. So next time you face a math problem, remember these functions are your friends. They simplify complex ideas and make problem-solving a breeze. Keep practicing, and you’ll find that algebraic functions become second nature. Happy calculating!