28 Facts About Equivalence Relations

What is an Equivalence Relation?

Equivalence relations are a fundamental concept in mathematics. They help group elements that share a common property. Understanding these relations can simplify complex problems.

1. 01Definition: An equivalence relation on a set is a relation that is reflexive, symmetric, and transitive.
2. 02Reflexive Property: Every element is related to itself. For example, in a set of numbers, each number is equal to itself.
3. 03Symmetric Property: If one element is related to another, then the second element is related to the first. If a = b, then b = a.
4. 04Transitive Property: If one element is related to a second, and the second is related to a third, then the first is related to the third. If a = b and b = c, then a = c.

Examples of Equivalence Relations

Examples make abstract concepts easier to grasp. Here are some common examples of equivalence relations.

5. 05Equality: The most straightforward example. If two numbers are equal, they are related.
6. 06Congruence Modulo n: In number theory, two integers are congruent modulo n if their difference is divisible by n. For instance, 7 and 12 are congruent modulo 5.
7. 07Parallel Lines: In geometry, two lines are parallel if they never intersect. This is an equivalence relation among lines.
8. 08Similarity of Triangles: Triangles are similar if their corresponding angles are equal and their sides are proportional.

Applications of Equivalence Relations

Equivalence relations are not just theoretical; they have practical applications in various fields.

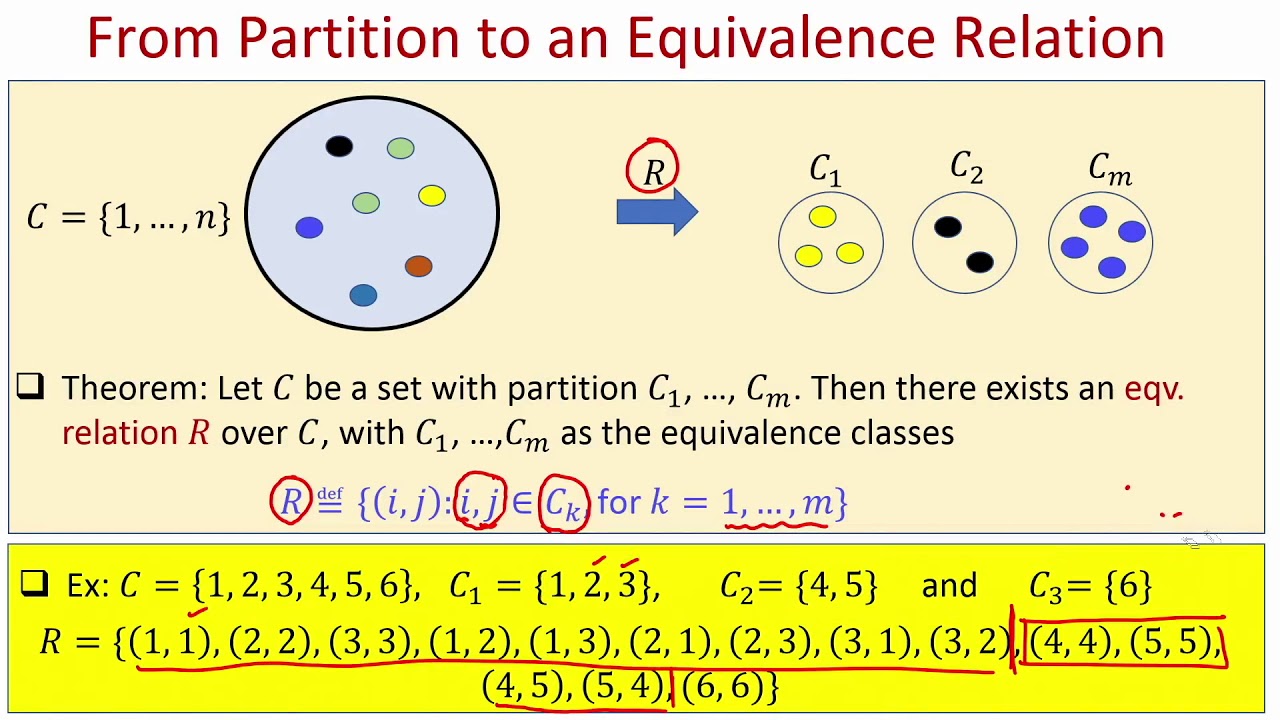
9. 09Partitioning Sets: Equivalence relations can partition a set into disjoint subsets called equivalence classes.
10. 10Cryptography: In cryptography, equivalence relations help in creating secure communication channels.
11. 11Database Management: Equivalence relations are used to manage and query relational databases efficiently.
12. 12Coding Theory: They help in error detection and correction in coding theory.

Equivalence Classes

Equivalence classes are subsets formed by grouping elements related by an equivalence relation.

13. 13Definition: An equivalence class is a subset containing elements that are all equivalent to each other.
14. 14Notation: If ~ is an equivalence relation on a set, the equivalence class of an element a is denoted by [a].
15. 15Unique Partition: Every element of the set belongs to exactly one equivalence class.
16. 16Example: In the set of integers, the equivalence class of 0 under congruence modulo 3 is {…, -6, -3, 0, 3, 6, …}.

Properties of Equivalence Classes

Equivalence classes have unique properties that make them useful in various mathematical contexts.

17. 17Disjoint: Equivalence classes are disjoint; no element can belong to two different classes.
18. 18Union: The union of all equivalence classes is the entire set.
19. 19Cardinality: If the set is finite, the number of equivalence classes is also finite.
20. 20Representative: Each equivalence class can be represented by any of its elements.

Real-World Examples

Equivalence relations are not just confined to textbooks; they appear in everyday life.

21. 21Social Networks: People are grouped based on mutual friendships, forming equivalence classes.
22. 22Language: Words with the same meaning form equivalence classes in a thesaurus.
23. 23Legal Systems: Laws that apply equally to all citizens create equivalence classes of individuals.
24. 24Education: Students grouped by grade level form equivalence classes.

Advanced Concepts

For those who want to dive deeper, here are some advanced concepts related to equivalence relations.

25. 25Quotient Sets: Formed by partitioning a set using an equivalence relation.
26. 26Homogeneous Spaces: Spaces that look the same at every point, often described using equivalence relations.
27. 27Factor Groups: In group theory, equivalence relations help define factor groups.
28. 28Topological Spaces: Equivalence relations are used to define quotient topologies in topological spaces.

Final Thoughts on Equivalence Relations

Equivalence relations might seem tricky at first, but they’re super useful in math and computer science. They help us group things that are similar in a meaningful way. Remember, an equivalence relation needs to be reflexive, symmetric, and transitive. This means each element relates to itself, if one element relates to another, the second relates back, and if one relates to a second and the second to a third, the first relates to the third. These properties make it easier to understand complex structures by breaking them into simpler, related parts. Whether you’re working with numbers, shapes, or even data sets, understanding equivalence relations can simplify your work. Keep these facts in mind, and you’ll find them popping up in all sorts of interesting places!